How to Solve Mixture Problems in Simple Steps 1

Submitted by Atanu Chaudhuri on Sun, 03/04/2016 - 22:24

how to solve mixture problems in simple steps 1

How to Solve Mixture Problems by Ratio Portions concepts in Simple Quick Steps

Learn how to solve mixture problems with two or more liquids mixed in given ratios. Basic ratio mixture concepts are explained by solving selected problems.

The mixture problem concepts and techniques covered here form the foundation in solving various types of mixture problems

The concept article is divided in sections,

Basic Mixture and alligation Concepts - Types of mixing two liquids or two solids in alloys or even mixing two mixtures.
Basic Concepts on Ratios and Ratio techniques.
Basic Mixture Problem Solving Techniques.
How to solve Mixture problems on mixing two (and three) mixtures.

For your convenience we will start this session with Concepts part and then only will start solving specific problems for showing quick and elegant ways to reach the solutions in a few steps.

Mixture and Alligation concepts

By definition, the term Mixture means mixture of two components (usually liquids, occasionally solids) that can be mixed together.

By itself the concept or mechanism of mixing is not enough to form various kinds of challenging mixture problems for all types of competitive exams.

The term Alligation introduces the concept of mixing two components in Ratios. In other words, it provides a clear way of mixing the components with no ambiguity.

Combining the two concepts together,

Mixture and Alligation problems involve MIXING TWO OR MORE COMPONENTS IN RATIOS OF COMPONENTS.

Mixture problems may be quite difficult to solve.

Nature of the operation of mixing

Homogeneity of mixtures is the most basic starting concept,

Mixture and alligation problems involves ratios of amount of two or more liquids that can be mixed homogeneously.

One of the basic properties of mixing is,

Once you mix two liquids (or solids) you won't be able to separate out the two components from the mixture. That's inherent in mixing homogeneously.

For example, a milkman might sell diluted milk, but when one of his important customers objects, the maximum he can do is to mix more milk in the diluted milk to make it more concentrated milk, but he won't be able to take out the water from the initial diluted milk that he had sold him.

Repeated mixing of one or the other liquid in a container increases complexity, because in a mixing problem you might know the ratio of amounts of two liquids mixed, but you may not know the actual amounts mixed.

Mixture problem type 1: Substitution of some amount of mixture with a component liquid (or solid)

A frequent operation in mixing problems is to take out, say 5 liters, of mixture from the diluted milk and then replace it with water. By this operation, the starting volume remains unchanged but the ratio of amounts of milk and water in the mixture changes.

The core concept in dealing with such problems is,

When you take out the 5 liters of diluted milk, you take out milk and water both in certain proportions. While replacing the 5 liters though you put in only water. The milk portion taken out is wholly lost, but the 5 liters of water that you put in won't increase the amount of water by the whole of 5 liters as, while taking out 5 liters of diluted milk you took out a portion of water also that was lost.

Repeated substitution creates complexity and more difficulties.

Mixture problem type 2: Mixing of two mixtures

Instead of carrying out the mixing operations on one mixture, two mixtures with two different proportions of same liquids in them may also be mixed, that too in different proportions for increasing the complexity level.

We recommend,

Be clear about the basic and rich concepts and use the concepts in solving problems rather than use specific procedures 9or formulas) for specific types of problems.

Basic concepts on ratios and Ratio techniques

You need to have clear understanding about ratios and what does a ratio really represent, because ratios form the base concept layer where you may encounter difficulties in many mixing and other types of problems.

Definition of a ratio expression

A ratio represents comparison of one common characteristic in same units of two or more entities and is expressed as a series of numbers or variables separated by the symbol of colon ':'.

This is an abstract but exhaustive definition of ratios. This abstraction coupled with exhaustivity will help you to deal with different types of situations in a specific area, say of ratios.

A simple example of use of ratios follows.

Problem example: If ratio of ages of son and father is 1 : 3 and 12 years back 1 : 7, what are their ages?

Ratio concepts and techniques

Fractional form: A ratio is conventionally shown in minimized fraction form. In fact an important charateristic of ratios is,

A ratio can always be expressed as a fraction and conventionally is expressed in minimized fraction form (with all common factors cancelled out).

HCF is canceled out between two quantities in ratio: All common factors cancelled out between the two terms of a ratio is the HCF of the actual values of the two original quantities involved in the ratio.
Re-introduction of HCF technique: For convenience, you may always re-introduce this canceled out HCF multiplying each of the two ratio terms. In our example, the present ratio of ages of 1 : 3 can very well be expressed as $x : 3x$, with $x$ as the HCF of the two ages of son and father. With this re-introduction of a single variable $x$ in the proceedings, we may now represent the problem state 12 years back as, $(x - 12) : (3x - 12) = 1 : 7$, Or, $7x - 84 = 3x - 12$, or, $x = 18$, and present ages of son and the father respectively, 18 years, and 54 years.
You won't introduce two variables of two unknowns in ratio sums, just use one variable to get the two ratio terms in the single variable expressions. This is the key technique in ratio sums.
Expressing amount of each component in terms of portion of total amount in the mixture: This re-introduction of the canceled out HCF as a single variable $x$ enables us to express the total age as, $x + 3x = 4x$, and more importantly, each ratio term as a portion of the total - son's age single portion of total age and father's age automatically the rest three portions of total age. This is a very important way of looking at changing ratios especially in mixing sums where total volume is fixed. If we express each liquid component as a portion of the fixed total volume, we don't have to deal with two varying quantities, our thinking load clearly diminishes significantly.

Basic Mixture Problem Solving Techniques

Expressing amount of liquids as portions of total mixed amount

Two mixable liquids (oil and water can't easily be mixed) milk and water are mixed thus forming a diluted milk with milk to water ratio, say 4 : 1.

Many times this statement may be expressed in more usable forms of,

A total 5 portions of diluted milk contains 4 portions of milk and 1 portion of water.
Milk in diluted mixture is four-fifths of total volume and water one-fifth of total volume of mixture.
In 5 portions of diluted milk, 4 portions of total mixture is milk and 1 portion of total mixture is water.

Operation of replacement of certain amount of mixture with only one liquid

Most frequently a fixed amount, say 3 liters, of a mixture, say our diluted milk of milk to water ratio 4 : 1, is taken out of the mixture and replaced by the same amount of a single liquid (it could be any of the two liquids or may even be another mixture), say by water.

We can perceive that by this operation, the diluted milk get further diluted, but what exactly does happen in the replacement operation?

Result of first operation of taking out 3 liters of mixture

The 3 liters of diluted milk that we take out contained both milk and water in the ratio of 4 : 1. So by our third statement expressing amount of each liquid as a portion of total mixture volume, we may be clear that by taking out this 3 liters of diluted milk,

$3\times{\displaystyle\frac{4}{5}}=\displaystyle\frac{12}{5}$ liters of milk and $3\times{\displaystyle\frac{1}{5}}=\displaystyle\frac{3}{5}$ liters of water are taken out.

This is dealing with exact amounts of milk and water taken out in the first part of replacement operation.

Result of second operation of putting in 3 liters of water

The first conclusion is obvious: by adding 3 liters of water, total volume of mixture returns to its earlier value. It effectively remains unchanged. But what happens with portions of milk and water and the final result of two part operation? Just note that volume of mixture is not known.

It is clear that, milk amount is reduced by exactly $\displaystyle\frac{12}{5}$ liters but water amount has not increased by 3 liters, in fact it is increased by, $3 - \displaystyle\frac{3}{5} = 3\left(1 - \displaystyle\frac{1}{5}\right)$ litres.

There are more to alligation concepts. Later, we will elaborate on the concepts as required.

Let us now solve a few mixing liquid problems in as few steps as possible using the basic ratio and mixing liquids concepts and requisite problem solving strategies.

How to solve Mixture Problem 1: Two mixtures are mixed together

In two containers of equal capacity, milk to water ratio is 3 : 1 in the first and 5 : 2 in the second. If these two are mixed together, the ratio of milk to water in the new mixture becomes,

41 : 28
41 : 15
28 : 41
15 : 41

Solution to mixture problem 1 using conceptual reasoning:

First stage Problem analysis:

Total volume in the first mixture in terms of portions is 4 portions and in the second 7 portions. We can then assume the total volume to be LCM of the two, that is, 28 portions without affecting the individual ratios.

This is application of our powerful Base equalization technique. We have equalized the total volumes of two mixtures in terms of portions to the same LCM value without affecting the individual ratios. The big advantage in taking this step is in equalizing the amounts of a portion in each mixture as well, as the actual volumes are same.

By using mathematical reasoning, we have avoided the use of a variable $x$ here, representing the total volume of each container. We could do that because in both the containers the total volume is same and when mixed together the new volume will also be just the double of the old volume.

Adding the milk and water portions individually we get,

Milk portions = $\frac{28}{4}\times{3} + \frac{28}{7}\times{5} = 21 + 20 = 41$ portions out of total 56 portions, and

Water portions = $\frac{28}{4}\times{1} + \frac{28}{7}\times{2} = 7 + 8 = 15$ portions out of total 56 portions.

We have used the enhanced basic concept of mixing, that is, expressing amount of one liquid as portion of total volume of mixture.

So the milk to water ratio in new mixture is 41 : 15.

Answer: Option b: 41 : 15.

Key concepts used:

By the use of enhanced basic concept of mixing, expressing first the individual liquid amounts as portions of total mixture amount.
By using mathematical reasoning and base equalization technique, assumption of the total amount as a feasible number of LCM of 4 and 7, that is, 28 portions in the beginning.
As the amount of a portion is equalized in the two mixtures with this LCM total volume, we can now add up the portions of milk and water in two mixtures individually to get the final amount of portions of each.
A simple ratio of the two gives us the answer as each of 41 and 15 are in terms of equal portions of total volume.
Here we have used the concept of ratio also without mentioning it. Whenever the final answer is wanted in terms of a ratio, we can assume a convenient value of the quantity in terms of which the individual ratio terms are expressed. The total quantity cancels out in forming the ratio. This important property of ratio may be used in reaching elegant solutions in many other problem areas, especially commercial maths, such as, profit and loss.

Conventional solution using unknown variable $x$

Let the capacity of each container be $x$ units. Thus, in the first container,

Milk is $=\frac{3}{4}x$ and water $=\frac{1}{4}x$.

Similarly in the second container,

Milk is $=\frac{5}{7}x$ and water $=\frac{2}{7}x$.

Total milk $=\frac{41}{28}x$ and,

Total water $=\frac{15}{28}x$.

Thus final milk to water ratio is, 41 : 15.

Remarks: This is a deductive process suitable for formal deductive and descriptive answering environment. As such nothing is wrong in this solution except that it mechanically follows procedures to the solution and thereby takes more time.

Comparing two solutions you can see now that the variable $x$ cancels out. Knowing this beforehand, why should you get involved in this deduction! Go straight to the solution wholly in your mind without putting your pen to the paper. This situation holds in case of MCQ based tests.

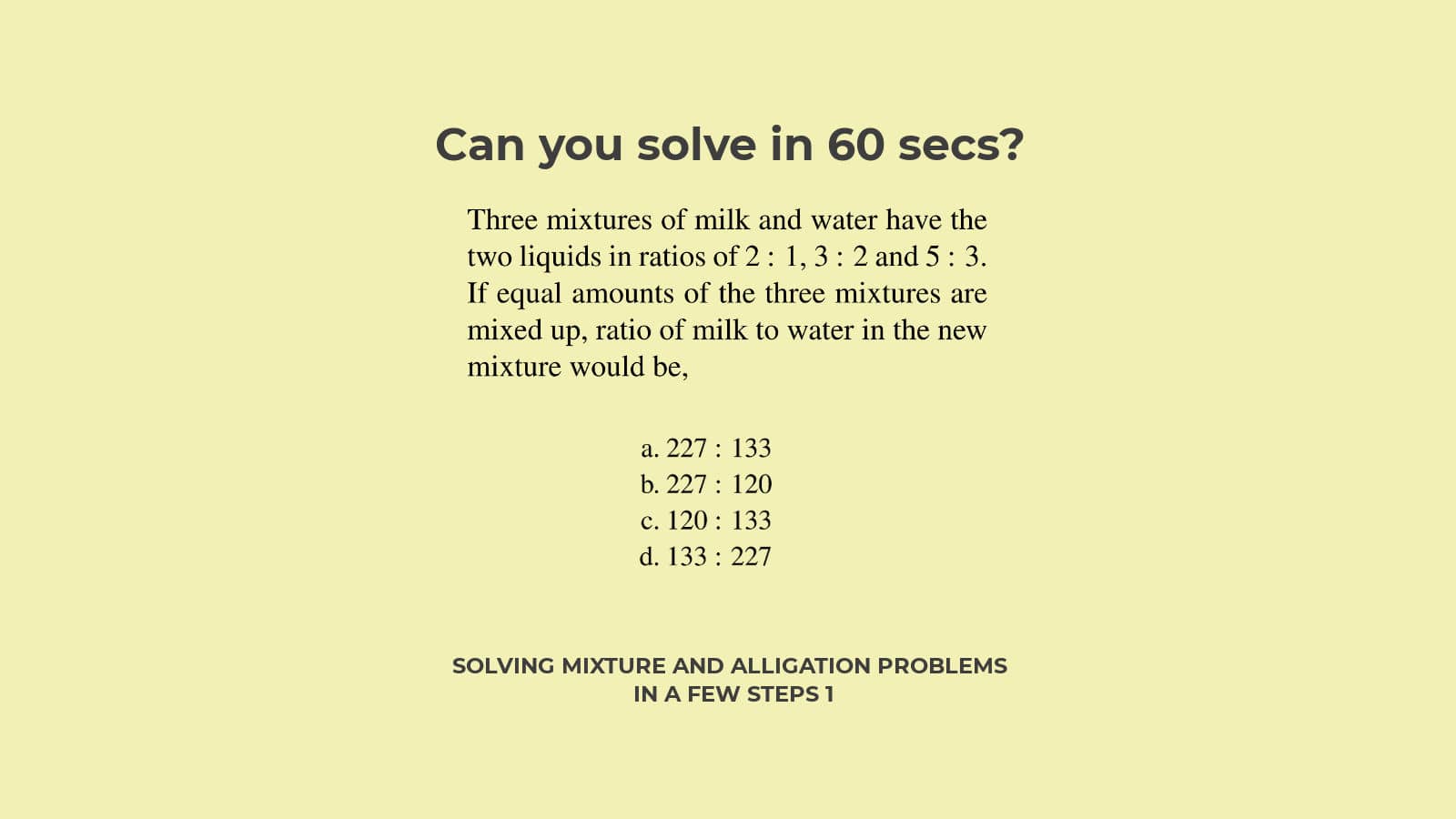
How to solve Mixture Problem 2: Equal amounts of three mixtures mixed together

Three mixtures of milk and water have the percentages of the two in ratios of 2 : 1, 3 : 2 and 5 : 3. If equal amounts of the three mixtures are mixed up the ratio of milk to water in the new mixture would be,

227 : 133
227 : 120
120 : 133
133 : 227

Solution to mixture problem 2 by conceptual reasoning:

Total volume in the first mixture is $2+1=3$ portions, in the second $3+2=5$ portions and in the third $5+3=8$ portions.

We can then assume the volume to be equalized as LCM of 3, 5 and 8, that is, 120 portions.

At this LCM of total portions volume, a portion of each of the mixtures will be of same value.

Stop a moment to absorb this fundamental concept.

We need to transform each of the ratios so that total volume of each becomes 120 portions.

To do this you need to multiply the first ratio by $\frac{120}{3}=40$, the second by $\frac{120}{5}=24$ and the third by $\frac{120}{8}=15$ (these are results of dividing 120 by each of the initial total volume in portions 3, 5 and 8 respectively).

This is an application of our powerful Base equalization technique. We have equalized the total volume portions to the same LCM value without affecting the individual ratios in any way.

Thus we have avoided the use of a variable $x$ here.

Adding the milk and water portions individually we get,

Milk portions = $\frac{120}{3}\times{2} + \frac{120}{5}\times{3} + \frac{120}{8}\times{5} $

$= 120(\frac{2}{3} + \frac{3}{5} + \frac{5}{8}) = 120\frac{80 + 72 + 75}{120} = 227$ portions out of total, and

Water portions = $\frac{120}{3}\times{1} + \frac{120}{5}\times{2} + \frac{120}{8}\times{3} $

$= 120(\frac{1}{3} + \frac{2}{5} + \frac{3}{8}) = 120\frac{40 + 48 + 45}{120} = 133$ portions out of total.

Answer: Option a: 227 : 133.

Key concepts used:

Like the previous problem we have assumed total volume as the LCM of the totals of individual mixtures (in terms of portions).
Now while summing up the three components of one liquid we have not calculated the actual values. Instead we have taken out the common factor of 120 out of the brackets and just calculated the sum of fractions.
The ratio of numerators would give us the answer.

Summary of Steps taken:

Individual amounts of liquids are expressed as portions of total volume in each mixture.
Total volume of the mixtures are equalized to LCM of total portions of all three mixtures by suitably transforming the ratio terms of the mixtures. At this total portion volume that are equal, amount of 1 portion in all three mixtures becomes equal.
These equal portion amounts of three mixtures are added up (or mixed) to 360 portions.
Converted ratio term values of ONE component WATER in each mixture are added up to get 133 portions of WATER in the final mixture.
That decided rest $360-133=227$ portions to be MILK.
No variable is used in this conceptual solution.

If concepts are clear, the method gives you the solution in a few tens of seconds.