Circle questions for SSC CGL Solved geometry question set 96
Solve circle questions for SSC CGL and other geometry questions 10 in 15 minutes in set 96. Verify correctness from answers. Learn to solve from solutions.
Many of these 10 problems are difficult.
The solved question set contains,
- Circle questions for SSC CGL and other geometry questions to be answered in 15 minutes (10 chosen questions)
- Answers to the questions, and,
- Detailed conceptual solutions to the questions.
For best results, take the test first and then learn from solutions.
Circle questions for SSC CGL and other geometry questions set 96 - answering time 15 mins
Q1. The distance between the centres of two circles of radii $r_1$ and $r_2$ is $d$. They will touch each other internally if,
- $d=r_1 \text{ or } r_2$
- $d=r_1+r_2$
- $d=\sqrt{r_1r_2}$
- $d=r_1-r_2$
Q2. An arc of $30^0$ in one circle is double an arc in a second circle, the radius of which is three times the radius of the first. Then the angle subtended by the arc of the second circle at its centre is,
- $4^0$
- $6^0$
- $5^0$
- $3^0$
Q3. P is a point outside the circle at a distance of 6.5 cm from centre O of the circle. PR is a secant such that it intersects the circle at Q and R. If $PQ=4.5$ cm, and $QR=3.5$ cm, then what is the radius (in cm) of the circle?
- $2$
- $2.5$
- $3$
- $1.5$
Q4. In the given figure, O is the incentre of $\triangle ABC$. If $\displaystyle\frac{AO}{OE}=\frac{5}{4}$ and $\displaystyle\frac{CO}{OD}=\frac{3}{2}$, then what is the value of $\displaystyle\frac{BO}{OF}$?

- $\displaystyle\frac{38}{7}$
- $\displaystyle\frac{19}{7}$
- $\displaystyle\frac{19}{14}$
- $\displaystyle\frac{38}{17}$
Q5. The lengths of the sides of a triangle are $a$, $b$, and $c$ respectively. If $a^2+b^2+c^2=ab+bc+ca$, then the triangle is,
- right-angled
- scalene
- equilateral
- isosceles
Q6. In a $\triangle ABC$, the side BC is extended up to D, such that $CD=AC$. If $\angle BAC=109^0$ and $\angle ACB=72^0$, then the value of $\angle ABC$ is,
- $35^0$
- $45^0$
- $40^0$
- $60^0$
Q7. In the given figure, area of isosceles $\triangle ABE$ is 72 sq cm, $BE=AB$ and $AB=2AD$. If $AE||DC$, then what is the area (in sq. cm) of the trapezium ABCD?

- $144$
- $124$
- $108$
- $136$
Q8. $\triangle XYZ$ is similar to $\triangle PQR$. If ratio of perimeter of $\triangle XYZ$ and perimeter of $\triangle PQR$ is $16:9$ and $PQ=3.6$ cm, then what is the length (in cm) of $XY$?
- $3.2$
- $4.8$
- $8.6$
- $6.4$
Q9. PQ is a diameter of a circle with centre O. RS is a chord parallel to PQ that subtends an angle of $40^0$ at the centre of the circle. If PR and QS are produced to meet at T, then what will be the measure (in degrees) of $\angle PTQ$?
- $55$
- $90$
- $60$
- $70$
Q10. A circle is inscribed in a $\triangle ABC$. It touches the sides AB, BC and AC at points R, P and Q respectively. If $AQ=4.5$ cm, $PC=5.5$ cm and $BR=6$ cm, then perimeter of the triangle is,
- $26.5$ cm
- $30.5$ cm
- $32$ cm
- $28$ cm
Answers to the circle questions for SSC CGL and other geometry questions set 96
Q1. Answer: Option d: $d=r_1-r_2$.
Q2. Answer: Option c: $5^0$.
Q3. Answer: Option b: $2.5$.
Q4. Answer: Option a: $\displaystyle\frac{38}{7}$.
Q5. Answer: Option c: equilateral.
Q6. Answer: Option a: $35^0$.
Q7. Answer: Option a: $144$.
Q8. Answer: Option d: $6.4$.
Q9. Answer: Option d: $70$.
Q10. Answer: Option c: $32$ cm.
Solutions to the circle questions for SSC CGL and other geometry question set 96 - answering time was 15 mins
Q1. The distance between the centres of two circles of radii $r_1$ and $r_2$ is $d$. They will touch each other internally if,
- $d=r_1 \text{ or } r_2$
- $d=r_1+r_2$
- $d=\sqrt{r_1r_2}$
- $d=r_1-r_2$
Solution 1: Quick solution by visualization of the two internally touching circles
For easy visualization, let us draw the figure of the two internally touching circles as below.
Note: When you draw by hand, the figure need not be to the scale, or accurate with perfect circles. You need to capture just the fundamental relation between the radii of the two circles.
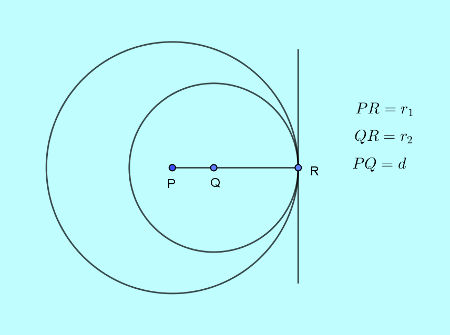
The radius of the larger circle is PR from its centre P to the common touching point R. The radius of the smaller circle QR from its centre Q to the same touching point R overlaps the radius of the larger circle PR and falls short by PQ.
This is the distance between the two centres and equals the difference of the two radii,
$d=r_1-r_2$.
Answer: Option d: $d=r_1-r_2$.
Key concepts used: Visualization -- Two internally touching circles concept -- Solving in mind.
Q2. An arc of $30^0$ in one circle is double an arc in a second circle, the radius of which is three times the radius of the first. Then the angle subtended by the arc of the second circle at its centre is,
- $4^0$
- $6^0$
- $5^0$
- $3^0$
Solution 2: Quick solution by arc angle subtended at the centre to perimeter relation of a circle and unitary method
The following figure shows the problem situation
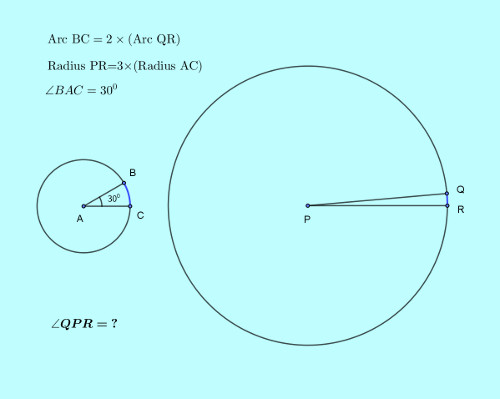
Let $r_1$ and $r_2$ be the radii and $A_1$ and $A_2$ be the arcs of the smaller and the larger circles respectively.
Also let the desired angle subtended by the arc of the larger circle be $\theta$.
In a circle, a basic property of an arc is,
The length of an arc is proportional to the angle subtended by it at the centre.
Because of this proportionality, unitary method can be applied to find the angle subtended by an arc or the length of an arc from the reference figure of the whole periphery,
The whole circle periphery of length $2\pi r_1$ subtends an angle of $360^0$ at the centre.
So in the smaller circle, as angle subtended $30^0$ is $\displaystyle\frac{30^0}{360^0}=\frac{1}{12}$th of the angle subtended by the whole periphery of $2\pi r_1$, its length would be,
$A_1=\displaystyle\frac{2\pi r_1}{12}=\displaystyle\frac{\pi r_1}{6}$.
This is double of $A_2$. So,
$A_2=\displaystyle\frac{A_1}{2}=\frac{\pi r_1}{12}$
$=\displaystyle\frac{\pi r_2}{36}$, as $r_2=3r_1$.
Expressing $A_2$ in terms of periphery length,
$A_2=\displaystyle\frac{2\pi r_2}{72}$.
It subtends at the centre an angle that is $\frac{1}{72}$th of The angle $360^0$ subtended by the whole periphery at the centre.
So, $\theta=\displaystyle\frac{360^0}{72}=5^0$.
Answer: Option c: $5^0$.
Key concepts used: Relation between arc length and the angle subtended by it at the centre -- Proportionality of arc length to arc angle -- Unitary method-- Solving in mind.
Explanation of the concept of arc length and the angle it subtends at the centre
Think about the question,
What is the length of an arc that subtends an angle of $90^0$ at the centre?
The circle on the left in the following figure shows such an arc.
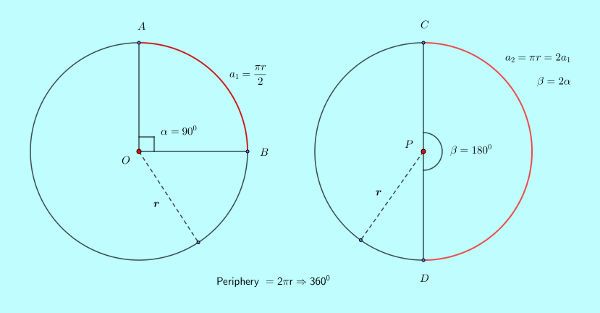
The arc is one-fourth the perimeter, that is, $\displaystyle\frac{\pi}{2} r$, where $r$ is the radius.
And the angle that it subtends at the centre is just, $90^0=\displaystyle\frac{\pi}{2}$.
We can then clearly specify that,
An arc that is $\displaystyle\frac{\pi}{2} r$ units long subtends an angle of $90^0$ at the centre.
Now what will be the situation for the arc shown on the right of the above figure where the arc covers half the perimeter?
In this case, the arc length is just be double of the arc on the left, that is, it is $\pi r$ units long and the angle subtended is also the double of that of the arc on the left, that is, it is $180^0=\pi$.
This confirms the very important property of an arc,
The length of an arc is proportional to the angle it subtends at the centre of the circle.
The reference arc for finding length of any arc subtending a given angle at the centre or vice versa is the whole circle periphery,
Reference arc for finding values of length or angle subtended by any arc, is the whole periphery of the circle of perimeter length $2\pi r$ and angle subtended at the centre $360^0=2\pi$.
This is Reference arc in arc angle proportionality concept.
Arc length being proportional to its angle subtended, unitary method is applied for finding the length of an arc given its angle subtended at the centre and vice versa.
Q3. P is a point outside the circle at a distance of 6.5 cm from centre O of the circle. PR is a secant such that it intersects the circle at Q and R. If $PQ=4.5$ cm, and $QR=3.5$ cm, then what is the radius (in cm) of the circle?
- $2$
- $2.5$
- $3$
- $1.5$
Solution 3: Quick solution by secant segment relation for a circle that is based on external angle property of a chord
The following figure shows the problem graphic.

By external angle property of a chord in circle, the external angle of chord QT formed with tangent at T equals the angle subtended by the chord at point R on the alternate segment of the circle,
$\angle PTQ=\angle TRP$ in $\triangle PTQ$ and $\triangle PRT$ respectively.
The angle $\angle P$ is common the both these two triangles. So the third pair of corresponding angles are also equal,
$\angle PQT=\angle PTR$ in the two triangles $\triangle PTQ$ and $\triangle PRT$ respectively.
By A-A-A condition of similarity, the two triangles are then similar.
By corresponding pair of side ratio equality property in two similar triangles,
$\displaystyle\frac{PT}{RP}=\frac{PQ}{PT}$,
Or, $PT^2=PQ\times{RP}$
$=4.5\times{(3.5+4.5)}=4.5\times{8}=36=6^2$.
This is the secant segment relation for a circle.
Again as radius OT is tangent to tangent section PT, in right $\triangle PTO$ by Pythagoras theorem,
$r^2=(6.5)^2-(6)^2$
$=(6.5+6)(6.5-6)=6.25=(2.5)^2$.
Answer: Option b: $2.5$.
Key concepts used: External angle property of a chord -- Secant segment relation in a circle -- Pythagoras theorem -- Solving in mind.
Q4. In the given figure, O is the incentre of $\triangle ABC$. If $\displaystyle\frac{AO}{OE}=\frac{5}{4}$ and $\displaystyle\frac{CO}{OD}=\frac{3}{2}$, then what is the value of $\displaystyle\frac{BO}{OF}$?

- $\displaystyle\frac{38}{7}$
- $\displaystyle\frac{19}{7}$
- $\displaystyle\frac{19}{14}$
- $\displaystyle\frac{38}{17}$
Solution 4: Quick solution by the concept of Segmentation ratio at the incentre of a triangle and by smart algebraic techniques
By the segmentation relation of the internal angle bisector at the incentre with the triangle sides,
The ratio of two segments of an internal angle bisector equals the ratio of the sum of the two sides adjacent to the angle bisected and the side opposite to the angle bisected.
By this concept, for the internal angle bisector AE and CD respectively in $\triangle ABC$,
$\displaystyle\frac{AO}{OE}=\frac{b+c}{a}=\frac{5}{4}$, where short forms of side names are used, and,
$\displaystyle\frac{CO}{OD}=\frac{a+b}{c}=\frac{2}{3}$.
We know by the above concept, the target ratio value is,
$\displaystyle\frac{BO}{OF}=\frac{c+a}{b}$.
That is why our objective is to form the sum $(c+a)$, and to do it we must equalize the numerators to the same value $(a+b+c)$ and invert the results.
Applying this target oriented smart algebraic technique add 1 to both sides of the two ratios above for the results,
$\displaystyle\frac{a+b+c}{a}=\frac{9}{4}$, and,
$\displaystyle\frac{a+b+c}{c}=\frac{5}{2}$.
This is application of componendo technique.
Now as visualized, invert each and add the two results,
$\displaystyle\frac{c+a}{a+b+c}=\displaystyle\frac{2}{5}+\displaystyle\frac{4}{9}=\displaystyle\frac{38}{45}$.
To get $b$ as the counterpart of ratio term $(c+a)$ in its denominator, we must apply the dividendo technique of subtracting 1 from both sides after inverting the ratio,
$\displaystyle\frac{a+b+c}{c+a}-1=\frac{45}{38}-1$,
Or, $\displaystyle\frac{b}{c+a}=\frac{7}{38}$.
Finally, invert again to get the answer.
$\displaystyle\frac{c+a}{b}=\frac{BO}{OF}=\frac{38}{7}$.
Answer: Option a: $\displaystyle\frac{38}{7}$.
Key concepts used: Triangle side to incentre segment relations -- Target driven input transformation -- Target matching -- Componendo technique-- Dividendo -- Smart algebraic techniques -- Solving in mind.
To understand how the segmentation relation at the incentre comes to be true, read our concept tutorial article,
Basic and Rich Geometry Concepts 8, Segmentation ratio at the incentre of a triangle.
Q5. The lengths of the sides of a triangle are $a$, $b$, and $c$ respectively. If $a^2+b^2+c^2=ab+bc+ca$, then the triangle is,
- right-angled
- scalene
- equilateral
- isosceles
Solution 5: Immediate solution by Zero sum of square terms algebraic principle
Multiply the given relation between the sides by 2. Result is,
$2(a^2+b^2+c^2)=2(ab+bc+ca)$,
Or, $(a^2-2ab+b^2)+(b^2-2bc+c^2)+(c^2-2ca+a^2)=0$,
Or, $(a-b)^2+(b-c)^2+(c-a)^2=0$.
This is a zero sum of square terms or expressions. Each term must individually be zero for their sum to be zero (as squaring would make each positive).
That is,
$(a-b)=(b-c)=(c-a)=0$,
Or, $a=b=c$.
The triangle must be equilateral.
Answer: Option c: equilateral.
Key concepts used: Key pattern identification -- Target pattern driven input transformation -- Collection of like terms together -- Zero sum of square terms principle -- Solving in mind.
The solution of this problem depends mostly on algebraic concepts and techniques.
Q6. In a $\triangle ABC$, the side BC is extended up to D, such that $CD=AC$. If $\angle BAC=109^0$ and $\angle ACB=72^0$, then the value of $\angle ABC$ is,
- $35^0$
- $45^0$
- $40^0$
- $60^0$
Solution 6: Quick solution by angle properties of an external angle of a triangle and an isosceles triangle
The following figure describes the problem situation,
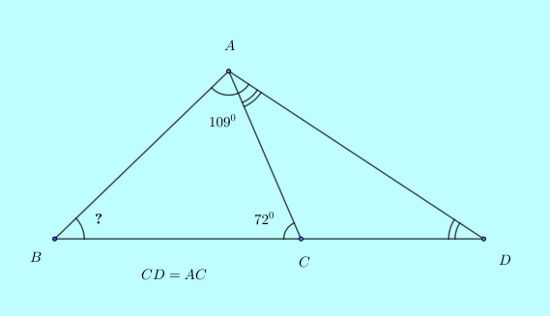
As in isosceles $\triangle CAD$, $AC=CD$,
$\angle CAD=\angle CDA=x$, say.
External angle to $\triangle CAD$,
$\angle ACB=72^0=2x$, sum of opposite angles.
So, $\angle CAD=\angle CDA=36^0$, and,
$\angle BAC=109^0-36^0=73^0$
Finally in $\triangle ABC$,
$\angle ABC=180^0-(73^0+72^0)=35^0$.
Answer: Option a: $35^0$.
Key concepts used: External angle to a triangle -- Isosceles triangle base angle equality property -- Total of angles in a triangle -- Solving in mind.
Q7. In the given figure, area of isosceles $\triangle ABE$ is 72 sq cm, $BE=AB$ and $AB=2AD$. If $AE||DC$, then what is the area (in sq. cm) of the trapezium ABCD?

- $144$
- $124$
- $108$
- $136$
Solution 7: Quick solution by Key pattern identification and area compensating shape introduction technique
The following figure enhanced from the problem figure explains the solution nearly completely,

The additional properties that are to be specified clearly are,
- $\angle ABE=90^0$, and,
- $AD||BC$ as ABCD is a trapezium.
First get the length of BE from the area formula of the $\triangle ABE$,
$72=\frac{1}{2}(BE)^2$, as $\text{height }AB=\text{base }BE$,
Or, $BE=\sqrt{144}=12$ cm.
So, $AD=\frac{1}{2}AB=\frac{1}{2}BE=6$ cm.
AD parallel to BC is extended to meet EF, the perpendicular to BC at point E.
With $\angle B=90^0$, $AB=BE$ and $AB||EF$, $AF||BE$, ABEF is a square of area,
$(12)^2=144$ sq cm.
As $AF=BE$ and $AD=\frac{1}{2}BE$, D is the mid-point of AF and $DF=AD=6$ cm.
Again, AECD is a parallelogram with $AD=EC=DF$.
In the whole process a new element $\triangle DGF$ is introduced. This triangle is congruent to $\triangle GEC$ as all three pairs of angles are equal as well as one pair of sides, $DF=EC$.
Area of these two triangles are then equal.
If you cut-out $\triangle GEC$ from trapezium ABCD and add it as $\triangle DGF$, you get the square ABEF of area 144 sq cm that is same as the area of the trapezium.
This is new geometric element introduction technique together with compensation technique.
Answer: Option a: $144$.
Key concepts used: Area of triangle as half of product of base and height -- Key pattern identification of ABEF as a square -- New geometric element introduction technique -- Compensation principle -- Trapezium concepts -- Solving in mind.
There is little calculation, only identifying key pattern, applying basic concepts and applying the compensation technique together with new geometric element introduction technique.
Q8. $\triangle XYZ$ is similar to $\triangle PQR$. If ratio of perimeter of $\triangle XYZ$ and perimeter of $\triangle PQR$ is $16:9$ and $PQ=3.6$ cm, then what is the length (in cm) of $XY$?
- $3.2$
- $4.8$
- $8.6$
- $6.4$
Solution 8: Quick solution by corresponding side ratio equality of similar triangles, chained equation treatment and ratio concepts
In similar triangles $\triangle XYZ$ and $\triangle PQR$, the ratio of three pairs of corresponding sides are equal,
$\displaystyle\frac{XY}{PQ}=\frac{YZ}{QR}=\frac{ZX}{RS}=a$, say.
So,
$XY=a.PQ$,
$YZ=a.QR$, and,
$ZX=a.RS$.
Sum up three equations and you would get the relation between the perimeters,
$(XY+YZ+ZX)=a(PQ+QR+RS)$,
Or, $\displaystyle\frac{XY+YZ+ZX}{PQ+QR+RS}=\frac{16}{9}=a$.
We have to find length of XY. So we have to use the ratio of sides involving XY,
$XY=a.PQ=\displaystyle\frac{16}{9}\times{3.6}=16\times{0.4}=6.4$ cm.
Answer: Option d: $6.4$.
Key concepts used: Side ratio equality of similar triangles -- Chained equation treatment by using dummy variable $a$ -- Perimeter of a triangle -- Ratio concept -- Solving in mind.
Q9. PQ is a diameter of a circle with centre O. RS is a chord parallel to PQ that subtends an angle of $40^0$ at the centre of the circle. If PR and QS are produced to meet at T, then what will be the measure (in degrees) of $\angle PTQ$?
- $55$
- $90$
- $60$
- $70$
Solution 9: Quick solution by Key pattern identification of formation of isosceles triangles and total of angles of a triangle concept
The following figure describes the problem and helps explaining the solution.

The concepts required to solve the problem are really basic.
Identify that $\triangle ORS$, $\triangle OPR$ and $\triangle OQS$ are all isosceles as radii pairs, $OR=OS$, $OP=OR$ and $OQ=OS$. This results in the pairs of base angles equal in the three triangles.
First let us see the status in $\triangle ORS$.
With $\angle ROS=40^0$,
$2\angle ORS=180^0-40^0=140^0$,
Or, $\angle ORS=\angle OSR=70^0$.
Next, as $PO||RS$, opposite internal angles are equal,
$\angle ORS=\angle POR=70^0$.
Again in isosceles $\triangle OPR$,
$2\angle OPR=180^0-70^0=110^0$,
Or, $\angle OPR=\angle ORP=55^0$.
In the same way,
$\angle OQS=\angle OSQ=55^0$.
Finally in larger $\triangle PTQ$, with two base angle equal to $55^0$, the apex angle value evaluates to,
$\angle PTQ=180^0-2\times{55^0}=70^0$.
Answer: Option d: $70$.
Key concepts used: Key pattern identification of isosceles triangles, angle equality for a line intersecting a pair of parallel lines and total of angles in a triangle concept.
Q10. A circle is inscribed in a $\triangle ABC$. It touches the sides AB, BC and AC at points R, P and Q respectively. If $AQ=4.5$ cm, $PC=5.5$ cm and $BR=6$ cm, then perimeter of the triangle is,
- $26.5$ cm
- $30.5$ cm
- $32$ cm
- $28$ cm
Solution 10: Quick solution by Congruent triangles formation by two tangents to a circle from an external point and Key pattern identification
The following figure describes the problem,
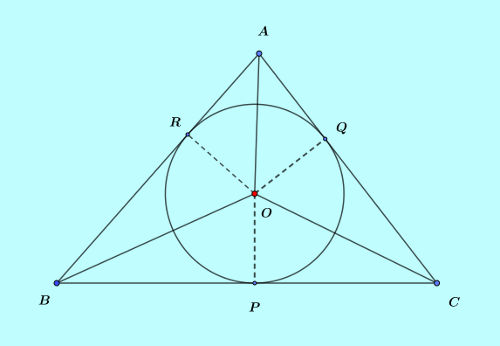
From the external point A, the tangents AR and AQ to the circle forms two congruent triangles at centre O,
$\triangle ARO \cong \triangle AQO$.
This is a basic property of two tangents to a circle from a single external point.
Reason: AO the line segment from A to incentre O of $\triangle ABC$ bisects the $\angle A$, that is,
$\angle RAO=\angle QAO$.
Also, radii OR and OQ are perpendicular to the tangent at tangent points R and O forming a second equal pair of angles of value $90^0$.
So the third pair of angles are also equal,
$\angle ROA=\angle QOA$.
By A-A-A similarity criterion, the two triangles $\triangle ARO$ and $\triangle AQO$ are similar.
But in addition the pair of radii, $OR=OQ$.
This makes the two triangles $\triangle ARO$ and $\triangle AQO$ congruent, and so,
$AR=AQ=4.5$ cm.
Similarly,
$PC=QC=5.5$ cm, and,
$BR=BP=6$ cm.
Sum of the three given line segments 16 cm is then just half of the sum of the three sides or perimeter of the $\triangle ABC$.
Thus perimeter of the triangle is,
$AB+BC+CA$
$=(AR+BR)+(BP+PC)+(AQ+QC)$
$=2\times{16}=32$ cm.
Answer: Option c: $32$ cm.
Key concepts used: Two tangents to a circle from an external point -- Tangents from a common point -- Congruent triangles -- Internal angle bisectors of a triangle meet at the incentre -- Key pattern identification -- Perimeter concept -- Solving in mind.
End note
Observe that, each of the problems could be quickly and cleanly solved in minimum number of steps using basic and rich geometry concept based key patterns and methods in each case.
This is the hallmark of quick problem solving:
- Concept based pattern and method formation, and,
- Identification of the key pattern and use of the method associated with it. Every special pattern has its own method, and not many such patterns are there.
Important is the concept based pattern identification and use of quick problem solving method.
Guided help on Geometry in Suresolv
To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,
The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.
