Can you solve it in 20 secs? Learn How in SSC CHSL Trigonometry 1

Submitted by Atanu Chaudhuri on Thu, 11/06/2020 - 20:19

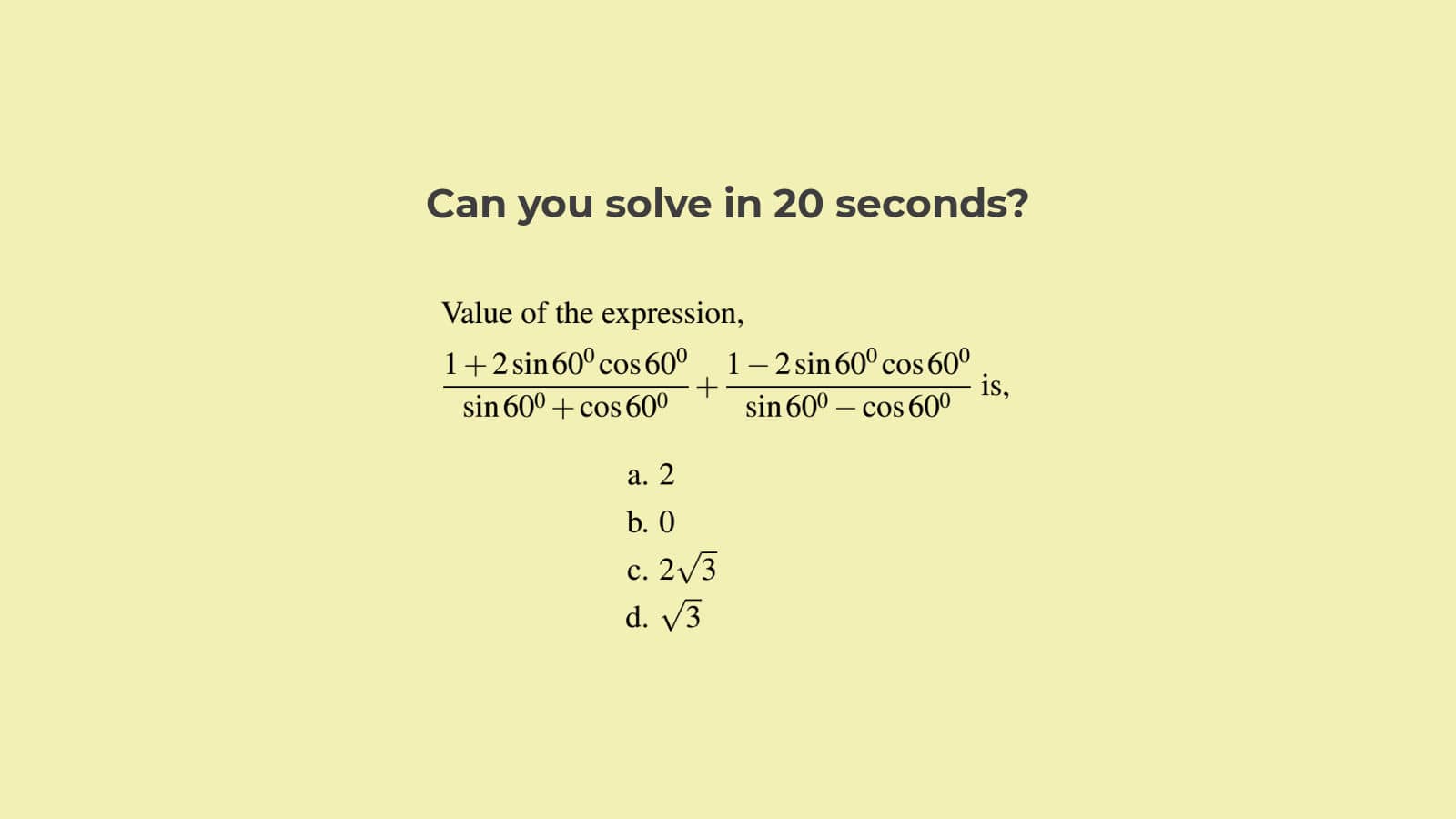
SSC CHSL Trigonometry solved questions set 1

Trigonometry problem solving - SSC CHSL Trigonometry solved questions set 1

Take the test first and then learn how to solve 10 selected SSC CHSL Trigonometry questions set 1 quickly in few steps. Learn trigonometry problem solving.

It contains,

SSC CHSL Trigonometry Questions to be answered in 15 minutes (10 chosen questions)
Answers to the questions, and
Detailed quick solutions to the questions.

For best results, take the timed test first, verify answers and then learn how to solve quickly from the solutions.

IMPORTANT: To absorb the concepts, techniques and reasoning explained in the solutions fully and apply those in solving problems on Trigonometry quickly, one must solve many problems in a systematic manner using the conceptual analytical approach.

Learning by doing is the best learning. There is no other alternative towards achieving excellence.

10 Selected SSC CHSL Trigonometry Questions Set 1 - answering time 15 mins

Q1. If $\sin (60^0-x)=\cos (y+60^0)$, then the value of $\sin (x-y)$ is,

$\displaystyle\frac{1}{2}$
$\displaystyle\frac{1}{\sqrt{2}}$
$1$
$\displaystyle\frac{\sqrt{3}}{2}$

Q2. If $4\sin^2 \theta -1=0$, and angle $\angle \theta$ is less than $90^0$, then the value of $\cos^2 \theta+\tan^2 \theta$ is, (Take $0^0 \lt \theta \lt 90^0$)

$\displaystyle\frac{17}{15}$
$\displaystyle\frac{12}{11}$
$\displaystyle\frac{13}{12}$
$\displaystyle\frac{11}{9}$

Q3. Value of the expression $\displaystyle\frac{1+2\sin 60^0 \cos 60^0}{\sin 60^0+\cos 60^0}+\displaystyle\frac{1-2\sin 60^0 \cos 60^0}{\sin 60^0-\cos 60^0}$ is,

$2$
$0$
$2\sqrt{3}$
$\sqrt{3}$

Q4. If $\cos \theta+\sin \theta=m$ and $\text{sec }\theta+\text{cosec }\theta=n$, then the value of $n(m^2-1)$ is equal to,

$2m$
$2n$
$mn$
$4mn$

Q5. Find the numerical value of $\displaystyle\frac{9}{\text{cosec}^2 \theta}+4\cos^2 \theta+\displaystyle\frac{5}{1+\tan^2 \theta}$.

Q6. If $\displaystyle\frac{1}{\tan A+\text{cot A} }=x$, then the value of $x$ is,

$\cos A\sin A$
$\text{cosec }A\text{ sec }A$
$\text{cosec}^2 A\text{ sec}^2 A$
$\cos^2 A\sin^2 A$

Q7. If $\sin C-\sin D=x$, then the value of $x$ is,

$2\sin \left[\displaystyle\frac{C+D}{2}\right]\cos \left[\displaystyle\frac{C-D}{2}\right]$
$2\cos \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$
$2\cos \left[\displaystyle\frac{C+D}{2}\right]\cos \left[\displaystyle\frac{C-D}{2}\right]$
$2\sin \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$

Q8. if $\cos 45^0 -\text{sec }60^0=x$, then the value of $x$ is,

$\displaystyle\frac{\sqrt{3}-4}{2\sqrt{3}}$
$\displaystyle\frac{\sqrt{3}+\sqrt{2}}{2}$
$1$
$\displaystyle\frac{1-2\sqrt{2}}{\sqrt{2}}$

Q9. If $\displaystyle\frac{1+\cos A}{2}=x$, then the value of $x$ is,

$\sin^2\left(\displaystyle\frac{A}{2}\right)$
$\cos^2\left(\displaystyle\frac{A}{2}\right)$
$\sqrt{\sin \left(\displaystyle\frac{A}{2}\right)}$
$\sqrt{\cos \left(\displaystyle\frac{A}{2}\right)}$

Q10. If $\text{sec }\theta+\text{cosec } \theta=\sqrt{2}\text{sec }(90^0-\theta)$ then what is the value of $\text{cot } \theta$?

$\sqrt{2}+1$
$\sqrt{2}-1$
$2$
$\sqrt{2}$

Answers to the SSC CHSL Trigonometry Questions Set 1

Q1. Answer: Option a: $\displaystyle\frac{1}{2}$.

Q2. Answer: Option c: $\displaystyle\frac{13}{12}$.

Q3. Answer: Option d: $\sqrt{3}$.

Q4. Answer: Option a: $2m$.

Q5. Answer: Option b: $9$.

Q6. Answer: Option a : $\cos A\sin A$.

Q7. Answer: Option b: $2\cos \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$.

Q8. Answer: Option d: $\displaystyle\frac{1-2\sqrt{2}}{\sqrt{2}}$.

Q9. Answer: Option b: $\cos^2\left(\displaystyle\frac{A}{2}\right)$.

Q10. Answer: Option a: $\sqrt{2}+1$.

Quick solutions to the SSC CHSL Trigonometry Questions Set 1 - answering time was 15 mins

Q1. If $\sin (60^0-x)=\cos (y+60^0)$, then the value of $\sin (x-y)$ is,

$\displaystyle\frac{1}{2}$
$\displaystyle\frac{1}{\sqrt{2}}$
$1$
$\displaystyle\frac{\sqrt{3}}{2}$

Solution 1: Quick solution by Complementary angle trigonometric relations

By complementary angle trigonometric relations, when sum of two angles $\angle \alpha$ and angle $\angle \beta$ is $90^0$,

$\sin \alpha=\cos \beta=\sin (90^0-\beta)$, and,

$\cos \alpha=\sin \beta=\cos (90^0-\beta)$.

Applying complementary angle trigonometric relations on the given equation,

$\sin (60^0-x)=\cos (y+60^0)$,

$=\sin(90^0-y-60^0)=\sin(30^0-y)$,

Or, $60^0-x=30^0-y$,

Or, $x-y=60^0-30^0=30^0$.

So, $\sin (x-y)=\sin 30^0=\displaystyle\frac{1}{2}$.

Answer: Option a: $\displaystyle\frac{1}{2}$.

Key concepts used: Complementary angle trigonometric relations -- Solving in mind.

If you know the concepts and discover the key pattern, you will surely be able to solve the problem in mind.

Q2. If $4\sin^2 \theta -1=0$, and angle $\angle \theta$ is less than $90^0$, then the value of $\cos^2 \theta+\tan^2 \theta$ is, (Take $0^0 \lt \theta \lt 90^0$)

$\displaystyle\frac{17}{15}$
$\displaystyle\frac{12}{11}$
$\displaystyle\frac{13}{12}$
$\displaystyle\frac{11}{9}$

Solution 2: Quick solution by finding the value of $\angle \theta$ from the given equation and applying it on the target expression

Find the value of $\angle \theta$ directly from the given equation,

$4\sin^2 \theta -1=0$,

Or, $\sin^2 \theta=\displaystyle\frac{1}{4}$,

Or, $\sin \theta=\displaystyle\frac{1}{2}=\sin 30^0$, with $\theta$ acute $\sin \theta$ cannot be negative.

So, $\angle \theta=30^0$.

At $30^0$ the values of $\cos \theta$ and $\tan \theta$ are,

$\cos \theta=\cos 30^0=\displaystyle\frac{\sqrt{3}}{2}$, and,

$\tan \theta=\tan 30^0=\displaystyle\frac{\sin 30^0}{\cos 30^0}=\frac{1}{\sqrt{3}}$.

Substituting,

$\cos^2 \theta+\tan^2 \theta=\displaystyle\frac{3}{4}+\displaystyle\frac{1}{3}=\displaystyle\frac{13}{12}$.

Answer: Option c: $\displaystyle\frac{13}{12}$.

Key concepts used: Trigonometric function values at specific angles -- Solving in mind.

Alternate solution that is quicker

Get the value of $\sin^2 \theta$ first,

$4\sin^2 \theta -1=0$,

Or, $\sin^2 \theta=\displaystyle\frac{1}{4}$.

Simplify the target expression,

$E=\cos^2 \theta+\tan^2 \theta$

$=(1-\sin^2 \theta)+(\sec^2 \theta -1)$

$=-\displaystyle\frac{1}{4}+\displaystyle\frac{1}{1-\sin^2 \theta}$

$=-\displaystyle\frac{1}{4}+\displaystyle\frac{4}{3}$

$=\displaystyle\frac{13}{12}$.

This solution can easily be done in mind.

Q3. Value of the expression $\displaystyle\frac{1+2\sin 60^0 \cos 60^0}{\sin 60^0+\cos 60^0}+\displaystyle\frac{1-2\sin 60^0 \cos 60^0}{\sin 60^0-\cos 60^0}$ is,

$2$
$0$
$2\sqrt{3}$
$\sqrt{3}$

Solution 3: Quick solution key pattern identification and Other way round approach of splitting 1 to $(\sin^2 60^0+\cos^2 60^0)$

This is the challenge question.

Can you solve it in 20 secs?

Yes. Of course.

Identify the key possibility of expanding 1 in both numerators as,

$1=\sin^2 60^0+\cos^2 60^0$.

What happens?

The two numerators are transformed to respectively,

$(\sin 60^0+\cos 60^0)^2$, and,

$(\sin 60^0-\cos 60^0)^2$.

Now look at the respective denominaors. Both cancel out and the target expression is simplified to,

$(\sin 60^0+\cos 60^0)+(\sin 60^0-\cos 60^0)=2\sin 60^0=\sqrt{3}$.

Answer: Option d: $\sqrt{3}$.

All in mind and well within 20 secs if you can identify the possibility and use the trigonometry problem solving technique of expanding 1 to $(\sin^2 60^0+\cos^2 60^0)$

Note: Usually, you use the relation $(\sin^2 \theta+\cos^2 \theta)=1$ to simplify trigonometric relations. But here, using the Other way round approach, you are expanding the 1 to $(\sin^2 \theta+\cos^2 \theta)$ using the relation in reverse direction, that is, $1=(\sin^2 \theta+\cos^2 \theta)$.

This is key pattern identification along with application of Other way round approach, a powerful problem solving approach.

To show you the steps explicitly,

$\displaystyle\frac{1+2\sin 60^0 \cos 60^0}{\sin 60^0+\cos 60^0}+\displaystyle\frac{1-2\sin 60^0 \cos 60^0}{\sin 60^0-\cos 60^0}$

$=\displaystyle\frac{\sin^2 60^0+\cos^2 60^0+2\sin 60^0 \cos 60^0}{\sin 60^0+\cos 60^0}$

$\hspace{10mm}+\displaystyle\frac{\sin^2 60^0+\cos^2 60^0-2\sin 60^0 \cos 60^0}{\sin 60^0-\cos 60^0}$

$=\displaystyle\frac{(\sin 60^0+\cos 60^0)^2}{\sin 60^0+\cos 60^0}+\displaystyle\frac{(\sin 60^0-\cos 60^0)^2}{\sin 60^0-\cos 60^0}$

$=(\sin 60^0+\cos 60^0)+(\sin 60^0-\cos 60^0)$

$=2\sin 60^0=\sqrt{3}$.

Answer: Option d: $\sqrt{3}$.

Key concepts used: Key pattern identification -- Other way round approach -- Expanding $1$ to $(\sin^2 60^0+\cos^260^0)$ -- Solving in mind.

Q4. If $\cos \theta+\sin \theta=m$ and $\text{sec }\theta+\text{cosec }\theta=n$, then the value of $n(m^2-1)$ is equal to,

$2m$
$2n$
$mn$
$4mn$

Solution 4: Quick solution by key pattern identification of the simplified evaluated form of the second factor $(m^2-1)$

Evaluate $(m^2-1)=2\sin \theta\cos \theta$. Mentally form the product of this result with each term of $n=\text{sec }\theta+\text{cosec }\theta$. Final result is simply $2m$.

The steps are: target expression,

$n(m^2-1)$

$=(\text{sec }\theta+\text{cosec }\theta)\left[(\cos \theta+\sin \theta)^2-1\right]$

$=(\text{sec }\theta+\text{cosec }\theta)(1+2\sin \theta\cos \theta-1)$, first simplify the second factor,

$=2\sin \theta\cos \theta(\text{sec }\theta+\text{cosec }\theta)$

$=2(\sin \theta+\cos \theta)=2m$.

Answer: Option a: $2m$.

Key concepts used: Key pattern identification of simplified form of second factor -- Solving in mind.

Q5. Find the numerical value of $\displaystyle\frac{9}{\text{cosec}^2 \theta}+4\cos^2 \theta+\displaystyle\frac{5}{1+\tan^2 \theta}$.

Solution 5: Solve quickly by key pattern identification by simplifying the last term first

First simplify the last term, combine the result with the second and then combine this result with the transformed first term.

You do this as you identify the key pattern that working in this right to left direction instead of left to right processing would give you the answer in quickest time.

So, the third term denominator is simplified to $1+\tan^2 \theta=\text{sec}^2 \theta$.

Invert it to form the term as, $5\cos^2 \theta$.

Add it to the second term to get the result as, $9\cos^2 \theta$

Invert the denominator of the first term now to convert the term to $9\sin^2 \theta$.

Final result is thus simply,

$9(\sin^2 \theta+\cos^2 \theta)=9$.

Answer: Option b. $9$.

Key concepts used: Key pattern identification of conversion of third term first and working backwards -- Solving in mind.

Let us show you the deductions in more details.

The given expression,

$\displaystyle\frac{9}{\text{cosec}^2 \theta}+4\cos^2 \theta+\displaystyle\frac{5}{1+\tan^2 \theta}$

$=\displaystyle\frac{9}{\text{cosec}^2 \theta}+4\cos^2 \theta+\displaystyle\frac{5}{\text{sec}^2 \theta}$

$=\displaystyle\frac{9}{\text{cosec}^2 \theta}+4\cos^2 \theta+5\cos^2 \theta$

$=\displaystyle\frac{9}{\text{cosec}^2 \theta}+9\cos^2 \theta$

$=9(\sin^2 \theta+\cos^2 \theta)=9$.

Q6. If $\displaystyle\frac{1}{\tan A+\text{cot A} }=x$, then the value of $x$ is,

$\\sin A\cos A$
$\text{cosec }A\text{sec }A$
$\text{cosec}^2 A\text{sec}^2 A$
$\cos^2 A\sin^2 A$

Solution 6: Quick solution adopting Variable reduction technique by converting $\text{cot } A$ to $\tan A$

A problem always is simplified by reducing the number of variables involved. Here the variables are two, $\tan A$ and $\text{cot } A$.

Reduce number of variables to 1 by converting $\text{cot } A$ to $\tan A$ getting,

$\displaystyle\frac{1}{\tan A+\text{cot A} }=x$,

Or, $\displaystyle\frac{1}{\tan A+\displaystyle\frac{1}{\tan A} }=x$,

Or, $x=\displaystyle\frac{\tan A}{1+\tan^2 A}$.

So, $x=\displaystyle\frac{\tan A}{\text{sec}^2 A}=\sin A \cos A$.

Answer: Option a: $\sin A \cos A$.

Key concepts used: Variable reduction technique by function conversion -- Solving in mind.

Q7. If $\sin C-\sin D=x$, then the value of $x$ is,

$2\sin \left[\displaystyle\frac{C+D}{2}\right]\cos \left[\displaystyle\frac{C-D}{2}\right]$
$2\cos \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$
$2\cos \left[\displaystyle\frac{C+D}{2}\right]\cos \left[\displaystyle\frac{C-D}{2}\right]$
$2\sin \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$

Solution 7: Quick solution by Compound angle trigonometric relations and substitution

The compound angles in choices indicate that you have to use compound angle relationships,

$\sin (\alpha +\beta)=\sin \alpha\cos \beta+\cos \alpha\sin \beta$, and

$\sin (\alpha -\beta)=\sin \alpha\cos \beta-\cos \alpha\sin \beta$.

Subtracting the second from the first you get,

$\sin (\alpha +\beta)-\sin (\alpha +\beta)=2\cos \alpha\sin \beta$.

Now substitute,

$C=\alpha +\beta$, and,

$D=\alpha -\beta$.

So,

$\displaystyle\frac{C+D}{2}=\alpha$, and,

$\displaystyle\frac{C-D}{2}=\beta$.

Substituting back, finally you get,

$\sin C-\sin D=2\cos \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$.

Answer: Option: b: $2\cos \left[\displaystyle\frac{C+D}{2}\right]\sin \left[\displaystyle\frac{C-D}{2}\right]$.

Key concepts used: Compound angle relationships -- Suitable variable substitution -- Solving in mind.

Q8. if $\cos 45^0 -\text{sec }60^0=x$, then the value of $x$ is,

$\displaystyle\frac{\sqrt{3}-4}{2\sqrt{3}}$
$\displaystyle\frac{\sqrt{3}+\sqrt{2}}{\sqrt{2}}$
$1$
$\displaystyle\frac{1-2\sqrt{2}}{\sqrt{2}}$

Solution 8: Quick solution by direct substitution of trigonometric function values for specific angles

Substitute,

$\cos 45^0=\displaystyle\frac{1}{\sqrt{2}}$, and,

$\text{sec }60^0=2$.

The result you get from the given equation,

$x=\cos 45^0 -\text{sec }60^0=\displaystyle\frac{1}{\sqrt{2}}-2=\displaystyle\frac{1-2\sqrt{2}}{\sqrt{2}}$.

Answer: Option d: $\displaystyle\frac{1-2\sqrt{2}}{\sqrt{2}}$.

Key concepts used: Trigonometric function values for specific angles -- Solving in mind.

Q9. If $\displaystyle\frac{1+\cos A}{2}=x$, then the value of $x$ is,

$\sin^2\left(\displaystyle\frac{A}{2}\right)$
$\cos^2\left(\displaystyle\frac{A}{2}\right)$
$\sqrt{\sin \left(\displaystyle\frac{A}{2}\right)}$
$\sqrt{\sin \left(\displaystyle\frac{A}{2}\right)}$

Solution 9: Quick solution using Compound angle relations

From the choice values you know that you have to use the expanded relation for $\cos 2\alpha$.

Using the basic compound relation for $\cos(\alpha+\beta)$ with $\angle \alpha=\angle \beta$,

$\cos(\alpha+\beta)=\cos 2\alpha=\cos^2 \alpha-sin^2 \alpha$.

To match the problem variables substitute $A=2\alpha$. Result is,

$\cos A=\cos^2 \left(\displaystyle\frac{A}{2}\right)-\sin^2 \left(\displaystyle\frac{A}{2}\right)=2\cos^2 \left(\displaystyle\frac{A}{2}\right)-1$,

Or, $1+\cos A=2\cos^2 \left(\displaystyle\frac{A}{2}\right)$.

Dividing by 2 you will get the value of $x$ as,

$x=\displaystyle\frac{1+\cos A}{2}=\cos^2 \left(\displaystyle\frac{A}{2}\right)$.

This is effectively use of submultiple angle trigonometric relations.

Answer: Option b: $\cos^2 \left(\displaystyle\frac{A}{2}\right)$.

Key concepts used: Compound angle trigonometric relations -- Submultiple angle trigonometric relations -- Solving in mind.

Q10. If $\text{sec }\theta+\text{cosec } \theta=\sqrt{2}\text{sec }(90^0-\theta)$ then what is the value of $\text{cot } \theta$?

$\sqrt{2}+1$
$\sqrt{2}-1$
$2$
$\sqrt{2}$

Solution 10: Quick solution by target driven simplification of given equation using complementary angle trigonometric relation

The given equation is,

$\text{sec }\theta+\text{cosec } \theta=\sqrt{2}\text{sec }(90^0-\theta)=\sqrt{2}\text{cosec }\theta$,

Or, $\text{sec }\theta=(\sqrt{2}-1)\text{cosec }\theta$,

Or, $\text{cot } \theta=\displaystyle\frac{1}{\sqrt{2}-1}$.

Rationalize the denominator by multiplying both numerator and denominator of the RHS by $(\sqrt{2}+1)$,

$\text{cot } \theta=\sqrt{2}+1$.

Answer: Option a: $\sqrt{2}+1$.

Key concepts used: Target driven simplification -- Surd rationalization -- Solving in mind.

End note

Observe that, each of the problems could be quickly and cleanly solved in minimum number of mental steps using special key patterns and methods in each case.

This is the hallmark of pattern based quick problem solving:

Concept based pattern and method formation, and,

Identification of the key pattern and use of the method associated with it. Every special pattern has its own method, and not many such patterns are there.

Important is the concept based pattern identification and use of quick problem solving method.

SSC CHSL level Question and Solution sets

Trigonometry

SSC CHSL level Solved Question set 15 on Trigonometry 1

SSC CHSL level Solved Question set 16 on Trigonometry 2

SSC CHSL Trigonometry

Trigonometry problem solving

You are here

SSC CHSL Solved question Set 15, Trigonometry 1