Geometry basic concepts Part 1, points lines and triangles

Concepts on Geometry basics points lines and triangles with examples and exercise

Geometry basics, points lines and triangles: Straight lines, parallel lines, angles, triangles, properties of triangles with examples and exercise.

Basic Geometry concepts covered are,

1. Points and lines: Straight line and curved line, Closed shape, Parallel lines, Distance between two lines, angle between two lines, laws of intersection, line intersecting a pair of parallel lines,
2. Angles and types of angles,
3. Triangles: Basic concepts on triangles, External angle of a triangle, Types of triangles, Pythagoras theorem, Isosceles and equilateral triangles, Right-angled triangles, Similarity and Congruency of triangles, Conditions for similarity and Congruency, Triangle medians and angle bisectors, Incentre, Orthocentre and Centroid of a triangle.
4. Ten selected test level exercise questions on Geometry basics points lines and triangles with answer.

Points and lines

To form a shape you need building blocks. Any shape is formed by surfaces, any surface is formed by lines, and finally any line is formed by points. It just like building a solid object from atomic particles.

Point:

A point in space (3D or 2D) is defined as a unique location without any dimension. A point has no length, breadth, or height. It just is a location identified by parameters relative to a reference point. In co-ordinate plane geometry you use two perpendicularly intersecting axes $x$ and $y$ and define the location of any point with reference to the shortest distance of the point from the $x$ axis and the $y$ axis as a pair of values $(x, y)$. The reference point in this case is the intersecting point of the two axes. It is called the origin and has the co-ordinates $(0,0)$.

On a piece of paper or on a plane you are at liberty to place the origin at any convenient location and define all other points on the plane with reference to this origin.

But not always you need the reference point to study the properties of shapes. In other words, not always you need co-ordinate geometry. We will mainly discuss here pure geometry but occasionally use the two axes for clarity.

In real world, however small a point you draw on a piece of paper it would still have a dimension. So, the definition of a point that it is dimensionless is an ideal one and is called an Axiom.

A line:

When you connect two points you get a line. Lines are of two types:

Straight line and curved line:

You connect two points by many lines, in fact by infinite number of lines.

The shortest line connecting two points is a straight line.

All other lines connecting two points are curved lines and length of those lines are more than the straight line connecting the same two points.

Points make a line:

A line consists of infinite number of points one after the other. Following these points you reach from one end of a line to the other.

Ends of a line and closed shapes:

A line, straight or curved has two ends unless these two ends meet. A line can be of finite length or you can imagine each end of a line to extend indefinitely.

If the two ends of a line meet, the line must be a curved line, and it gives rise to a closed shape with curved perimeter.

Examples are Circles or Ellipses.

Such closed shapes with curved perimeter has only a single line bounding an area and the single line has no ends.

Any shape bounded by only straight lines consists of more than two lines (at least 3) and the meeting points of these lines are called vertices.

The closed shape bounded by smallest number of straight lines is a Triangle and is one of the most important geometric shapes in mathematics, not only geometry, but in other disciplines too.

Examples of a straight line and curved lines between two points:

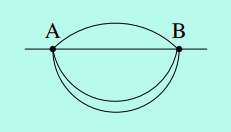
All the three curved lines connecting the two points $A$ and $B$ are longer than the straight line distance $AB$ between $A$ and $B$. There can be such infinite number of curved lines between two points but only one straight line connecting the two points.

The straight line may be of fixed definite length $AB$ but also both its ends may be continued indefinitely on two sides.

Use of straight lines in real life:

Straight lines are the edges of tables, the vertical edge where two walls of a room meet, the edges of your writing pad and so on. Producing a straight line edge wastes least amount of a material and also is easy to create in any manufacturing process. That's why all around we see straight line edges.

Parallel lines:

When two lines never meet when extended in both ends indefinitely, two reasons might be there, either the lines are not in a same plane or the lines are in the same plane but are parallel.

Two parallel lines on a plane never meet.

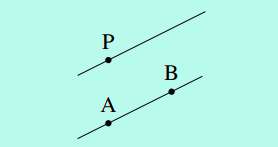
Example of a pair of parallel lines:

Distance between two lines:

Distance between two lines usually vary from point to point. It is defined as the shortest distance from a point on one line to the other line.

In case of two parallel lines $AB$ and $PQ$, the shortest distance from point $P$ on line $PQ$ to the second line $AB$ would be the length of the perpendicular drawn from $P$ onto $AB$, that is, $PC$. This is the distance from point $P$ to line $AB$. Similarly the distance from a point $B$ on line $AB$ to line $PQ$ would be the length of the perpendicular $BD$.

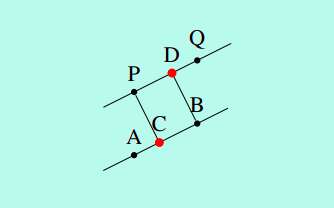
For a pair of parallel lines these two lengths will always be the same. Thus, for any point $P$ on $PQ$ and any point $B$ and $AB$, perpendicular distances, $ PC = BD$.

As distances between the parallel lines do not change, they can never come nearer and meet.

In contrast, for two intersecting lines the distance between two lines reduce as you approach towards the intersecting point at which it becomes zero.

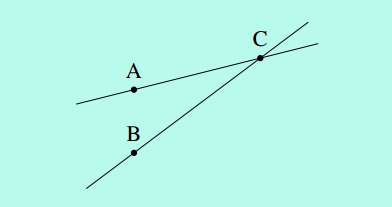
The two lines $AC$ and $BC$ meet at $C$ where distance between the two becomes zero. After the crossing at $C$ the inter-line distance again goes on increasing.

Angle between two lines:

Till now we have not talked about angles as this is the first time we have encountered intersecting lines and question of an angle comes only when two lines intersect. That's why we say angle between two lines.

At this stage we simply define angle as,

Amount of inclination of one line with respect to the other.

Obviously, angle between two parallel lines is zero.

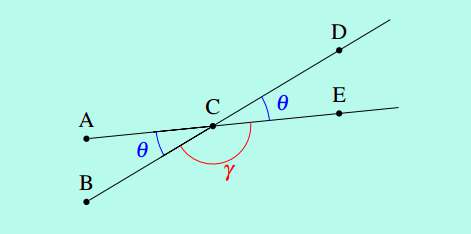
When two straight lines $ACE$ and $BCD$ intersect at a point $C$, the intersection creates four angles, namely, $\angle BCE$, $\angle ECD$, $\angle DCA$, and $\angle ACB$.

Laws of intersection:

Law 1: The opposite intersecting angles will be equal to each other. It means here,

$ \angle ACB = \angle ECD = \theta, \qquad \text{and,} \quad \angle BCE = \angle DCA = \gamma $

Law 2: Sum of the four angles at the intersection will be $360^0$ or $2\pi$. It means here,

$ 2\theta + 2\gamma = 360^0 = 2\pi \qquad \text{or, } \quad \theta + \gamma = 180^0 = \pi $

Straight line intersecting two parallel lines:

We will examine now the important aspects of a straight line intersecting two parallel lines.

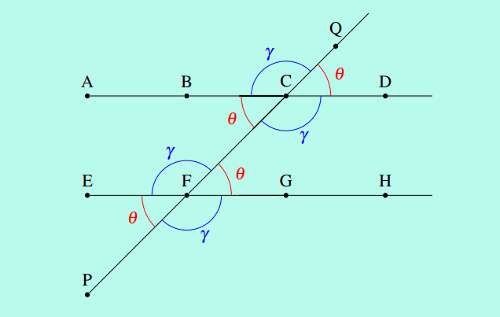
Here also two laws of intersection work on the eight (8) angles created by the straight line intersecting two parallel lines.

Law 3: Angles held by the two parallel lines with the intersecting line on the same side of the intersecting line: Outside angle $\angle DCQ$ and Inside angle of the second line $\angle CFH$ are equal. This law results in four equalities,

$ \angle DCQ = \angle GFC, \quad \angle BCF = \angle EFP, \quad \angle QCB = \angle CFE, \quad \angle FCD = \angle PFH $

Law 4: Inside opposite angles held by the two parallel lines with the intersecting line, $\angle BCF = \angle GFC$.

This is not really a separate independent law - it is derived law fron Law 1 of line intersection and the Law 3 of parallel line intersection.

$\angle BCF = \angle QCD$, by Law 1, $\angle QCD = \angle GFC$, by Law 3, and combining,

$\angle BCF = \angle GFC$. That's why we call this Law 4 as derived from Law 1 and Law 3.

These two Laws, Law 3 and Law 4 results in finally only two unique different values of eight angles created by the intersecting straight line,

$ \angle DCQ = \angle GFC = \angle BCF = \angle EFP = \theta, $

and, 

$\angle QCB = \angle FCD = \angle CFE = \angle PFH = \gamma $

Angles:

Conventionally, angles are measured by rotating a radial line centred at the origin anti-clock-wise. At the start the rotating line rests along the $x$-axis pointing to the right. This is the angle zero.

• As it starts rotating the line separates from the $x$-axis and its inclination with the starting position or $x$-axis is the angular separation or the angle held by it with the $x$-axis.
• When the rotating line stands vertically pointing upwards, it has moved through an angle of $90^0$ or $\pi$/2 radian.
• This is quarter of the full revolution. One quarter later it again rests along the $x$-axis but pointing away to the left. The angle it has moved is $180^0$ or $\pi$ radian.
• Pointing straight downwards after one more quarter, it has traversed $270^0$ or $3\pi$/4.
• Finally after a full revolution, when it reaches its original position, it has traveled through an angle of $360^0$ or $2\pi$ radian.

Angles are of three types:

Acute angle which is less than $90^0$,

Obtuse angle which is more than $90^0$ and,

Right angle which is $90^0$.

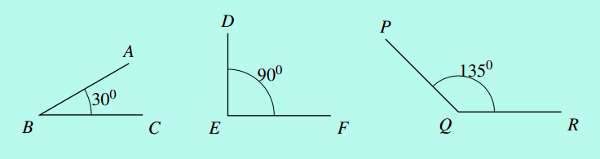
The first angle $\angle ABC = 30^0$ is an acute angle, the second angle $\angle DEF = 90^0$ is a right angle and the third angle $\angle PQR=135^0$ is an obtuse angle.

Triangles

Basic concepts on triangle

• A triangle can be defined as - three points on a plane, not lying on a straight line and connected to each other by three straight lines enclosing a space on a plane.
• Thus a triangle has three vertices connected by three edges.
• A triangle is the closed shape bounded by smallest number of edges or sides. A quadrangle has 4 sides, a pentagon has 5 sides, an hexagon has 6 sides and so on.
• Total of the three angles of a triangle is $180^0$.
• Total length of any two sides of a triangle is larger than the length of the third side.
• The total length of two edges gets smaller and smaller as the vertex which is the connecting point of the two sides, is pulled down towards the third side. The sum of these two lengths becomes equal to the length of the third side when the vertex containing the two sides falls onto the third side - but then the triangle no longer remains a triangle, it becomes a straight line.

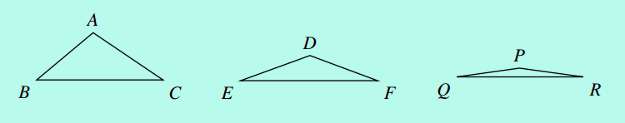
In the third triangle $\triangle PQR$ the vertex $P$ has nearly fallen onto the base $QR$, but still the sum of the two smaller sides $PQ$ and $PR$ is larger than the longer side $QR$. Thus the longest side $QR$ can never be larger than the total length of other two smaller sides in a triangle.

External angle equal to two opposite internal angles:

In any triangle, an external angle is equal to the sum of two of its opposite angles.

$\angle \alpha$ is an external angle at $B$. Its opposite angles inside the triangle are $\angle \beta$ and $\angle \gamma$. So we have the following relationship,

$ \alpha = \beta + \gamma$

This relationship holds because,

$ \alpha + \angle ABC = 180^0 = \beta + \gamma + \angle ABC \qquad \text{and so, } \alpha = \beta + \gamma $.

Types of triangles

Broadly triangles can be categorized into four types,

Right angled triangle:

This is one of the most important geometric shapes, as apart from other special uses, the topic of Trigonometry is based on this triangle. In this type of triangle,

One of the three angles is $90^0$

and consequently, the sum of the other two angles is also $90^0$.

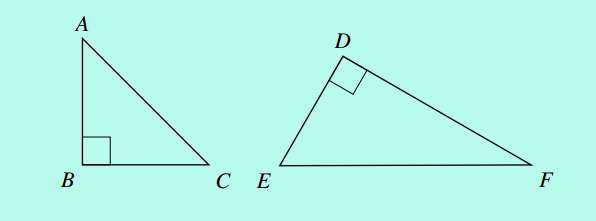
In a right triangle, all three sides may be unequal. The only condition is: one of the three angles must be a right angle.

Both $\triangle ABC$ and $\triangle EDF$ are right triangles with all three sides unequal.

Pythagoras theorem:

This is one of the most important and basic theorems in Geometry establishing a definite relationship between the length of the sides of a right triangle.

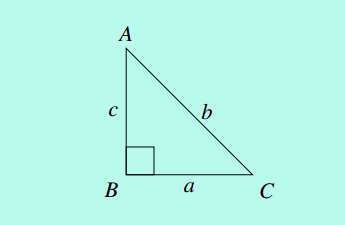
In $\triangle ABC$ the $\angle ABC$ is the right angle, the side $BC=a$ is the $Base$, the side $AB=c$ is the $Height$ and the side $AC=b$ is the $hypotenuse$.

By Pythagoras Theorem,

$ (Base)^2 + (Height)^2 = (Hypotenuse)^2 \qquad \textit{or, } \quad a^2 + c^2 = b^2 $

In the right triangle $\triangle EDF, Base$ is $DE$, $Height$ is $DF$ and $Hypotenuse$ is $FE$.

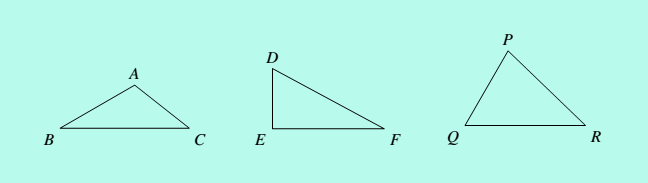
General triangle with all sides unequal - Scalene triangle:

In a scalene triangle there is no special characteristic of the sides. It has all its sides of different lengths and all its angles of different degrees.

No two sides are of same lengths and no two angles are same.

With all three sides unequal and all three angles unequal as well, even a right-angled triangle may be a scalene triangle. An example is the $\triangle DEF$ out of the three scalene triangles shown below.

Isosceles triangle:

This is the type of triangle where,

Two of its sides are of equal lengths. The two angles adjacent to the equal sides are also equal.

A perpendicular from the vertex $A$ containing the equal sides on to the unequal side $BC$ bisects the unequal side, the vertex angle $\angle A$ and the area of the triangle $\triangle ABC$ as shown below.

The only other conclusion that can be made from these two basic properties of an isosceles triangle is,

The third unequal angle must be of even degrees.

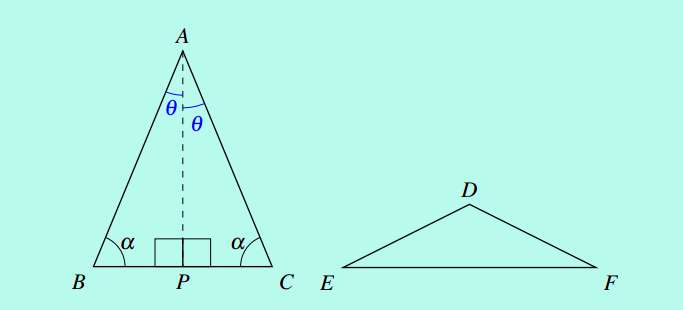
Reason: why the Base and the Vertex angle are bisected and why the two angles adjacent to the equal sides are equal:

By Pythagoras theorem,

$ AB^2 = PB^2 + PA^2 = AC^2 = PA^2 + PC^2$

and so, $PB^2 = PC^2 \qquad \text{or, } PB=PC $

Thus, in the two triangles $\triangle APB$ and $\triangle APC$ all corresponding sides are equal. This makes the two triangles Congruent and consequently all corresponding angles of the two triangles are equal. Thus,

$ \angle ABP = \angle ACP = \alpha \qquad \text{and, } \angle BAP = \angle CAP = \theta $

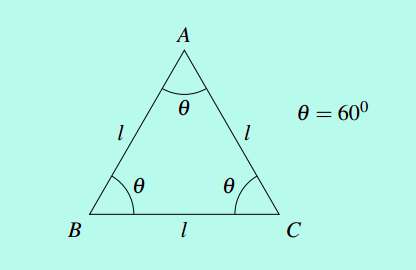
Equilateral triangle:

In an equilateral triangle $\triangle ABC$ all the three sides are equal. Consequently all the three angles are also equal and of value $60^0$.

Similar to the isosceles triangle, in an equilateral angle also the perpendicular from the vertex $A$ onto the base $BC$ bisects both the $\angle A$ and the base $BC$.

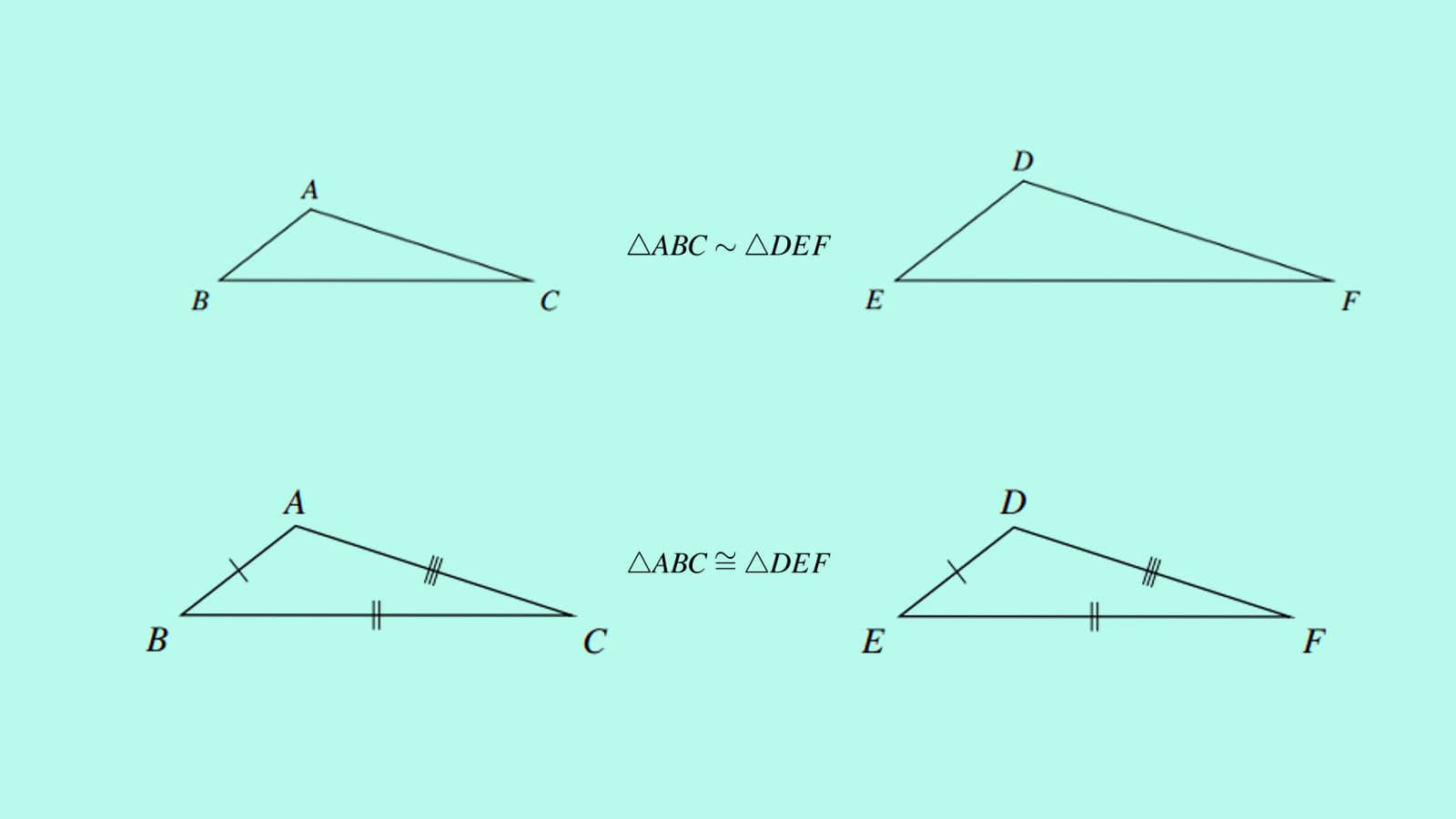
Comparison between two triangles

Two triangles can be related to each other in two special ways: Similar and Congruent.

Similar triangles:

In two similar triangles,

• Three angles of one triangle $\triangle ABC$ are same as the three corresponding angles of the second triangle $\triangle DEF$. That is, $\angle A=\angle D$, $\angle B=\angle E$, and $\angle C=\angle F$, and,
• Ratio of corresponding sides of the two triangles are also same. That is, $ \displaystyle\frac{AB}{DE}=\displaystyle\frac{BC}{EF}=\displaystyle\frac{CA}{FD}$.

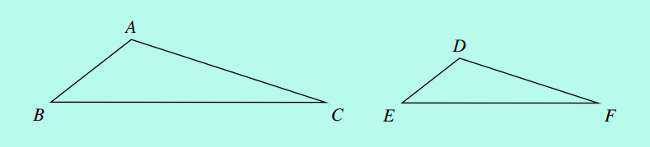
The $\triangle ABC$ and $\triangle DEF$ are similar. If you look at them and compare you can perceive the similarity. In more concrete terms, all three pairs of corresponding angles are equal: $\angle A=\angle D$, $\angle B=\angle E$ and $\angle C=\angle F$. The first condition is thus satisfied.

Also, the ratios of corresponding sides are equal:

$ \displaystyle\frac{AB}{DE}=\displaystyle\frac{BC}{EF}=\displaystyle\frac{CA}{FD}=1.4 $

It is as if the smaller triangle has been made larger without changing the aspect ratio, which is a familiar term in dealing with bit-mapped pictures. Unless the angles were same, this could not have been achieved.

Any of these triangles could have been rotated by any angle, but the similarity won't have been disturbed.

If the ratio of corresponding sides equals 1, we get the triangles exactly equivalent or Congruent.

Congruent triangles:

This is a special case of similar triangles where corresponding sides are equal, or in other words, the ratio of corresponding sides equal 1.

Additionally because of similarity the corresponding angles are also equal. Effectively one triangle is duplicate of the other.

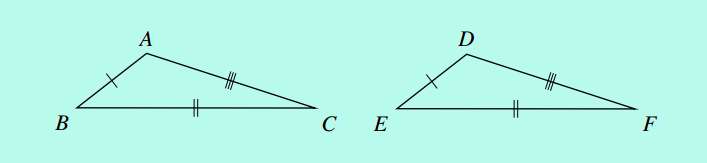
Testing if two triangles are similar:

There are three tests. If two triangles pass in any of these tests those are similar to each other.

1. AA: or Angle-Angle : If two pairs of corresponding angles of two triangles are equal, the triangles must be similar. Equality of two pairs of corresponding angles results in equality of the third pair of corresponding angles also and the test can be termed as AAA similarity.

2. SSS: or Side-Side-Side : If ratios of three pairs of corresponding sides are equal, the two triangles are similar.

3. SAS: or Side-Angle-Side : If two corresponding sides are in same ratio and the included angles are equal the two triangles are then similar. Corresponding sides AB, DE and AC, DF are in equal ratio and included angles $\angle A = \angle D$.

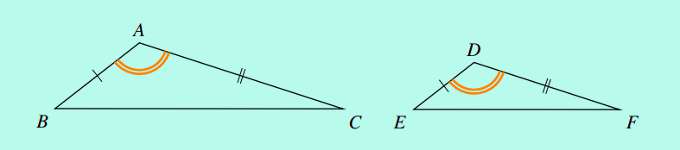
Reason: As the included angle is same as well as the ratio of the two sides of the included angle, the smaller triangle can be superimposed on the larger triangle in such a way that the vertices at the equal angles are superimposed on each other in a coincident manner and the two including sides of the smaller triangle would also lie along the two including sides of the larger triangle.

The situation would be as if two triangles $\triangle ABC$ and $\triangle DEF$ are superimposed with $\angle D$ merged with common $\angle A$ and ratio of including sides equal, $AB : AE = AC : AF$.

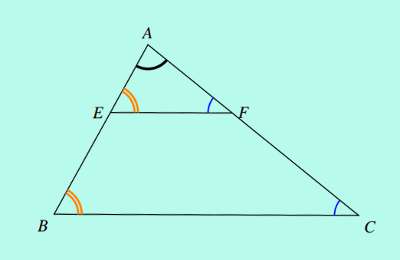
After superimposition, the vertex angle $\angle A$ will be same (with merged $\angle D$) and ratio of sides AB and AE will be equal to ratio of sides AC and AF. This will automatically make EF parallel to BC.

The two triangles $\triangle ABC$ and $\triangle DEF$ will then be similar as $EF || BC$. Consequently, in the two triangles $\angle A=\angle A$, $\angle B= \angle E$, and $\angle C=\angle F$. Furthermore the ratio of the corresponding sides will also be equal,

$ \displaystyle\frac{AB}{AE}=\displaystyle\frac{AC}{AF}=\displaystyle\frac{BC}{EF} $.

Testing for congruency:

If two triangles pass any of the following tests, those are congruent.

1. SSS test: If three sides are equal, the triangles are congruent. It is obvious.

2. SAS test: If two sides are equal as well as the included angle, the triangles are congruent.

This follows from the SAS similarity test.

3. ASA test: If two angles are equal as well as the side included between them the triangles are congruent.

If two angles are equal, the third angle would also be equal and the triangles would be similar with the corresponding sides in a fixed ratio. As two corresponding sides are equal (with ratio 1), the other two pairs of corresponding sides would also be equal (with ratio 1).

By this reasoning, a fourth congruency test becomes feasible.

4. AAS test: If two angles are equal and any other adjacent side, the two triangles are congruent.

5. RHS test: This is a special cases of right triangle. If hypotenuse and any other side in a pair of right triangles are equal, the triangles are congruent.

Reason: By Pythagoras theorem, the third side will also be equal and it will be transformed into SSS test.

Special points in a triangle

A triangle has a few important special points in it.

Intersection of perpendicular bisectors of three sides of a triangle - the Circumcenter

The perpendicular bisectors of the three sides of a triangle meet at a single point, the Circumcenter of the triangle.

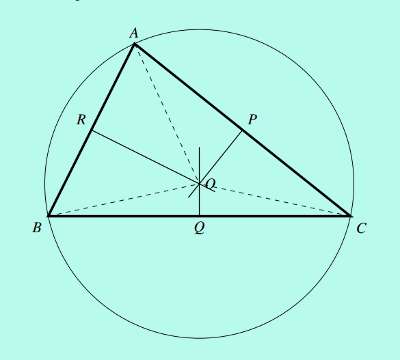
The Circle on which the three vertices of the triangle lie is the Circumcircle of the triangle; the radius of the circle - any of the lengths of AO, BO or CO is the Circumradius of the triangle and center point $O$ is the Circumcenter of the triangle.

As the distance of the circumcenter from the three vertices are same, the circumceter may also be defined as,

The point equidistant from the three vertices of a triangle.

Method of getting the Circumcenter, and Circumcircle:

• Draw three perpendicular bisectors on the three sides of the triangle.

• Discover that the three perpendicular bisectors will always meet at a point inside the triangle. This is the Circumcenter of the triangle.

• Join the vertices $A$, $B$ and $C$ with this new point $O$. The lengths of $AO$, $BO$ and $CO$ will be equal.

• Draw a circle with center at $O$ and radius as any of three $AO$, $BO$ or $CO$

Reason:

The sides of the triangle are three chords of the circumcircle, and a perpendicular from the center of a circle to a chord always bisects the chord. Thus the perpendicular bisectors of the three sides of the triangle $\triangle ABC$ will meet at the center of a circle on which the vertices of the triangle lie.

In triangle $\triangle BOC$, $OQ$ is the perpendicular to $BC$. As $OB=OC$, being the radii, and common side being $OQ$, in the two right triangles, $\triangle BOQ$ and $\triangle COQ$ the third sides are also same by Pythagoras theorem, that is, $BQ=QC$.

So, the perpendicular from the centre of a circle to a chord bisects the chord.

Intersection of perpendiculars from vertices to opposite sides - the Orthocenter:

The perpendiculars from the three vertices to the opposite sides meet at a point which is the Orthocenter of the triangle.

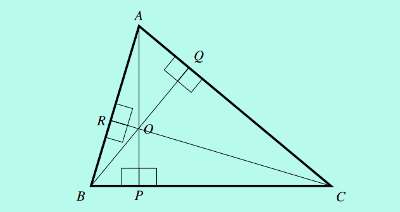
• In an acute angled triangle, as in the example, the Orthocenter lies inside the triangle.
• In an obtuse angled triangle, the orthocenter lies outside the triangle, and
• in a right triangle the orthocenter is coincident with the right angle vertex.

The following is an example of orthocenter in an obtuse angled triangle.

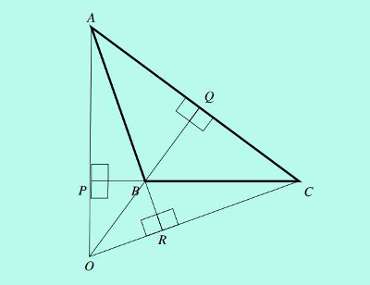
$AP$ is the height of the triangle on base side $CB$ extended, $BQ$ is the height on base side $AC$ and $CR$ is the height on the base $AB$ extended.

These three heights or altitudes do not meet inside the triangle. These meet at $O$ outside the triangle after extending through the obtuse angle $\angle B$ of vertex $B$.

Intersection of medians - the Centroid

A median is the line connecting a vertex with the mid-point of the opposite side.

The three medians of a triangle meet at a single point Centroid.

This is called the centre of gravity of a triangle.

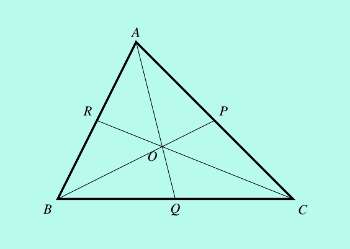
The centroid divides each median into a ratio of 2:1.

As a result we have,

$ \displaystyle\frac{AO}{OQ}=\displaystyle\frac{BO}{OP}=\displaystyle\frac{CO}{OR}=2 $.

Intersection of angle bisectors - the Incenter:

The three internal angle bisectors meet at the point named as incenter. This is the center of a circle inscribed inside the triangle. The three sides of the triangle are tangents to the circle.

The angle bisectors $AI$, $BI$ and $CI$ bisect the angles $\angle A$, $\angle B$ and $\angle C$ respectively and meet at the point $I$ which is the centre of the inscribed circle of radius $IP$ or $IR$. This is the incenter of the triangle $\triangle ABC$.

As the radius of a circle is perpendicular to a tangent, when two tangents $BC$ and $AC$ meet at $C$, $IP$ and $IQ$ are perpendiculars on $CB$ and $CA$ and also are equal, being the radii of the inscribed circle.

Thus, the two triangles $\triangle ICQ\equiv\triangle ICP$. This results in $\angle ICQ=\angle ICP$, that is, $CI$ is the bisector of the angle $\angle C$.

By the same logic, $BI$ and $AI$ are the bisectors of the angles $\angle B$ and $\angle A$.

Thus the center of the inscribed circle of a triangle, the incenter, is the meeting point of its three angle bisectors.

We will know more about triangles and about new shapes quadrangles, polygons and circles in,

Geometry basic concepts part 2 on quadrilaterals polygons squares

Geometry basic concepts part 3 on Circles.

Let us end the session with a problem exercise set for you.

---

Problem exercise

The recommended time limit is 18 minutes

Problem 1.

$\triangle ABC$ is an isosceles triangle with $AB=AC$ and $AD$ as the median to base $BC$. If $\angle ABC = 35^0$, the $\angle BAD$ is

1. $70^0$
2. $35^0$
3. $55^0$
4. $110^0$

Problem 2.

In isosceles triangle $\triangle FGH$,  $FG \lt 3$ cm and $GH = 8$cm. Then the correct relation is,

1. $GH = FH$
2. $GH \lt FH$
3. $GF = GH$
4. $FH \gt GH$

Problem 3.

The sum of three altitudes of a triangle is,

1. equal to the sum of three sides
2. twice the sum of sides
3. greater than the sum of sides
4. less than the sum of sides

Problem 4.

The length of 3 sides of a triangle are, 6cm, 8cm and 10cm. The length of the median to the greatest side is then,

1. 5cm
2. 8cm
3. 4.8cm
4. 6cm

Problem 5.

$O$ and $C$ are the Orthocenter and the Circumcenter of an acute angled triangle $\triangle PQR$ respectively. The points $P$ and $O$ are joined and produced to meet the side $QR$ at $S$. If $\angle QCR = 130^0$ and $\angle PQS = 60^0$ then $\angle RPS$ is,

1. $100^0$
2. $35^0$
3. $30^0$
4. $60^0$

Problem 6.

If $I$ is the incenter of $\triangle ABC$, $\angle ABC = 65^0$ and $\angle ACB = 55^0$, the $\angle BIC$ is,

1. $110^0$
2. $120^0$
3. $130^0$
4. $140^0$

Problem 7.

If the median drawn on the base of a triangle is half its base, the triangle will be,

1. acute-angled
2. obtuse-angled
3. right-angled
4. equilateral

Problem 8.

In a right angled triangle the product of its two sides equals half of the square of the third side which is the hypotenuse. One of the acute angles must then be,

1. $15^0$
2. $30^0$
3. $45^0$
4. $60^0$

Problem 9.

In $\triangle ABC$, two points $D$ and $E$ are taken on the lines $AB$ and $BC$ respectively in such a way that $AC$ is parallel to $DE$. The $\triangle ABC$ and $\triangle DBE$ are then,

1. always similar
2. alway conguent
3. similar only if $D$ lies outside the line segment $AB$
4. congruent only if $D$ lies outside the line segment $AB$

Problem 10.

$AD$ is a median of $\triangle ABC$ and $O$ is the centroid such that $AO = 10cm$. Length of $OD$ (in cm) is,

1. 7
2. 5
3. 4
4. 2

Answers to the exercise problems

Problem 1: c: $55^0$.

Problem 2: a: $GH=FH$.

Problem 3: d: less than the sum of sides.

Problem 4: a: 5cm.

Problem 5: b: $35^0$.

Problem 6: b: $120^0$.

Problem 7: c: right-angled.

Problem 8: c: $45^0$.

Problem 9: a: always similar.

Problem 10: b: 5.

---

These questions form the SSC CGL level Question set 18 on Geometry 1.

Detailed solutions are in SSC CGL level Solution set 18 on Geometry 1.

---

Guided help on Geometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,

Suresolv Geometry Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.

---

→