A challenging problem needing as many as five rich Geometry concepts for systematic solution
The problem we are going to solve now was reported in The Sydney Morning Herald as the hardest one in the recent Math Extension 2 exam paper in NSW HSW 2019 test in Australia.
It turned out to be not so difficult, but what interested us is the rich partially hidden possibilities leading to the systematic solution. What made the problem more popular perhaps is its balance—it is not too difficult so that only the math specialists can solve it. Any brave student (yes, to solve math problems you can't afford to have fears) knowing the basic and rich Geometry concepts taught in schools can solve it without much difficulty.
Our recommendation: Before going through the solution, make a good attempt to solve it yourself. If you do that, you would surely learn a lot more when you go through the solutions.
The Problem—Question 16c in NSW HSC Math Extension 2 exam in 2019
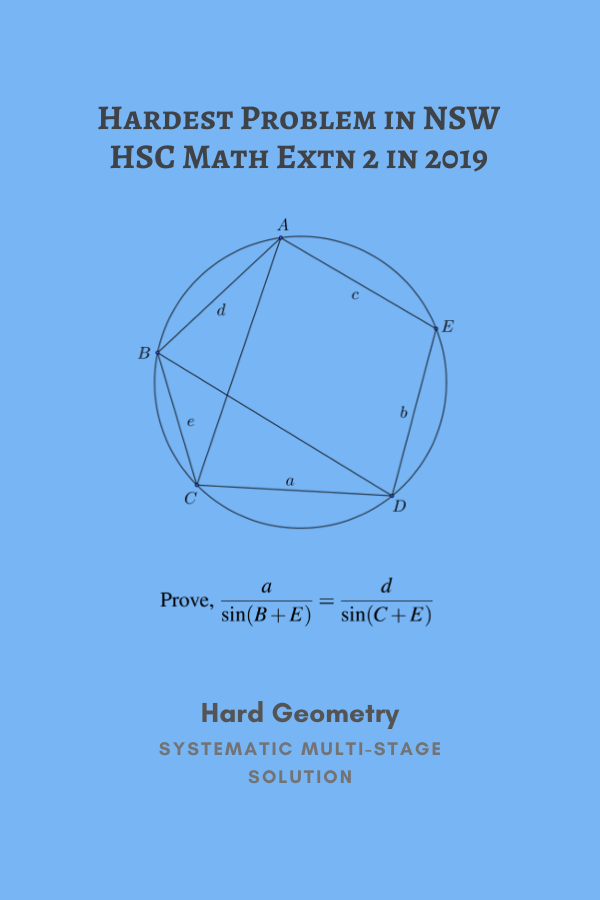
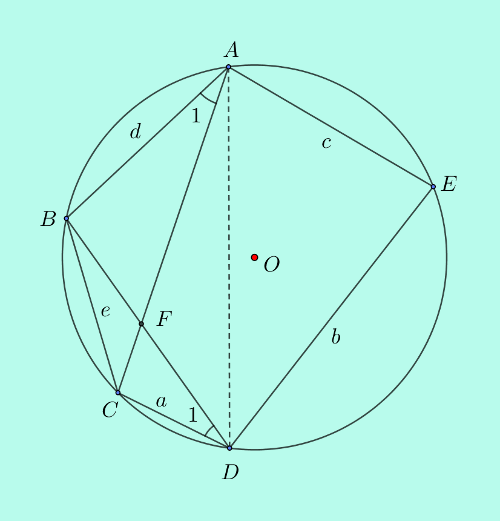
The vertices $A$, $B$, $C$, $D$ and $E$ of a pentagon lie on a circle. The lengths of the sides opposite $A$, $B$, $C$, $D$ and $E$ are $a$, $b$, $c$, $d$ and $e$, as shown.

Using the same convention as in the sine rule, the vertex name also represents the size of the angle at that vertex. For example $A$ is used to represent the size of $\angle BAE$.
Show that, $\displaystyle\frac{a}{\sin (B+E)}=\frac{d}{\sin(C+E)}$.
Systematic solution to Question 16c in NSW HSC Math Extension 2 exam in 2019
You are gently nudged in the question itself to use the important Law of sines in Geometry and Trigonometry.
Solution Stage 1: Problem analysis and strategy decision to establish connection between $a$ and $d$ through an intermediate link element
In the definition of law of sines, for a $\triangle ABC$ with vertices $A$, $B$ and $C$, the opposite side lengths are named as, $a$ opposite to $\angle A$ at vertex $A$, $b$ opposite to $\angle B$ at vertex $B$, and $c$ opposite to $\angle C$ at vertex $C$.
The law of sines establishes the relation between the side lengths and angles as,
$\displaystyle\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C}$
To know how the law of sines formula is formed (mechanism and proof), you may go through our concept article,
Basic and rich Geometry concepts part 7, laws of sines and cosines.
The target relation that we have to prove is in the form of the ratios in law of sines as,
$\displaystyle\frac{a}{\sin (B+E)}=\frac{d}{\sin(C+E)}$ ...... (1)
But the pairs of side lengths and the angles do not belong to the same triangle and the angles also are not the angles opposite to the sides.
It is clear that we have to use the law of sines, but,
We need to establish connections between $a$ and $d$ first using an intermediate side element common to the two triangles to which $a$ and $d$ belong.
This innovative strategy we have used often to solve many of the difficult problems in Geometry or even in other topics in abstraction. We call this as Link element use technique.
Solution Stage 2: Connection established between two numerators $a$ and $d$ using intermediate side element $e$ linking the two
Let us show you the problem figure again for convenience of understanding.
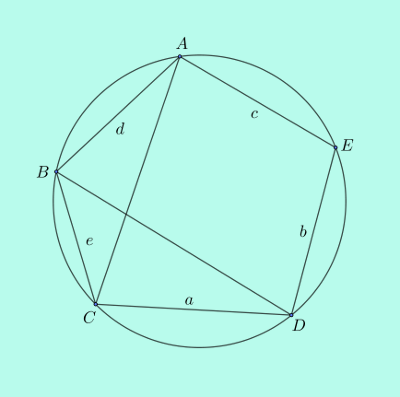
First the relation between $a$ and $e$ using law of sines in the $\triangle BCD$ is confirmed as,
$\displaystyle\frac{a}{\sin \angle CBD}=\frac{e}{\sin \angle BDC}$ ...... (2)
And next in $\triangle ABC$, the law of sines is again applied to confirm the relation between $e$ and $d$ now as,
$\displaystyle\frac{e}{\sin \angle BAC}=\frac{d}{\sin \angle ACB}$ ...... (3)
You have formed two equations of a pair of ratios satisfying Law of sines in which $e$ in numerator is common. But the angles associated $e$ are different. If only these two angles were equal we would have been able to relate $a$ and $d$ in two sine ratios forming a single ratio equation.
Solution Stage 3: Moving one step more towards solution by applying arc angle subtending property
Now we will use the problem figure with a little more information as below.
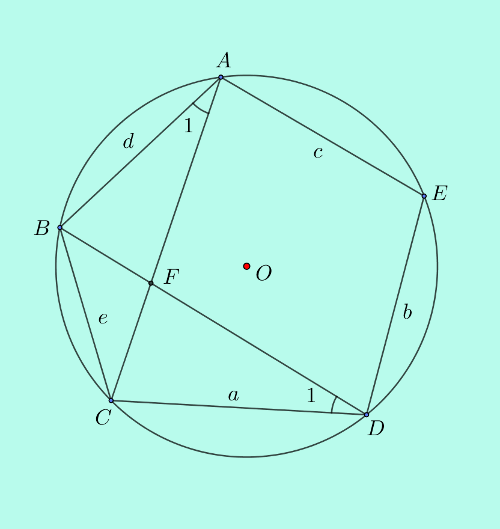
The arc angle subtending property says,
An arc in a circle subtends the same angle at every point on its complementary arc (whole circle periphery excluding the arc).
Observe that the arc (not the chord) $BC$ subtends $\angle BAC$ and $\angle BDC$ both on the complementary arc and so by this powerful property,
$\angle BAC=\angle BDC$.
If you want to know the mechanism of how this property is considered true (or in simple words—its proof), you may refer to our concept article,
Basic and rich Geometry concepts part 4, proof of arc angle subtending property.
In the new problem figure above the two equal angles are labelled simply as $\angle 1$.
Because of this equality of the two angles in the denominators in the RHS of equation (2) and LHS of equation (3), you have finally an equality of two ratios that is nearly same as the equality to be proved as in equation (1), except that the angles are different (but the numerators are same $a$ and $d$ as to be proved). This favorable relation formed is,
$\displaystyle\frac{a}{\sin \angle CBD}=\frac{e}{\sin \angle BDC}=\displaystyle\frac{e}{\sin \angle BAC}=\frac{d}{\sin \angle ACB}$
Or, $\displaystyle\frac{a}{\sin \angle CBD}=\frac{d}{\sin \angle ACB}$ ...... (4)
Interchange the numerator of RHS and denominator of LHS and have a more favorable relation as,
$\displaystyle\frac{a}{d}=\frac{\sin \angle CBD}{\sin \angle ACB}$ ...... (5).
Now you only have to prove,
$\displaystyle\frac{\sin \angle CBD}{\sin \angle ACB}=\frac{\sin (B+E)}{\sin (C+E)}$.
You have transformed the original problem to a simpler problem in which you only have to think about angles.
Solution Penultimate Stage 4: Applying opposite angle equality property of a cyclic quadrilateral
By the property of a cyclic quadrilateral,
Sum of each pair of opposite angles in a cyclic quadrilateral inscribed in a circle is $180^0$.
To know why this property of cyclic quadrilateral is true, you may refer to our concept article at,
Geometry—Basic and rich concepts part 3 on circles.
By this property in the figure above, first,
$\angle DBA +\angle E=180^0$,
Or, $\angle E = 180^0-\angle DBA$.
But, $\angle B=\angle DBA +\angle CBD$.
Adding the two,
$\angle B+\angle E=180^0+\angle CBD$ ...... (6)
Applying the property of cyclic quadrilateral again on $\angle E$ and $\angle DCA$, sum of the two is also $180^0$,
$\angle E+\angle DCA=180^0$.
Or, $\angle E=180^0-\angle DCA$.
But, $\angle C=\angle DCA +\angle ACB$.
Add the two to eliminate $\angle DCA$,
$\angle C +\angle E=180^0+\angle ACB$ ...... (7)
Take the ratio of sines of both sides of the two equations (6) and (7),
$\displaystyle\frac{\sin (B+E)}{\sin (C+E)}=\frac{\sin (180^0+\angle CBD)}{\sin (180^0+\angle ACB)}$ ...... (8).
Solution Final stage: solution by applying third quadrant sine angle transformations
Notice how the problem is transformed stage by stage in a reasoned and analyzed systematic path towards the solution.
From the sinusoidal curve of $y=\sin \theta$ against variations of $\theta$, it would be clear how $\sin \theta$ and $\sin (180^0+\theta)$ are related (where $\theta$ is acute) in the third quadrant ($180^0 \lt \theta \lt 270^0$).
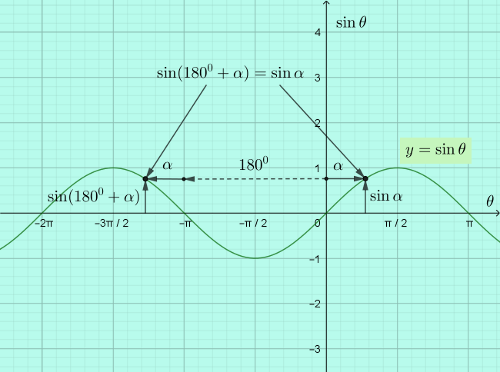
In the third quadrant, for any acute $\angle \alpha$,
$\sin (180^0+\alpha)=\sin \alpha$.
Both $\angle CBD$ and $\angle ACB$ being acute, apply this relation on the sine functions on the RHS of equation 8 to get,
$\sin (180^0+\angle CBD)=\sin \angle CBD$, and,
$\sin (180^0+\angle ACB)=\sin \angle ACB$.
This simplified relation results finally in,
$\displaystyle\frac{\sin (B+E)}{\sin (C+E)}=\frac{\sin \angle CBD}{\sin \angle ACB}=\frac{a}{d}$,
Or, $\displaystyle\frac{a}{\sin (B+E)}=\frac{d}{\sin (C+E)}$.
Proved.
Summary of analytical reasoning for reaching the solution mentally
From the relation to be proved it was clear that law of sines has to be applied.
So applying Law of sines on the two triangles $\triangle CBD$ and $\triangle ACB$, two equality ratios in sides $a$, linking side $e$ and side $d$ are obtained with common side $e$ clearly playing the role of linking element.
The side $e$ is the chord of arc $BC$ subtending equal angles $\angle BAC$ and $\angle BDC$ on the complementary arc and these are the angles opposite to side $e$ in the two triangles.
This results in the first success in establishing ratio equality relation similar to the target relation,
$\displaystyle\frac{a}{\sin \angle CBD}=\frac{d}{\sin \angle ACB}$.
It now remained to prove that,
$\displaystyle\frac{\sin (B+E)}{\sin (C+E)}=\frac{\sin \angle CBD}{\sin \angle ACB}$.
Using property of sum of opposite angles of a cyclic quadrilateral, this equality ratio with an additional $180^0$ could be obtained easily as,
$\displaystyle\frac{\sin (B+E)}{\sin (C+E)}=\frac{\sin (180^0+\angle CBD)}{\sin (180^0+\angle ACB)}$.
Both $\angle CBD$ and $\angle ACB$ being acute, the angles on the RHS fall in the third quadrant and so their sines become equal to sines of each plus $180^0$.
General form of the problem
In the given problem, $\angle E$ is obtuse. In a general form of the problem, $\angle E$ may be $90^0$ or even be acute. What would be the nature of $\angle CBD$ and $\angle ACB$ in these two situations? Would these angles still remain acute so that the third quadrant compound angle sine identities hold?
If $\angle E$ is $90^0$, both the opposite angles $\angle DBA$ and $\angle DCA$ have to be $90^0$ also. It follows then the angles $\angle CBD$ and $\angle ACB$ will be acute because in a circle, inscribed angle $\angle B$ or $\angle C$ must be less than $180^0$.
The following graphic shows such a problem situation.

In this first variation, $\angle E$ is $90^0$ and opposite angles, $\angle DBA$ and $\angle DCA$ are both $90^0$. So the two angles $\angle CBD$ and $\angle ACB$ remain acute satisfying the third quadrant compound angle sine function relations.
Again if $\angle E$ is acute, both the opposite angles $\angle DBA$ and $\angle DCA$ must be obtuse (their sum with $\angle E$ to be $180^0$). In this case also then both the angles $\angle CBD$ and $\angle ACB$ must be acute.
The following graphic shows such a problem situation.
In this second variation, $\angle E$ is acute and opposite angles, $\angle DBA$ and $\angle DCA$ are obtuse. So the two angles $\angle CBD$ and $\angle ACB$ remain acute satisfying the third quadrant compound angle sine function relations.
In general, the relation $\displaystyle\frac{a}{\sin (B+E)}=\frac{d}{\sin (C+E)}$ in the given problem will be true.
End note
We classify this as a problem with especially rich potential of learning because as many as five rich concepts were needed to solve the problem step by step systematically.
If the problem solver is at ease with the concepts, it doesn't take much time to reach the solution, though it might take a bit of time to write down all the required steps of the solution.
Overall, this systematic problem solving approach ensures assured and confident solution in steps.