SSC CGL Tier II level Solution Set 14, Algebra 5

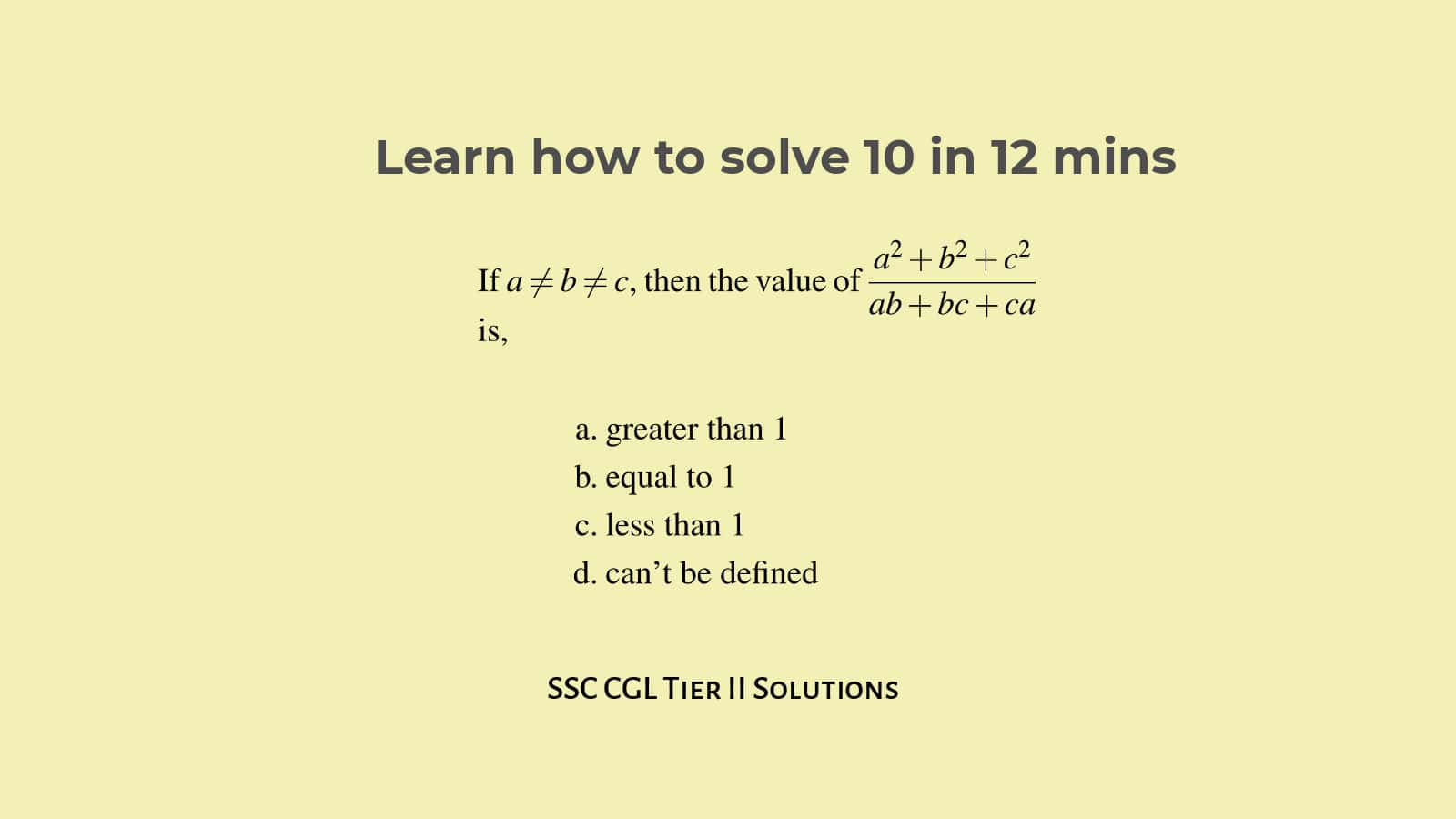
Elegant quick solutions to algebra questions for SSC CGL Tier 2 Set 14

Go through the algebra solutions for SSC CGL Tier 2 Set 14 to learn how the 10 not so easy questions can be solved in scheduled 12 minutes' time.

To solve such problems quickly, identification of inherent patterns and use of associated methods are necessary. The solution set encapsulates the approach.

For best results take the test first at,

SSC CGL Tier II level question set 14 on Algebra 5.

Taking the test will enable you to appreciate the pattern based solution process better.

---

Algebra solutions for SSC CGL tier 2 question Set 14 - answering time was 15 mins

Q1. If $x=\displaystyle\frac{\sqrt{13}+\sqrt{11}}{\sqrt{13}-\sqrt{11}}$, and $y=\displaystyle\frac{1}{x}$, then the value of $3x^2-5xy+3y^2$ is,

1. 1771
2. 1717
3. 1177
4. 1171

Solution 1: Problem analysis and first stage of execution: Solving in mind

As $y$ is inverse of $x$, and in the target expression the coefficients of $x^2$ and $y^2$ are equal with middle term effectively numeric, the expression can easily be transformed to a sum of squares of inverses. This is the identification technique for the pattern of sum of inverses.

Thus the target expression,

$3x^2-5xy+3y^2$

$=3\left(x^2+\displaystyle\frac{1}{x^2}\right)-5$.

This can be quickly evaluated by applying principle of interaction of inverses if we are able to transform the given expression in $x$ as a sum of inverses in $x$.

On the way to achieve this objective our first target would be to eliminate the square roots.

The given value of $x$ is componendo dividendo ready (pattern identification again), and so we will first carry out the three-step method in mind to get,

$\displaystyle\frac{x+1}{x-1}=\frac{\sqrt{13}}{\sqrt{11}}$.

Next step is obvious. We need to raise both sides of the equation to the power 2 to get rid of the square roots,

$\displaystyle\frac{(x+1)^2}{(x-1)^2}=\frac{13}{11}$.

Applying componendo dividendo again,

$\displaystyle\frac{x^2+1}{2x}=12$, it helped quick simplification this time,

Or, $x+\displaystyle\frac{1}{x}=24$.

It has been possible to arrive at this point mentally without writing any step. Our objectives that we set are met. Only one step remains.

Solution 1: Second stage of execution

The target expression is already in suitable form to apply principle of interaction of inverses,

$3x^2-5xy+3y^2$

$=3\left(x^2+\displaystyle\frac{1}{x^2}\right)-5$,

$=3\left[\left(x+\displaystyle\frac{1}{x}\right)^2-2\right]-5$

$=3(24^2-2)-5$, by the use of principle of interaction of inverses,

$=3\times{574}-5=1722-5=1717$.

Answer: Option b: 1717.

Problem analysis, effective strategy decision and pattern based power methods enable us to reach the solution wholly in mind.

Key concepts used: Problem analysis -- Key pattern identification -- Pattern identification technique -- Strategy decision -- Repeated componendo dividendo -- End state analysis approach -- input transformation -- Principle of interaction of inverses.

Q2. If $x=7+4\sqrt{3}$ find the value of $\displaystyle\frac{3x^6+2x^4+4x^3+2x^2+3}{x^4+x^3+x^2}$ is,

1. $\displaystyle\frac{8238}{15}$
2. $\displaystyle\frac{8138}{15}$
3. $\displaystyle\frac{8338}{15}$
4. $\displaystyle\frac{8138}{17}$

Solution 2: Problem analysis and key pattern identification: Solving in mind

At first look the target expression seemed awkward and troubling, but the pattern in the given expression could be identified immediately,

$x=7+4\sqrt{3}=\displaystyle\frac{1}{7-4\sqrt{3}}$,

as, $\left[7^2-(4\sqrt{3})^2\right]=1$,

Or, $(7+4\sqrt{3})(7-4\sqrt{3})=1$.

So, $x+\displaystyle\frac{1}{x}=(7+4\sqrt{3})+(7-4\sqrt{3})=14$

This is easy and done in mind. Best of all we got an expression in $x$ on which we can apply the powerful Principle of interaction of inverses.

Our next job will be to convert the target denominator and numerator in terms of this relation and replace each such factor by the value of 14.

Solution 2: Problem solving: Identifying the sum of inverse expression pattern by equal coefficient pairs: Pattern identification technique

To identify which pair of terms can be converted to $\left(x+\displaystyle\frac{1}{x}\right)$ form, we look for two terms with equal coefficient values as well as the difference between the power of $x$ in two terms of even value.

Taking the denominator and applying this pattern identification technique,

$x^4+x^3+x^2=x^3\left(x+1+\displaystyle\frac{1}{x}\right)=15x^3$.

This is easy and again solvable in mind.

Taking the numerator, first and fifth terms are paired as well as the second and the fourth,

$3x^6+2x^4+4x^3+2x^2+3$

$=3x^3\left(x^3+\displaystyle\frac{1}{x^3}\right)+2x^3\left(x+\displaystyle\frac{1}{x}\right)+4x^3$

$=3x^3\left[\left(x+\displaystyle\frac{1}{x}\right)^3-3\left(x+\displaystyle\frac{1}{x}\right)\right]+32x^3$, as $(a^3+b^3)=(a+b)^3-3ab(a+b)$,

$=3x^3(14^3-42)+32x^3$

$=8138x^3$.

As we already have the denominator evaluated to, $15x^3$, the answer would be,

$\displaystyle\frac{8138}{15}$.

Answer: Option b : $\displaystyle\frac{8138}{15}$.

Key concepts used: Key Pattern identification -- Pattern identification technique -- Principle of interaction of inverses -- Pattern of sum of inverses -- Target expression driven input transformation -- End state analysis approach.

Though we needed to write only the last calculative step of,

$=3x^3\left[\left(x+\displaystyle\frac{1}{x}\right)^3-3\left(x+\displaystyle\frac{1}{x}\right)\right]+32x^3$,

careful by hand calculations and overall reasoning resulted in solving time just about a minute and half. This is not a 30 second problem for us.

Q3. The value of the expression $\displaystyle\frac{(x^2-y^2)^3+(y^2-z^2)^3+(z^2-x^2)^3}{(x-y)^3+(y-z)^3+(z-x)^3}$  is,

1. $(x^2-y^2)(y^2-z^2)(z^2-x^2)$
2. $3(x+y)(y+z)(z+x)$
3. $(x+y)(y+z)(z+x)$
4. $3(x-y)(y-z)(z-x)$

Solution 3: Problem analysis, key pattern identification and solving in mind

Though the target expression is large and imposing, both its numerator and denominator have the simplifying pattern of $(a+b+c)=0$, where for the numerator, $a=(x^2-y^2)$, $b=(y^2-z^2)$, $c=(z^2-x^2)$ and for the denominator, $a=(x-y)$, $b=(y-z)$ and $c=(z-x)$.

And we also know from our rich algebraic concepts repertoire, if $a+b+c=0$, then $a^3+b^3+c^3=3abc$.

Replacing the sums of cubes by products of factors and cancelling out common factors, the solution, $(x+y)(y+z)(z+x)$, is reached in a few tens of seconds.

Answer: Option c: $(x+y)(y+z)(z+x)$.

Key concepts used: Key pattern identification -- Sum of cubes rich algebraic concept.

Derivation of Sums of cubes rich algebraic concept

$a+b+c=0$,

Or, $(a+b)^3=-c^3$,

Or, $a^3+b^3+c^3=-3ab(a+b)=3abc$, as $(a+b)=-c$.

Q4. If $a+b+c=5$, $ab+bc+ca=7$ and $abc=3$ find the value of $\left(\displaystyle\frac{a}{b}+\displaystyle\frac{b}{a}\right)+\left(\displaystyle\frac{b}{c}+\displaystyle\frac{c}{b}\right)+\left(\displaystyle\frac{c}{a}+\displaystyle\frac{a}{c}\right)$.

1. $9\displaystyle\frac{2}{3}$
2. $8\displaystyle\frac{2}{3}$
3. $8\displaystyle\frac{1}{3}$
4. $7\displaystyle\frac{2}{3}$

Solution 4: Problem analysis, pattern identification and Strategy decision

We could see that if product of the first two algebraic expressions in the LHSs is divided by $abc$, it will consist of 9 terms in the numerator out of which three terms will combine to form,

$3abc$,

and the rest 6 terms should (we are not fully sure but we expect) result in something like the target expression by their nature, when divided by $abc$.

This is the key pattern we identify by mental assessment of the product of first two expressions.

Solution 4: Problem solving execution

As thought out we get,

$\displaystyle\frac{(a+b+c)(ab +bc+ca)}{abc}$

$=\displaystyle\frac{3abc+a^2b+ca^2+ab^2+b^2c+bc^2+c^2a}{abc}$

$=3+\displaystyle\frac{a}{c}+\displaystyle\frac{a}{b}+\displaystyle\frac{b}{c}+\displaystyle\frac{b}{a}+\displaystyle\frac{c}{a}+\displaystyle\frac{c}{b}$

So,

$\left(\displaystyle\frac{a}{b}+\displaystyle\frac{b}{a}\right)+\left(\displaystyle\frac{b}{c}+\displaystyle\frac{c}{b}\right)+\left(\displaystyle\frac{c}{a}+\displaystyle\frac{a}{c}\right)$

$=\displaystyle\frac{35}{3}-3$

$=8\displaystyle\frac{2}{3}$.

Apparently the deduction is long, but only seemingly so. Because of the symmetry in the expressions reaching the final outcome is easy.

Answer: Option b: $8\displaystyle\frac{2}{3}$.

Key concepts used: Key pattern identification -- Efficient simplification.

Q5. If $a \neq b \neq c$, then the value of $\displaystyle\frac{a^2+b^2+c^2}{ab+bc+ca}$ is,

1. greater than 1
2. equal to 1
3. less than 1
4. can't be defined

Solution 5: Problem analysis and strategy decision

Recognizing that we need to apply inequality concepts, we identify that we should know the inequality or equality relation between 0 and,

$(a^2+b^2+c^2)-(ab+bc+ca)$.

Let us show how to reach the solution.

Solution 5: Pattern identification and Problem solving execution

Examining the two parts of the expression we identify the pattern that we must use,

$(a^2+b^2+c^2) -(ab +bc+ca)$

$=\displaystyle\frac{1}{2}\left[2(a^2+b^2+c^2)-2(ab+bc+ca)\right]$

$=\displaystyle\frac{1}{2}\left[(a-b)^2+(b-a)^2+(c-a)^2\right] \gt 0$, being sum of squares the expression will always be $\gt 0$ as $a \neq b \neq c$.

So,

$a^2+b^2+c^2 \gt ab+bc+ca$,

Or, $\displaystyle\frac{a^2+b^2+c^2}{ab+bc+ca} \gt 1$, by inequality concepts.

We have used here the pattern and method of zero sum of square terms.

Answer: Option a: greater than 1.

Key concepts used: Key pattern identification -- Deductive reasoning -- Principle of zero sum of square terms -- Efficient simplification -- inequality concepts.

Q6. If $x+y=4$ and $x^2+y^2=14$, where $x \gt y$, the values of $x$ and $y$ respectively are,

1. $2-\sqrt{2}$, $\sqrt{3}$
2. $3$, $1$
3. $2+\sqrt{3}$, $2-\sqrt{3}$
4. $2+\sqrt{3}$, $2\sqrt{2}$

Solution 6: Problem analysis and strategy decision

By examining the problem we decide that finally we need to find the value of $(x-y)$. With value of $(x+y)$ given we would then be able to find values of each of $x$ and $y$.

Working backwards one stage, we again decide, the easiest approach of getting the value of $(x-y)$ would be to raise the first expression to the power of 2, subtract the second expression from it, and get the value of $xy$. One step more will then give us the value of $(x-y)$.

Solution 6: Problem solving execution

The first given equation is,

$x+y=4$,

Or, $(x+y)^2=x^2+2xy+y^2=16$,

As by the second expression, $x^2+y^2=14$,

$2xy=2$.

So,

$x^2-2xy+y^2=(x-y)^2=14-2=12$.

As $x \gt y$ then,

$x-y=2\sqrt{3}$.

Adding with the first expression, $x+y=4$, we have first,

$2x=4+2\sqrt{3}$,

Or, $x=2+\sqrt{3}$.

Similarly,

$y=2-\sqrt{3}$.

Answer: Option c : $2+\sqrt{3}$, $2-\sqrt{3}$.

Key concepts used:  End state analysis -- Pattern identification -- Working backwards strategy.

Q7. If $a^2+b^2+c^2=2(2a-3b-5c)-38$, then the value of $(a-b-c)$ is,

1. $12$
2. $10$
3. $9$
4. $11$

Solution 7: Problem analysis, pattern identification and strategy decision

It doesn't seem to be feasible to get the value of target expression by deductive steps from the given expression which is quite asymetric and unbalanced in nature.

On the other hand accounting for the coefficient values, we could see the ease of transforming the given expression to a sum of square terms equal to zero from which we would get the values of $a$, $b$ and $c$ directly.

Solution 7: Problem solving execution

The given expression is,

$a^2+b^2+c^2=2(2a-3b-5c)-38$

Or, $(a-2)^2+(b+3)^2+(c+5)^2=0$, rearranging the terms and collecting suitable terms together, what we call principle of collection of friendly terms.

As each of the square expressions must be zero for their sum to be zero by principle of zero sum of square terms,

$a-2=0$,

Or, $a=2$.

Similarly,

$b=-3$, and

$c=-5$.

So,

$a-b-c=10$.

Answer: Option b: $10$.

Key concepts used:  Key pattern identificatoion -- Asymmetrtic expression -- Collection of friendly terms -- Zero sum of square terms.

Q8. The expression, $a^2+\displaystyle\frac{1}{a^2} -13a+\displaystyle\frac{13}{a}+34$ converted to product of factors is,

1. $\left(a+\displaystyle\frac{1}{a}-4\right)\left(a+\displaystyle\frac{1}{a}+9\right)$
2. $\left(a-\displaystyle\frac{1}{a}-4\right)\left(a-\displaystyle\frac{1}{a}-9\right)$
3. $\left(a-\displaystyle\frac{1}{a}+4\right)\left(a-\displaystyle\frac{1}{a}+9\right)$
4. $\left(a+\displaystyle\frac{1}{a}+4\right)\left(a-\displaystyle\frac{1}{a}+9\right)$

Solution 8: Problem analysis, pattern identification and solving execution

The obvious method to factorize the given expression is to express it as a suitable quadratic equation in $\left(a+\displaystyle\frac{1}{a}\right)$ or $\left(a-\displaystyle\frac{1}{a}\right)$. By suitablity we mean the quadratic expression that can be factorized easily. On further examination the choice becomes clear. 

The given expression,

$a^2+\displaystyle\frac{1}{a^2} -13a+\displaystyle\frac{13}{a}+34$

$=\left(a-\displaystyle\frac{1}{a}\right)^2-13\left(a-\displaystyle\frac{1}{a}\right) +36$

$=\left(a-\displaystyle\frac{1}{a}-4\right)\left(a-\displaystyle\frac{1}{a}-9\right)$.

We have used component expression substitution of $\left(a-\displaystyle\frac{1}{a}\right)$ mentally.

Answer: Option b: $\left(a-\displaystyle\frac{1}{a}-4\right)\left(a-\displaystyle\frac{1}{a}-9\right)$.

Key concepts used: Problem analysis -- Component expression substitution -- Solving quadratic equation.

Q9. If $a+b+c=0$, then $\displaystyle\frac{2a^2}{b^2+c^2-a^2}+\displaystyle\frac{2b^2}{c^2+a^2-b^2}+\displaystyle\frac{2c^2}{a^2+b^2-c^2}+3$ is equal to,

1. $3$
2. $-3$
3. $-4$
4. $0$

Solution 9: Problem analysis, strategy decision and problem solving

Following the strategy of denominator simplification, we would simplify the third denominator using given $(a+b+c)=0$,

$a+b+c=0$,

Or, $a+b=-c$

Or, $(a+b)^2=c^2$

Or, $a^2+b^2-c^2=-2ab$.

Similarly,

$b^2+c^2-a^2=-2bc$, and

$c^2+a^2-b^2=-2ca$.

Substituting in the given expression,

$\displaystyle\frac{2a^2}{b^2+c^2-a^2}+\displaystyle\frac{2b^2}{c^2+a^2-b^2}+\displaystyle\frac{2c^2}{a^2+b^2-c^2}+3$

$=\displaystyle\frac{2a^2}{-2bc}+\displaystyle\frac{2b^2}{-2ca}+\displaystyle\frac{2c^2}{-2ab}+3$.

Now we must equalize the denominators to sum up the first three terms. We simply multiply the numerator and denominator of the first three terms by $a$, $b$ and $c$ respectively to get,

$\displaystyle\frac{2a^2}{-2bc}+\displaystyle\frac{2b^2}{-2ca}+\displaystyle\frac{2c^2}{-2ab}+3$

$=-\displaystyle\frac{a^3}{abc}-\displaystyle\frac{b^3}{abc}-\displaystyle\frac{c^3}{abc}+3$

$=-\displaystyle\frac{a^3+b^3+c^3}{abc}+3$

$=-\displaystyle\frac{3abc}{abc}+3$, when $a+b+c=0$, $a^3+b^3+c^3=3abc$

$=0$

Answer: Option d: $0$.

Though the deduction steps seemed to be long, the outcome was visible quickly.

Key concepts used: Key pattern identification -- Denominator simplification -- Strategy decision -- Denominator equalization -- Three variable sum of cubes pattern -- Efficient simplification.

Q10. If $a=2+\sqrt{3}$, then the value of $\displaystyle\frac{a^3}{a^6+3a^3+1}$ is,

1. $55$
2. $\displaystyle\frac{3}{55}$
3. $\displaystyle\frac{1}{55}$
4. $\displaystyle\frac{1}{40}$

Solution 10: Problem analysis, pattern identification of sum of inverses and strategy decision

Examining the given surd expression we identify the key pattern of sum of inverses inherent in it,

$a=2+\sqrt{3}=\displaystyle\frac{1}{2-\sqrt{3}}$, as

$(2+\sqrt{3})(2-\sqrt{3})=4-3=1$.

So,

$a+\displaystyle\frac{1}{a}=2+\sqrt{3}+2-\sqrt{3}=4$.

This sum of inverses is a powerful problem solving resource according to the Principle of interaction of inverses. Our strategy would then be converting the target expression in terms of this sum of inverses and substitute each such factor by its value of 4. 

Solution 10: Problem solving execution

We would find the sum of inverses factor in the target expression by the specific pattern identification technique of pairing terms with equal coefficients and power of $x$ differing by an even value.

The target expression,

$\displaystyle\frac{a^3}{a^6+3a^3+1}$

$=\displaystyle\frac{1}{a^3+3+\displaystyle\frac{1}{a^3}}$.

The problem is now transformed to finding the value of $\left(a^3+\displaystyle\frac{1}{a^3}\right)$ when, $\left(a+\displaystyle\frac{1}{a}\right)=4$.

Our intermediate target expression,

$a^3+\displaystyle\frac{1}{a^3}$

$=\left[\left(a+\displaystyle\frac{1}{a}\right)^3-3\left(a+\displaystyle\frac{1}{a}\right)\right]$, as $(x^3+y^3)=(x+y)^3-3xy(x+y)$ and here $xy=1$,

$=4^3-3\times{4}$

$=52$.

So the original target expression would be of value,

$\displaystyle\frac{1}{a^3+3+\displaystyle\frac{1}{a^3}}$

$=\displaystyle\frac{1}{55}$.

Answer: Option b: $\displaystyle\frac{1}{55}$.

Key concepts used: Key pattern identification -- Input transformation to sum of inverses -- Strategy decision -- Pattern identification technique for finding sum of inverses in target expression -- Principle of interaction of inverses -- Sum of cubes rich concept -- patterns and methods.

Note: Observe that most of the solutions could be done in a few steps and largely in mind by first: Problem analysis, second: Key pattern identification and then application of the methods associated with the key pattern. Basically this we call as solution by patterns and methods, that lie at the heart of problem solving quickly in a few steps.

---

Guided help on Algebra in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Algebra in Suresolv, follow the guide,

Suresolv Algebra Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Algebra in Suresolv and is up-to-date.

---