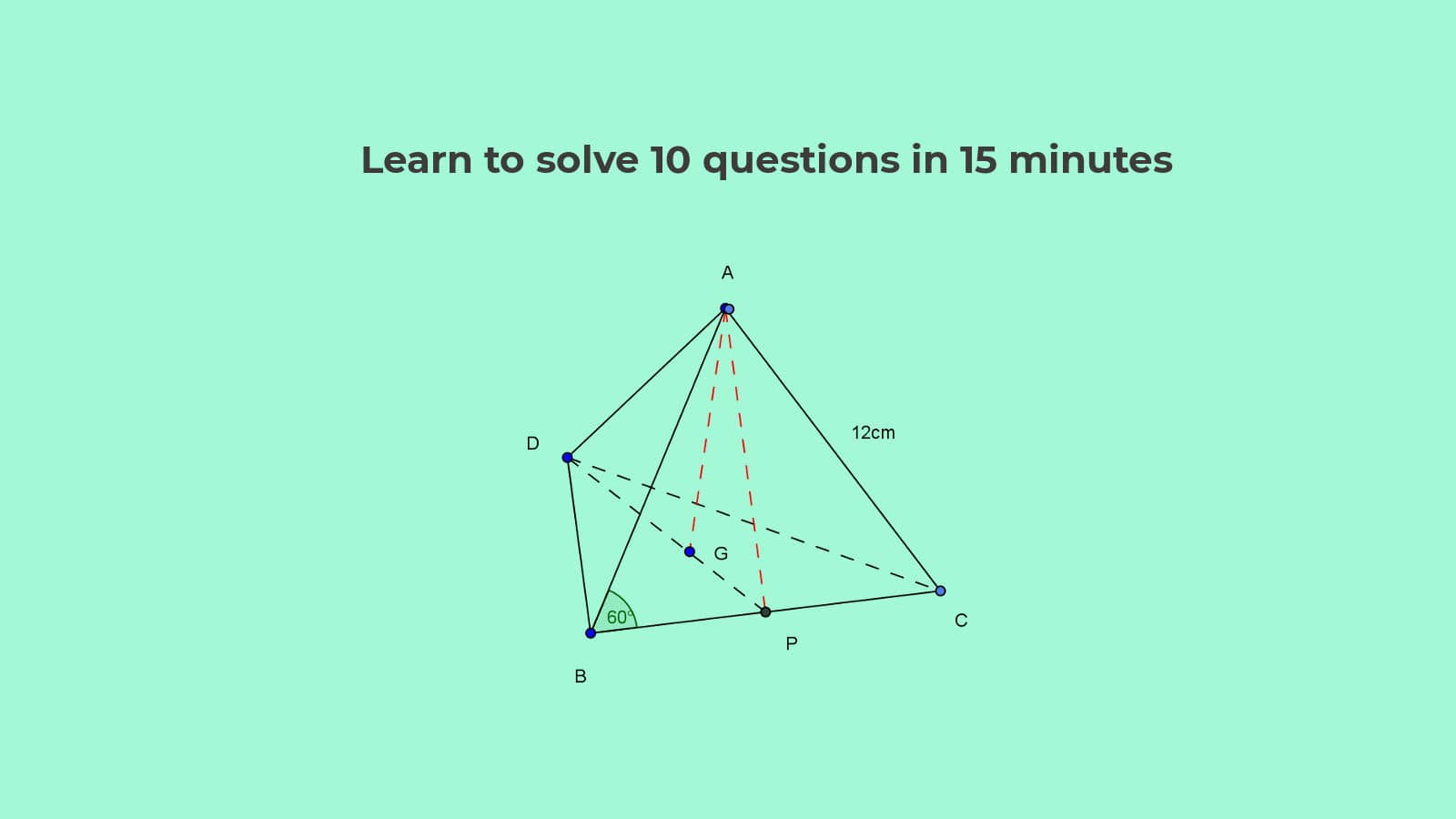
Learn to Solve Questions on Mensuration for SSC CGL Set 41

Submitted by Atanu Chaudhuri on Thu, 06/10/2016 - 13:52

Learn how to solve 10 questions on mensuration for SSC CGL Set 41 in 15 minutes using mensuration formulas and mensuration quick solution techniques.

For best results take the test first at,

SSC CGL level Question Set 41, Mensuration 3.

Quick solutions to questions on mensuration for SSC CGL Set 41 - Answering time was 15 mins

Problem 1.

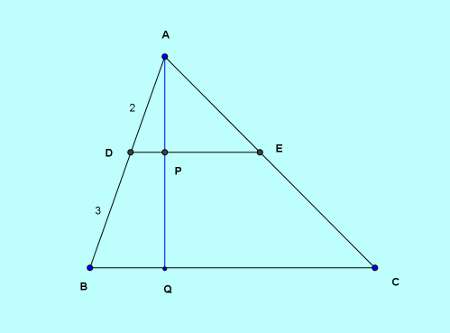
If in $\triangle ABC$, D and E are the two points on the sides AB and AC respectively so that DE || BC and $\displaystyle\frac{AD}{BD}=\displaystyle\frac{2}{3}$, then $\displaystyle\frac{\text{The area of trapezium DECB}}{\text{The area of }\triangle ABC}$ is equal to,

$\displaystyle\frac{21}{25}$
$\displaystyle\frac{5}{9}$
$5\displaystyle\frac{1}{4}$
$1\displaystyle\frac{4}{5}$

Solution 1 - Problem analysis and execution stage 1

The following figure describes the problem,

ssc cgl solution set 41 mensuration 3-1

As, DE || BC, $\triangle ABC$ is similar to $\triangle ADE$ with ratios of corresponding sides equal. So,

$\displaystyle\frac{AB}{AD}=\frac{BC}{DE}$.

Given,

$\displaystyle\frac{AD}{BD}=\frac{2}{3}$,

Or, $\displaystyle\frac{BD}{AD}=\frac{3}{2}$.

Adding 1 to both sides,

$\displaystyle\frac{BD+AD}{AD}=\frac{3+2}{2}$,

Or, $\displaystyle\frac{AB}{AD}=\frac{5}{2}=\frac{BC}{DE}$.

We have transformed the given ratio to match the similar triangle property of equal ratio of corresponding sides. At this stage we got what we wanted, the ratio of the bases of the two triangles. Ratio of rest of sides are not required. Now we need to find the ratio of heights of two triangles.

Our objective is to subtract the area of smaller $\triangle ADE$ from the area of the larger $\triangle ABC$ to get the area of the trapezium without using the formula of area of trapezium.

Solution 1 - Problem analysis and execution stage 2

The perpendicular AQ on BC intersects DE parallel to BC at P so that the two triangles, $\triangle ABQ$ and $\triangle ADP$ are also similar. Thus,

$\displaystyle\frac{AB}{AD}=\frac{5}{2}=\frac{AQ}{AP}$, where AQ and AP are heights of the two triangles.

Solution 1 - Problem execution stage 3

Finally then,

$\displaystyle\frac{\text{The area of trapezium DECB}}{\text{The area of }\triangle ABC}$

$=\displaystyle\frac{\text{Area of }\triangle ABC - \text{Area of }\triangle ADE}{\text{Area of }\triangle ABC}$

$=\displaystyle\frac{\frac{1}{2}BC\times{AQ}-\frac{1}{2}DE\times{AP}}{\frac{1}{2}BC\times{AQ}}$

$=1 - \displaystyle\frac{DE}{BC}\times{\displaystyle\frac{AP}{AQ}}$

$=1-\displaystyle\frac{2^2}{5^2}$

$=1-\displaystyle\frac{4}{25}$

$=\displaystyle\frac{21}{25}$.

Answer: Option a: $\displaystyle\frac{21}{25}$

Key concepts used: Similar triangles concepts -- Similar triangle properties -- Area of triangles concepts.

Note: In this problem, as the sides of the trapezium are to be derived from the similar triangle side ratios, the method followed above should reach you to the solution along the shortest and conceptually elegant path. Try using the formula of area of trapezium to solve the problem to appreciate.

Problem 2.

A solid cone of height 9cm with diameter of its base 18cm is cut out from a wooden solid sphere of radius 9cm. The percentage of wood wasted is,

Solution 2 - Problem analysis and solving

The volume of the sphere is,

$V_s=\frac{4}{3}{\pi}r^3$, where $r$ is the radius of the sphere

$\hspace{6mm}=\frac{4}{3}{\pi}\times{9^3}$ cm$^3$.

The volume of the cone is,

$V_c=\frac{1}{3}{\pi}R^2h$, where $R$ is the radius of the circular base, and $h$ is the height

$\hspace{6mm}=\frac{1}{3}{\pi}9^2\times{9}=\frac{1}{3}{\pi}\times{9^3}$ cm$^3$, which is one-fourth of the volume of the sphere.

So the wood wasted is three-fourth or 75% of the volume of the sphere.

Answer: Option b : 75%.

Key concepts used: Volume concepts of sphere and cone -- percentage concept.

Problem 3.

Two circles with centres A and B and radius 2cm touch each other externally at C. A third circle with centre at C and radius 2cm meets the other two at D and E on the same side of the line AB joining the two centres. Then the area of the quadrilateral ABED is,

$2\sqrt{2}$ sq cm.
$3\sqrt{2}$ sq cm
$2\sqrt{3}$ sq cm
$3\sqrt{3}$ sq cm

Solution 3 - Problem analysis and visualization

The following is the figure corresponding to the problem.

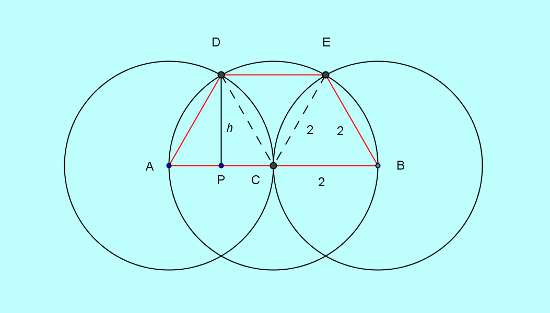
ssc cgl solution set 41 mensuration 3-3

Being radii of three circles of length 2cm each,

$AD=AC=CD$, and

$BC=BE=CE$.

So both $\triangle ACD$ and $\triangle CBE$ are equilateral triangles of side length 2cm. Also,

$\angle ACD = \angle BCE = 60^0$, so that,

$\angle DCE = 60^0$.

In isosceles $\triangle DCE$ then, vertex angle is $60^0$ and two sides,

$CD=CE=2$cm which makes this triangle also equilateral.

Finally then the quadrilateral ABED consists of three equilateral triangles of side length 2cm.

Area of each equilateral triangle is,

$A_t = \frac{1}{2}\times{2}\times{\sqrt{2^2-1^2}}=\sqrt{3}$ sq cm.

So the required area of the quadrilateral ABED$=3\sqrt{3}$ sq cm.

Answer: d: $3\sqrt{3}$ sq cm.

Key concepts used: Circle concepts -- equilateral triangle properties -- area of triangles concept.

Problem 4.

At each corner of a triangular field of side lengths 26m, 28m and 30m, a cow is tethered by a rope of length 7m. The area (in sq m) ungrazed by the cows is,

Solution 4 - Problem analysis

The following figure describes the problem.

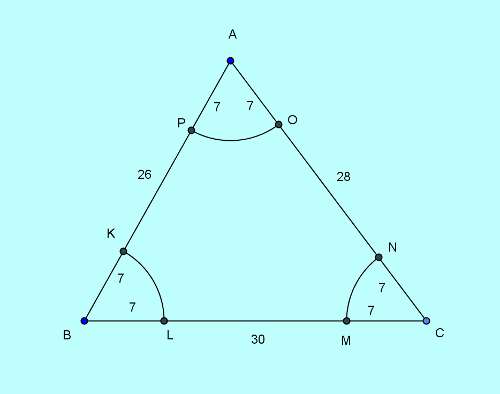
ssc cgl solution set 41 mensuration 3-4

We are to find out the area of the region KLMNOP which is equal to area of the $\triangle ABC$ minus the total area of the three arcs of radius 7cm with centres at A, B and C.

The area covered by each of the arcs will be proportional to the angle covered by the arc in comparison to $360^0$ covered by the whole circle. As we are concerned with total area covered by three arcs, and as the total angle covered by the three arcs is $180^0$, the total area of the three arcs will be,

$\displaystyle\frac{180^0}{360^0}{\pi}{7^2}=77$ sq m, as the radius of each arc is 7m.

Problem execution second stage - area of triangle

Area of triangle when length of three sides are given is,

$A=\sqrt{s(s-a)(s-b)(s-c)}$, where $s=\text{semi-perimeter of the triangle}$, and $a$, $b$ and $c$ are the side lengths.

Here, $s=\frac{1}{2}\times{(26+28+30)}=42$m.

So the area of the triangle is,

$A=\sqrt{42\times{16}\times{14}\times{12}}=336$ sq m.

Finally then the desired area ungrazed by the three cows is,

$\text{Area ungrazed }=336-77=259$ sq m

Answer: Option c: 259 sq m.

Key concepts used: Visualization -- Circle and arc area concept -- Arc angle proportionality concept -- area of triangle based on side lengths.

Problem 5.

ABC is an equilateral triangle of side 2cm. With A, B, C as centre and radius 1 cm three arcs are drawn. The area of the region within the triangle bounded by the three arcs is,

$\left(\displaystyle\frac{\pi}{2} - \sqrt{3}\right)$ sq cm
$\left(\sqrt{3} - \displaystyle\frac{\pi}{2}\right)$ sq cm
$\left(\sqrt{3} - \displaystyle\frac{3\pi}{2}\right)$ sq cm
$3\left(3\sqrt{3} - \displaystyle\frac{\pi}{2}\right)$ sq cm

Solution 5 - Problem analysis and visualization

The following figure represents the problem visual.

ssc cgl solution set 41 mensuration 3-5

The required area is,

$\text{Area of the triangle} - \text{Area covered by the three arcs}$.

Area of the equilateral triangle with side length 2cm is,

$A_t=\frac{1}{2}\times{2}\times{\sqrt{2^2 - 1^2}}=\sqrt{3}$ sq cm

Area of the circle covering $360^0$ and radius 1cm $= \pi$ sq cm.

So area of arc covering $60^0$ and with 1 cm radius $=\displaystyle\frac{\pi}{6}$ sq cm.

Total area of the three such arcs is then $=\displaystyle\frac{\pi}{2}$ sq cm.

Finally thus the required area is,

$\left(\sqrt{3} - \displaystyle\frac{\pi}{2}\right)$ sq cm.

Answer: Option b: $\left(\sqrt{3} - \displaystyle\frac{\pi}{2}\right)$ sq cm.

Key concept used: Area of equilateral triangle concept -- area of an arc subtending a part of whole $360^0$ angle corresponding to the full circle area -- simplification.

Problem 6.

If the length of each side of a regular tetrahedron is 12cm, then the volume of the tetrahedron is,

$144\sqrt{2}$ cm$^3$
$12\sqrt{2}$ cm$^3$
$72\sqrt{2}$ cm$^3$
$8\sqrt{2}$ cm$^3$

Solution 6 - Problem analysis and visualization, first stage

The visualized figure for the problem is as below.

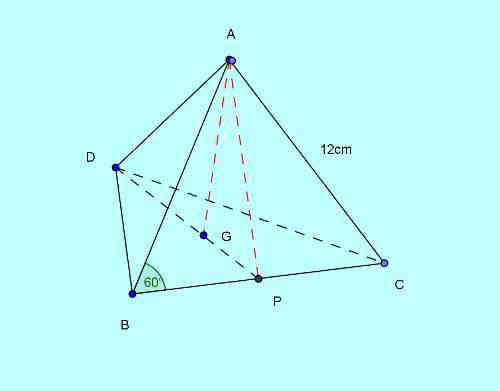
ssc cgl solution set 41 mensuration 3-6

A regular tetrahedron is a three dimensional shape with four faces each face an equilateral triangle. In our problem, the side length of the equilateral triangles is 12 cm.

The volume of a regular tetrahedrom of four sides each of which is an equilateral triangle is,

$\frac{1}{3}\times{\text{Base}}\times{\text{height}} $.

Area of the equilateral triangle base of side length 12cm is,

$\frac{1}{2}\times{12}\times{\sqrt{12^2 - 6^2}}$

$=6\sqrt{108}$ sq cm

$=36\sqrt{3}$ sq cm.

Our job is now to find the height AG of the tetrahedron.

From the figure, AG is perpendicular to the equilateral base from tetrahedron vertex A at G.

As centroid G of the equilateral base corresponds to the centre of gravity of the base, and as the three dimensional regular shape has all four sides as equilateral triangles of same side length, the perpendicular from the vertex to the base must also pass through the centre of gravity of the base, that is, G. AG in this case can be considered as the axis of symmetry of the three dimensional regular shape of tetrahedron.

Problem analysis and execeution - second stage

The perpendicular from vertex to base side of the base face $\triangle BCD$ is the median length,

$DP=\sqrt{12^2 - 6^2}=6\sqrt{3}$.

As G is the centroid of the base face $\triangle BCD$, the length of GP is $\frac{1}{3}$rd of the length of median DP. So,

$GP=2\sqrt{3}$.

Again, AP is the median of the equilateral vertical face $\triangle ABC$. So,

$AP=6\sqrt{3}$.

Thus height of the tetrahedron is,

$AG = \sqrt{AP^2 - GP^2}=\sqrt{108 - 12}=4\sqrt{6}$.

Finally we have then the volume of the tetrahedron as,

$=\frac{1}{3}\times{36\sqrt{3}}\times{4\sqrt{6}}$ cm$^3$

$=144\sqrt{2}$ cm$^3$.

Answer: Option a : $144\sqrt{2}$ cm$^3$.

Key concepts used: Tetrahedrom concept -- visualization -- centroid as centre of gravity concept -- median section ratio at centroid -- area of an equilateral triangle -- Pythagoras theorem.

Problem 7.

The surface area of three adjacent faces of a cuboid are, p, q and r. Its volume is,

$\sqrt{pq^2 + qr^2 + rp^2}$
$\sqrt{(p^2+q^2+r^2)(p+q+r)}$
$\sqrt{pqr}$
$(\sqrt{pq}+\sqrt{qr}+\sqrt{rp})(p^2+q^2+r^2)$

Solution 7 - Problem visualization and solution

The corresponding figure is as below.

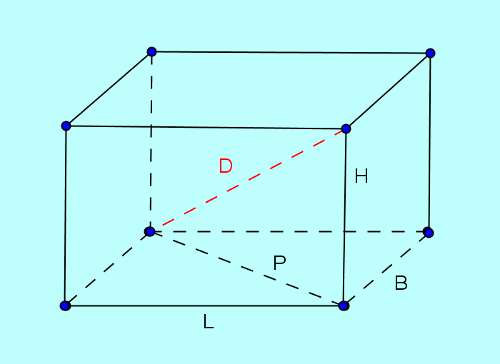
ssc cgl solution set 41 mensuration 3-7

The area of the cuboid face with side lengths L and B is, $p=LB$.

The area of the cuboid face with side lengths L and H is, $q=LH$.

The area of the cuboid face with side lengths B and H is, $r=BH$.

Multiplying the three we have,

$pqr = L^2\times{B^2}\times{H^2}$.

So the volume of the cuboid is,

$V = LBH = \sqrt{pqr}$.

Answer: c: $\sqrt{pqr}$.

Key concepts used: Cuboid visualization -- area of cuboid face concept -- volume of cuboid concept.

Problem 8.

The radii of the base of a cylinder and a cone are in the ratio $\sqrt{3}:\sqrt{2}$ and their heights are in the ratio of $\sqrt{2}:\sqrt{3}$. Their volumes are in the ratio of,

$\sqrt{2}:\sqrt{6}$
$\sqrt{3}:2\sqrt{2}$
$3\sqrt{3}:\sqrt{2}$
$\sqrt{3}:\sqrt{2}$

Solution 8 - Problem analysis and execution

Let $r_1$ and $r_2$ be the radii and $h_1$ and $h_2$ be the heights of the cylinder and the cone.

So,

$\displaystyle\frac{r_1}{r_2}=\frac{\sqrt{3}}{\sqrt{2}}$, and

$\displaystyle\frac{h_1}{h_2}=\frac{\sqrt{2}}{\sqrt{3}}$.

Volume of cylinder is,

$V_{cylinder}={\pi}{r_1}^2h_1$.

Volume of the cone is,

$V_{cone}=\frac{1}{3}{\pi}{r_2}^2h_2$.

So ratio of volumes of the cylinder and the cone is,

$\displaystyle\frac{V_{cylinder}}{V_{cone}} = 3\displaystyle\frac{{r_1}^2}{{r_2}^2}\times{\displaystyle\frac{h_1}{h_2}}$

$=3\times{\displaystyle\frac{3}{2}}\times{\displaystyle\frac{\sqrt{2}}{\sqrt{3}}}$

$=\displaystyle\frac{3\sqrt{3}}{\sqrt{2}}$

$=3\sqrt{3} : \sqrt{2}$.

Answer: Option c: $3\sqrt{3} : \sqrt{2}$.

Key concepts used: Volumes of cylinder and cone -- ratio concepts -- simplification.

Problem 9.

The height of a cone is 30cm. A small cone is cut-off at the top by a plane parallel to its base. If its volume is $\displaystyle\frac{1}{27}$th of the volume of the cone, at what height above the base is the section made?

10cm
6cm
8cm
20cm

Solution 9 - Problem analysis and visualization

The following figure corresponds to visualization of the problem.

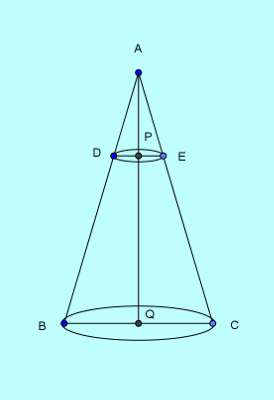
ssc cgl solution set 41 mensuration 3-9

$AQ=30$cm is the height of the cone ABC. A plane parallel to the base and passing through the line DE cuts off the small cone ADE from the top.

Ratio of volume of the smaller to the larger cone is $\frac{1}{27}$. We are to find the height PQ from base at which the smaller cone section is made.

As the section is made by a plane parallel to the base, the $\triangle ABC$ and $\triangle ADE$ are similar and also $\triangle APE$ and $\triangle AQC$ are similar. Here APQ is the perpendicular dropped from the vertex of the larger cone to its base so that AP also is perpendicular to the base of the smaller cone. BC and DE are diameters of the circular cross sections or bases of the two cones and are parallel to each other.

Problem execution - stage 2

The similarity of the triangles results in equal ratios of corresponding sides so that,

$\displaystyle\frac{PE}{QC}=\frac{AP}{AQ}$.

Volumes of the two cones are,

$V_{small}=\frac{1}{3}{\pi}{PE^2}\times{AP}$, and

$V_{large}=\frac{1}{3}{\pi}{QC^2}\times{AQ}$, where PE and QC are the radii and AP and AQ are the heights.

So the ratio of two volumes is,

$\displaystyle\frac{V_{small}}{V_{large}} = \frac{PE^2\times{AP}}{{QC^2}\times{AQ}}=\frac{1}{27}$

Or, $\left(\displaystyle\frac{AP}{AQ}\right)^3=\displaystyle\frac{1}{27}=\left(\displaystyle\frac{1}{3}\right)^3$.

Thus,

$\displaystyle\frac{AP}{AQ}=\frac{1}{3}$.

As $AQ = 30$cm, $AP=10$cm and,

$PQ=30-10=20$cm.

Answer: Option d: 20cm.

Key concepts used: Visualization -- cone volume concept -- triangle similarity rich concepts -- ratio concepts -- simplification.

Problem 10.

The radius of a cylinder is 10cm and height is 4cm. The number of centimetres that may be added either to the radius or to the height to get the same increase in volume is,

5cm
4cm
25cm
16cm

Solution 10 - Problem analysis

The volume of the cyclinder is,

$V_{initial}={\pi}{10^2}4$ sq cm

As equal change in radius of base in one case and of height in the second case results in same area change, the best possible course of deduction is chosen as an algebraic deduction process with equal change in either base radius or height assumed as $x$.

Problem solving execution

Increasing the radius of base by $x$ cm, the change of area becomes,

${\pi}{(10+x)^2}4 - {\pi}{10^2}4={\pi}{(20x+x^2})4$.

Similarly the change in area by increasing height by $x$ cm is,

${\pi}{10^2}(4+x) - {\pi}{10^2}4={\pi}100x$.

Equating the two,

${\pi}{(20x+x^2})4={\pi}100x$

Or, $4x^2=20x$

Or, $x=5$, considering $x \neq 0$.

Answer: a: 5 cm.

Key concepts used: Cylinder volume concept -- change analysis technique -- algebraic equations.

You are here

SSC CGL level Solution Set 41, Mensuration 3