You are here

Home » SSC CGL »

SSC CGL level Solution Set 47, Fractions decimals and surds 2

Submitted by Atanu Chaudhuri on Wed, 30/11/2016 - 09:26

Fractions Decimals BODMAS questions solved SSC CGL solutions 47

Solution set 47: BODMAS fraction questions, Decimal BODMAS questions

BODMAS fraction questions, Decimal BODMAS questions in SSC CGL solution set 47 solved easy and quick in 18 minutes using BODMAS and other rules.

Contents are,

  1. BODMAS fraction questions solved.
  2. Decimal BODMAS questions solved.
  3. Surds questions solved.
  4. All solutions are in minimum time.

If you have not taken the corresponding test yet, first take the test by referring to SSC CGL level Question Set 47 on fractions decimals surds 2 and then come back for the solution.

BODMAS fraction questions, Decimal BODMAS questions and SURDS questions SSC CGL Solution set 47 - time to solve was 18 mins

Problem 1.

Simplify $\displaystyle\frac{\displaystyle\frac{1}{3} + \displaystyle\frac{1}{4}\left[\displaystyle\frac{2}{5}-\displaystyle\frac{1}{2}\right]}{1\displaystyle\frac{2}{3}\text{ of } \displaystyle\frac{3}{4} - \displaystyle\frac{3}{4}\text{ of }\displaystyle\frac{4}{5}}$

  1. $\displaystyle\frac{74}{78}$
  2. $\displaystyle\frac{37}{13}$
  3. $\displaystyle\frac{37}{78}$
  4. $\displaystyle\frac{74}{13}$

Solution 1 : Problem analysis and execution:

We decided to evaluate the subtraction within the brackets in the numerator first as brackets come first by the well known BODMAS rule of order of evaluation of expressions,

$\left[\displaystyle\frac{2}{5}-\displaystyle\frac{1}{2}\right]=-\displaystyle\frac{1}{10}$.

This simplifies the numerator to,

$\displaystyle\frac{1}{3}-\displaystyle\frac{1}{40}=\displaystyle\frac{37}{120}$.

Till now it has been possible for us to do the simplification mentally. For the second subtraction we converted the first fraction to, $\frac{40}{120}$ and the second to, $\frac{3}{120}$.

Looking at the denominator we detected the possiblity of cancelling out 3 in the first term and 4 in the second term giving,

$\displaystyle\frac{5}{4}-\displaystyle\frac{3}{5}$.

This again could be done mentally.

Now we take a quick path of bringing down the denominator 120 of the fraction in the numerator and multiply it with the denominator terms. We foresaw that this action will transform both fractions in numerator and denominator to integers. The target expression is thus simplified to,

$E=\displaystyle\frac{37}{150-72}=\frac{37}{78}$.

We felt that efficient simplification has been achieved.

The step of multiplying the numerator and denominator of the transformed target expression by 120 did two positive things,

  • it transformed both the numerator and denominator from fraction to integers, thus simplifying the next steps significantly, and
  • it joined the already evaluated numerator with the denominator relieving us of keeping the numerator value in our memory any longer. We could of course have written down the intermediate numerator evaluation result $\displaystyle\frac{37}{120}$ in our scratch pad. This approach is recommended.

Recommendation

In complex simplification process, write down the intermediate results in scratch pad instead of keeping them in memory. This is a more reliable method increasing accuracy without sacrificing speed.

Answer: c: $\displaystyle\frac{37}{78}$.

Key concepts used: Simplification evaluation order of BODMAS -- fraction addition and subtraction -- cancelling out factors at the earliest opportunity -- simplification technique -- transforming both the numerator and denominator from fractions to integers at one stroke -- efficient simplification.

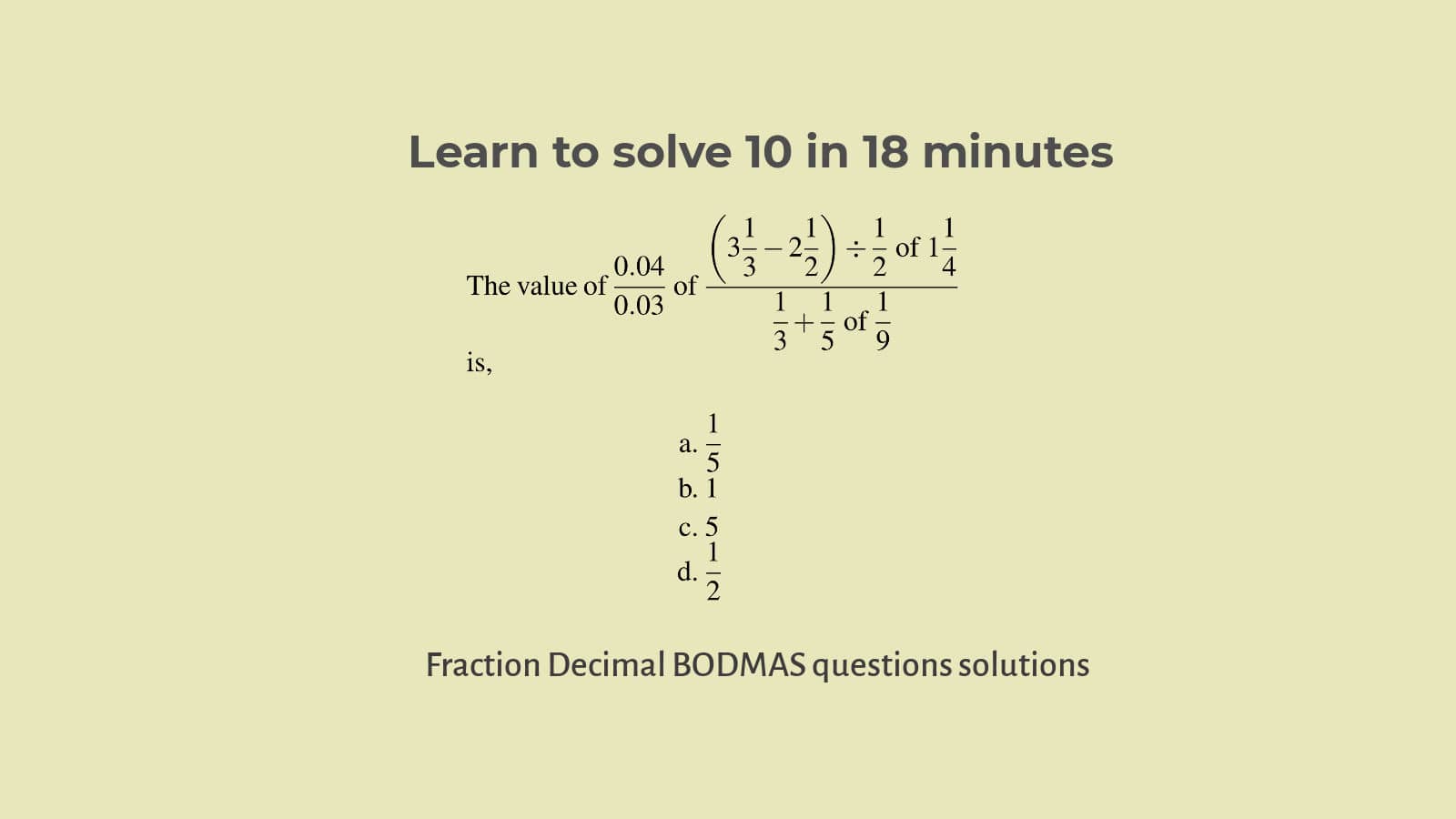
Problem 2.

The value of $\displaystyle\frac{0.04}{0.03}\text { of }\displaystyle\frac{\left(3\displaystyle\frac{1}{3}-2\displaystyle\frac{1}{2}\right)\div{\displaystyle\frac{1}{2}}\text{ of }1\displaystyle\frac{1}{4}}{\displaystyle\frac{1}{3}+\displaystyle\frac{1}{5}\text{ of }\displaystyle\frac{1}{9}}$ is,

  1. $\displaystyle\frac{1}{5}$
  2. $1$
  3. $5$
  4. $\displaystyle\frac{1}{2}$

Solution 2 : Problem analysis and execution

First we focused our attention to the numerator. Noticing the subtraction involved both terms as mixed fractions, instead of transforming the mixed fractions to pure fractions and then subtracting, we resorted to mentally break up the mixed fractions into integer and fraction parts and then carry out the integer and fraction subtraction separately.

$3\displaystyle\frac{1}{3}-2\displaystyle\frac{1}{2}=(3-2)+\left(\displaystyle\frac{1}{3}-\displaystyle\frac{1}{2}\right)=\displaystyle\frac{5}{6}$.

For larger integer parts this mixed fraction split up arithmetic speeds up simplification significantly.

The negative subtraction result had to be taken care of on the go.

Now we need to explicitly describe the BODMAS rule of simplification before taking further steps.

BODMAS rule of simplification of fraction and decimal expressions

The B stands for brackets and will have the highest priority in simplification. Proceeding from left to right, the expressions within brackets have to be evaluated first.

The O stands for powers and also "Of". The "Of" is equivalent to multiplication, but when used as "Of", its priority is higher than either division or multiplication but lower than Brackets. For example,

$\left(\frac{10}{3}-\frac{5}{2}\right)\div{\frac{1}{2}}\text{ of }\frac{5}{4}=\left(\frac{5}{6}\right)\div{\left(\frac{5}{8}\right)}=\frac{8}{6}=\frac{4}{3}$.

The $\text{ of }$ will be executed before the division as it has higher priority of execution than either division or multiplication.

The D stands for Division and M for multiplication, both having equal priority. Whichever operation of Division or Multiplication is encountered first in the processing from left to right will be executed first. Both division and multiplication operations have lower priority than Of and Brackets.

Lastly, A stands for Addition and S for Subtraction both having equal priority of execution, precedence between themselves determined by appearance in the expression from left to right. Both of these operations have lowest priority of execution in evaluation of expressions, and will be executed last.

Execution is carried out from left to right in the normal process taking care of relative priorities of BODMAS operands.

Solution 2 - further simplification

Thus applying BODMAS rule, the numerator of the major fraction part is simplified to, $\displaystyle\frac{4}{3}$.

Similarly the denominator is simplified to,

$\displaystyle\frac{1}{3} + \displaystyle\frac{1}{45}=\displaystyle\frac{16}{45}$.

Finally then the target expression is simplified as,

$\displaystyle\frac{4}{3}\times{\displaystyle\frac{4}{3}}\times{\displaystyle\frac{45}{16}}=5$.

Note that we evaluated the target expression part by part and in each part BODMAS rule was applied.

Answer: Option c : $5$ .

Key concepts used: BODMAS rule of simplification -- Problem breakdown technique in breaking up the expression into parts, usually into numerator evaluation, denominator evaluation and so on -- mixed fraction split up arithmetic for faster simplification.

Problem 3.

$\sqrt{\displaystyle\frac{(6.1)^2+(61.1)^2+(611.1)^2}{(0.61)^2+(6.11)^2+(61.11)^2}}$ is equal to,

  1. 0.1
  2. 100
  3. 1.1
  4. 10

Solution 3 : Problem analysis and execution:

On comparison of the similar looking terms in the numerator and denominator it became apparent that each term in the denominator inside the squares is one tenth of corresponding term inside the squares in the numerator.

As we factor this $\displaystyle\frac{1}{10}$ out of each square in the denominator, it is transformed to a factor of $\displaystyle\frac{1}{100}$ which again is transformed to $\displaystyle\frac{1}{10}$ as we take this factor in the denominator out of the square root. This makes the denominator and the numerator equal leaving a fator of $10$ in the numerator.

The process is carried out in mind. We will show the steps though as below. Target expression is transformed to,

$E=\sqrt{\displaystyle\frac{(6.1)^2+(61.1)^2+(611.1)^2}{(0.61)^2+(6.11)^2+(61.11)^2}}$

$=\sqrt{\displaystyle\frac{(6.1)^2+(61.1)^2+(611.1)^2}{(\frac{1}{10}\times{6.1})^2+(\frac{1}{10}\times{61.1})^2+(\frac{1}{10}\times{611.1})^2}}$

$=\sqrt{\displaystyle\frac{(6.1)^2+(61.1)^2+(611.1)^2}{\frac{1}{100}(6.1)^2+\frac{1}{100}(61.1)^2+\frac{1}{100}(611.1)^2}}$

$=10$

Answer: Option d: 10.

Key concepts used: Useful pattern identification-- decimal point shifting -- decimal arithmetic.

Problem 4.

$(0.\overline{1})^2\left[1-9(0.1\overline{6})^2\right]$ is equal to,

  1. $-\displaystyle\frac{1}{162}$
  2. $\displaystyle\frac{1}{109}$
  3. $\displaystyle\frac{1}{108}$
  4. $\displaystyle\frac{7696}{10^6}$

Solution 4 : Problem analysis and execution:

Generally with problems involving repeating decimals we first convert each repeating decimal term to its corresponding fraction. Here also we take this step first.

$0.\overline{1}=\displaystyle\frac{1}{9}$, this is straightforward.

$0.1\overline{6}=\displaystyle\frac{16-1}{90}=\frac{15}{90}=\frac{1}{6}$. Here we have used the rich repeating decimal conversion technique explained below.

Rich techniques of repeating decimal conversion to fraction

This rich technique helps to convert repeating decimals in two forms, such as, $0.\overline{16}$, where the repeating sequence starts just after the decimal point and $0.24\overline{37}$, where the repeating sequence starts after at least one place right of the decimal point. Treatment of conversion are a little different in two cases.

We will first show the conventional basic concept based methods that take a bit of time and the rich techniques that are based on the basic concepts but are fast and simple processes.

Once you know how the rich techniques of repeating decimal conversion actually work you can always use the simple to execute rich techniques instead of adopting the more time consuming basic methods.

We explain conversion of repeating decimal of both forms through the examples mentioned.

Conversion of $0.\overline{16}$ to fraction - conventional conceptual method and rich technique

Let us assume,

$x=0.\overline{16}$.

Multiplying both sides by 100 (as there are two digits in the repeating sequence) we get,

$100x=16.\overline{16}=16+0.\overline{16}=16+x$,

Or, $99x=16$,

Or, $x=\displaystyle\frac{16}{99}$.

We describe the corresponding rich technique as,

If the repeating digit sequence starts just after the decimal point, the equivalent fraction will be given by, numerator as the repeating digit sequence itself and the denominator as a number comprising of 9s of length same as the repeating digit sequence.

Conversion of $0.24\overline{37}$ - Conventional approach and rich technique

Same as before we will assume,

$x=0.24\overline{37}$

$=0.24+0.00\overline{37}$

$=\displaystyle\frac{24}{100}+\displaystyle\frac{37}{9900}$, taking $\frac{1}{100}$ as a factor, converting $0.\overline{37}$ to $\frac{37}{99}$ and reapplying the factor taken out.

Or, $x=\displaystyle\frac{24\times{99}+37}{9900}$

At this point following the conventional path we would have to calculate the numerator and form the fraction as,

$0.24\overline{37}=\displaystyle\frac{24\times{99}+37}{9900}=\displaystyle\frac{2413}{9900}$.

Instead we would take slighly different path to form the numerator easily,

$0.24\overline{37}=\displaystyle\frac{24\times{99}+37}{9900}$

$=\displaystyle\frac{24\times{(99+1)}+37-24}{9900}$

$=\displaystyle\frac{2400+37-24}{9900}$

$=\displaystyle\frac{2437-24}{9900}$

$=\displaystyle\frac{2413}{9900}$, the same result as before.

Similarly to convert say. $0.6\overline{43}$ to fraction, we will form the numerator simply by subtracting 6, the digit sequence outside the repeating sequence from the number formed by all the digits of the decimal. The denominator will start with as many 9s as the length of the repeating sequence as before, but will be suffixed by zeros of length of the non-repeating part of the decimal. In this second case then,

$0.6\overline{43}=\displaystyle\frac{643-6}{990}=\frac{637}{990}$.

To state this second form of repeating decimal conversion technique formally,

The numerator will be, the number formed from all the digits of the decimal including the first appearance of the repeating sequence minus the number formed from the non-repeating digits, while the denominator will consist of 9s of length same as the length of repeating portion suffixed by zeros of length same as the non-repeating part of the decimal.

If you think a bit, you will see that this is a more general technique that includes the first simpler case. In the case of repeating sequence starting right after the decimal, the number subtracted in the numerator will be 0 and the number of zeros suffixing the sequence of 9s in the denominator will also be 0.

Note: This is not a trick formula but a valuable simple to use rich technique derived from basic concepts for conversion of any form of rational repeating decimal to its equivalent fraction.

Coming back to our problem then, the target expression is transformed to,

$E=(0.\overline{1})^2\left[1-9(0.1\overline{6})^2\right]$

$=\left(\displaystyle\frac{1}{9}\right)^2\left[1-9\times{\left(\displaystyle\frac{1}{6}\right)^2}\right]$

$=\displaystyle\frac{1}{81}\left[1-\displaystyle\frac{1}{4}\right]$

$=\displaystyle\frac{1}{108}$.

Answer: c: $\displaystyle\frac{1}{108}$.

Key concepts used: Rich techniques of repeating decimal conversion to fraction -- efficient simplification by eliminating factors between numerator and denominator as far as possible.

Note: It is recommended for this problem that one should write at least some part of the steps on paper for ensuring speed with accuracy.

Problem 5.

The value of $\displaystyle\frac{2\displaystyle\frac{1}{3}-1\displaystyle\frac{2}{11}}{3+\displaystyle\frac{1}{3+\displaystyle\frac{1}{3+\displaystyle\frac{1}{3}}}}$ is,

  1. $\displaystyle\frac{38}{109}$
  2. $\displaystyle\frac{116}{109}$
  3. $1$
  4. $\displaystyle\frac{109}{38}$

Solution 5 : Problem analysis and execution:

As a part of efficient simplification process we first take up simplifying the denominator which in any case we have to do. This in fact is the essence of simplification technique. The denominator structure being repeating fractions and simple, we could easily evaluate mentally its final value as, $\displaystyle\frac{109}{33}$ transforming the target expression to,

$E=\displaystyle\frac{33}{109}\left[2\displaystyle\frac{1}{3}-1\displaystyle\frac{2}{11}\right]$

Now we apply our powerful mixed fraction split expression arithmetic technique and without evaluating the terms and the expression inside the bracket, we multiply the terms by 33,

$E=\displaystyle\frac{1}{109}\left[33(2-1)+(11-6)\right]$

$=\displaystyle\frac{1}{109}\left[38\right]$

$=\displaystyle\frac{38}{109}$.

The whole simplification could be done by the simple process mentally within a minute. This is a good example of applying mixed fraction split expression arithmetic technique as a part of efficient simplification practices.

Recommendation: If you find doing mental continued fraction evaluation a bit difficult, then write down parts of the results to ensure accuracy.

Answer: Option a: $\displaystyle\frac{38}{109}$.

Key concepts used: Mental continued fraction evaluation -- mixed fraction split expression arithmetic -- efficient simplification.

We will show you the simple steps of evaluating the continued fraction denominator,

$3+\displaystyle\frac{1}{3+\displaystyle\frac{1}{3+\displaystyle\frac{1}{3}}}$

$=3+\displaystyle\frac{1}{3+\displaystyle\frac{1}{\displaystyle\frac{10}{3}}}$

$=3+\displaystyle\frac{1}{3+\displaystyle\frac{3}{10}}$

$=3+\displaystyle\frac{1}{\displaystyle\frac{33}{10}}$

$=3+\displaystyle\frac{10}{33}$

$=\displaystyle\frac{109}{33}$

Problem 6.

Find the value of $27\times{1.\overline{2}}\times{5.526\overline{2}}\times{0.\overline{6}}$.

  1. $121.7\overline{5}$
  2. $121.\overline{75}$
  3. $121.\overline{57}$
  4. $121.576\overline{8}$

Solution 6 : Problem analysis and execution:

Examining the four product terms in repeating decimals we decide to keep aside the more complex third term for the time being and evaluate the product of the other three terms.

$E=27\times{1.\overline{2}}\times{5.526\overline{2}}\times{0.\overline{6}}$

$=27\times{1\frac{2}{9}}\times{0.\overline{6}}\times{5.526\overline{2}}$

$=27\times{\frac{11}{9}}\times{\frac{6}{9}}\times{5.526\overline{2}}$

$=33\times{\frac{2}{3}}\times{5.526\overline{2}}$

$=22\times{(5.526+0.000\overline{2})}$

$=110+11+22\times{0.026}+22\times{\frac{2}{9000}}$

$=121+0.44+22\times{0.006}+\frac{44}{9000}$

$=121.572+\frac{44}{9000}$

$=121.572+\frac{1}{1000}\frac{44}{9}$

$=121.572+\frac{1}{1000}(4.\overline{8})$

$=121.576\overline{8}$.

Answer: Option d : $121.576\overline{8}$.

Though the steps were shown in detail, we adopted the path of by hand one step multiplication of 22 by 5.526 writing the two in manual multiplicative form. This took small time. Evaluation of the overline repeating portion was also quick. Still, thinking of the strategy and executing it took more than a minute's time, even after application of the efficient simplification techniques.

Key concepts used: Problem breakdown into a simple and a difficult part -- problem breakdown by difficulty -- Simplification technique applied to simplify the three easily computable term product first --- repeating decimal to fraction conversion techniques.

Problem 7.

If $\displaystyle\frac{(x-\sqrt{24})(\sqrt{75}+\sqrt{50})}{\sqrt{75}-\sqrt{50}}=1$, then $x$ is,

  1. $5$
  2. $\sqrt{5}$
  3. $3\sqrt{5}$
  4. $2\sqrt{5}$

Solution 7 : Problem analysis and execution:

Examining the given expression we detect the presence of $\sqrt{25}=5$ as a factor of both denominator and numerator. Eliminating the factor the given expression is simplified to,

$\displaystyle\frac{(x-\sqrt{24})(\sqrt{75}+\sqrt{50})}{\sqrt{75}-\sqrt{50}}=1$,

Or, $\displaystyle\frac{(x-2\sqrt{6})(\sqrt{3}+\sqrt{2})}{\sqrt{3}-\sqrt{2}}=1$.

Rationalizing,

$(x-2\sqrt{6})(\sqrt{3}+\sqrt{2})^2=1$,

Or, $(x-2\sqrt{6})(5+2\sqrt{6})=1$,

Or, $x-2\sqrt{6}=\displaystyle\frac{1}{5+2\sqrt{6}}$.

Now rationalizing the RHS,

$x-2\sqrt{6}=5-2\sqrt{6}$,

Or, $x=5$.

Answer: Option a: $5$.

Key concepts used: Pattern identification -- surd rationalization to simplify -- input transformation to apply rationalization again to simplify to the solution directly -- successive surd rationalization -- surd simplification -- efficient simplification.

Problem 8.

$\displaystyle\frac{1}{\sqrt{2}+\sqrt{3}-\sqrt{5}}+\displaystyle\frac{1}{\sqrt{2}-\sqrt{3}-\sqrt{5}}$ is equal to,

  1. $\displaystyle\frac{1}{\sqrt{2}}$
  2. $0$
  3. $\sqrt{2}$
  4. $1$

Solution 8 : Problem analysis and execution:

Comparing the two denominators the opportunity to express them in the form of $(a+b)$ and $(a-b)$ could easily be identified. Following this path when we combine the two terms, the denominator is tramsformed to $a^2-b^2=(\sqrt{2}-\sqrt{5})^2 - (\sqrt{3})^2=4-2\sqrt{10}=2\sqrt{2}(\sqrt{2}-\sqrt{5})$.

In the numerator, the two $b$s cancel out, leaving $2a$, that is, $2(\sqrt{2}-\sqrt{5})$.

Thus the final result turns out to be, $\displaystyle\frac{1}{\sqrt{2}}$.

Let us show you the steps that should be done mentally,

$E=\displaystyle\frac{1}{\sqrt{2}+\sqrt{3}-\sqrt{5}}+\displaystyle\frac{1}{\sqrt{2}-\sqrt{3}-\sqrt{5}}$

$=\displaystyle\frac{1}{(\sqrt{2}-\sqrt{5}) +\sqrt{3}}+\displaystyle\frac{1}{(\sqrt{2}-\sqrt{5})-\sqrt{3}}$

$=\displaystyle\frac{2(\sqrt{2}-\sqrt{5})}{(\sqrt{2}-\sqrt{5})^2 - 3}$

$=\displaystyle\frac{2(\sqrt{2}-\sqrt{5})}{4-2\sqrt{10}}$

$=\displaystyle\frac{2(\sqrt{2}-\sqrt{5})}{2\sqrt{2}(\sqrt{2}-\sqrt{5})}$

$=\displaystyle\frac{1}{\sqrt{2}}$.

Answer: Option a: $\displaystyle\frac{1}{\sqrt{2}}$.

Key concepts used: Basic algebra concept of using the relationship $(a+b)(a-b)=a^2-b^2$ -- useful pattern identification -- surd factorization -- surd simplification -- efficient simplification.

Problem 9.

$\left[8-\left[\displaystyle\frac{4^{\frac{9}{4}}\sqrt{2.2^2}}{2\sqrt{2^{-2}}}\right]^\frac{1}{2}\right]$ is equal to,

  1. 1
  2. 32
  3. 0
  4. 8

Solution 9 : Problem analysis and execution:

The problem though looks awkwardly complex, we find it simple at the core because we need just to carefully keep track of the powers or indices while equalizing the base of every term to 2. It is nothing but a bit involved base equalization indices problem.

The target expression is transformed thus to,

$E=\left[8-\left[\displaystyle\frac{4^{\frac{9}{4}}\sqrt{2.2^2}}{2\sqrt{2^{-2}}}\right]^\frac{1}{2}\right]$

$=\left[8-\left[\displaystyle\frac{2^{\frac{9}{2}}\sqrt{2^3}}{2\sqrt{2^{-2}}}\right]^\frac{1}{2}\right]$

$=\left[8-\left[\displaystyle\frac{2^{\frac{9}{2}}2^{\frac{3}{2}}}{2\sqrt{2^{-2}}}\right]^\frac{1}{2}\right]$

$=\left[8-\left[\displaystyle\frac{2^6}{1}\right]^\frac{1}{2}\right]$

$=\left[8-2^3\right]$

$=0$

Answer: Option c: 0.

Key concepts used: Base equalization technique -- indices concepts -- indices arithmetic -- effficient simplification.

Problem 10.

The value of $\sqrt{\displaystyle\frac{(\sqrt{12}-\sqrt{8})(\sqrt{3}+\sqrt{2})}{5+\sqrt{24}}}$ is,

  1. $\sqrt{6}-\sqrt{2}$
  2. $\sqrt{6}-2$
  3. $2-\sqrt{6}$
  4. $\sqrt{6}+\sqrt{2}$

Solution 10 : Problem analysis and execution:

Being experienced in surd expression transformation and also following the usual simplifying step of focusing on the denominator, we recognize $5+\sqrt{24}=5+2\sqrt{6}=(\sqrt{3}+\sqrt{2})^2$.

Getting this square of $(\sqrt{3}+\sqrt{2})$ is good for simplification as one part of it is straightway canceled out with the factor in numerator. This leaves the expression, $(\sqrt{3}+\sqrt{2})$ in the denominator.

Again we rationalize the denominator and also simplify the factor, $(\sqrt{12}-\sqrt{8})=2(\sqrt{3}-\sqrt{2})$. The result is, $\sqrt{2}(\sqrt{3}-\sqrt{2})=\sqrt{6}-2$.

All the steps could be done mentally.

Let use show the steps,

$E=\sqrt{\displaystyle\frac{(\sqrt{12}-\sqrt{8})(\sqrt{3}+\sqrt{2})}{5+\sqrt{24}}}$

$=\sqrt{\displaystyle\frac{(\sqrt{12}-\sqrt{8})(\sqrt{3}+\sqrt{2})}{5+2\sqrt{6}}}$

$=\sqrt{\displaystyle\frac{(\sqrt{12}-\sqrt{8})(\sqrt{3}+\sqrt{2})}{(\sqrt{3}+\sqrt{2})^2}}$

$=\sqrt{\displaystyle\frac{(\sqrt{12}-\sqrt{8})}{(\sqrt{3}+\sqrt{2})}}$

$=\sqrt{\displaystyle\frac{2(\sqrt{3}-\sqrt{2})}{(\sqrt{3}+\sqrt{2})}}$

$=\sqrt{2(\sqrt{3}-\sqrt{2})^2}$

$=\sqrt{2}(\sqrt{3}-\sqrt{2})$

$=\sqrt{6}-2$.

Answer: Option b:$\sqrt{6}-2$.

Key concepts used: Pattern identification -- conversion to square of sum surd expression -- surd rationalization -- surd simplification -- efficient simplification -- denominator simplification.

Note: Much of the time-consuming deductions in the problems can be avoided by adopting efficient simplification strategies and techniques.

You may refer to the following related articles.

Guided help on Fractions, Surds and Indices in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on fractions, surds and indices in Suresolv, follow the guide,

Suresolv Fractions, Surds and Indices Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Fractions, Surds and Indices​ and relevant topics in Suresolv and is up-to-date.

BODMAS fraction questions
Decimal BODMAS questions