SSC CGL level Solution Set 73, Surds and Indices 7

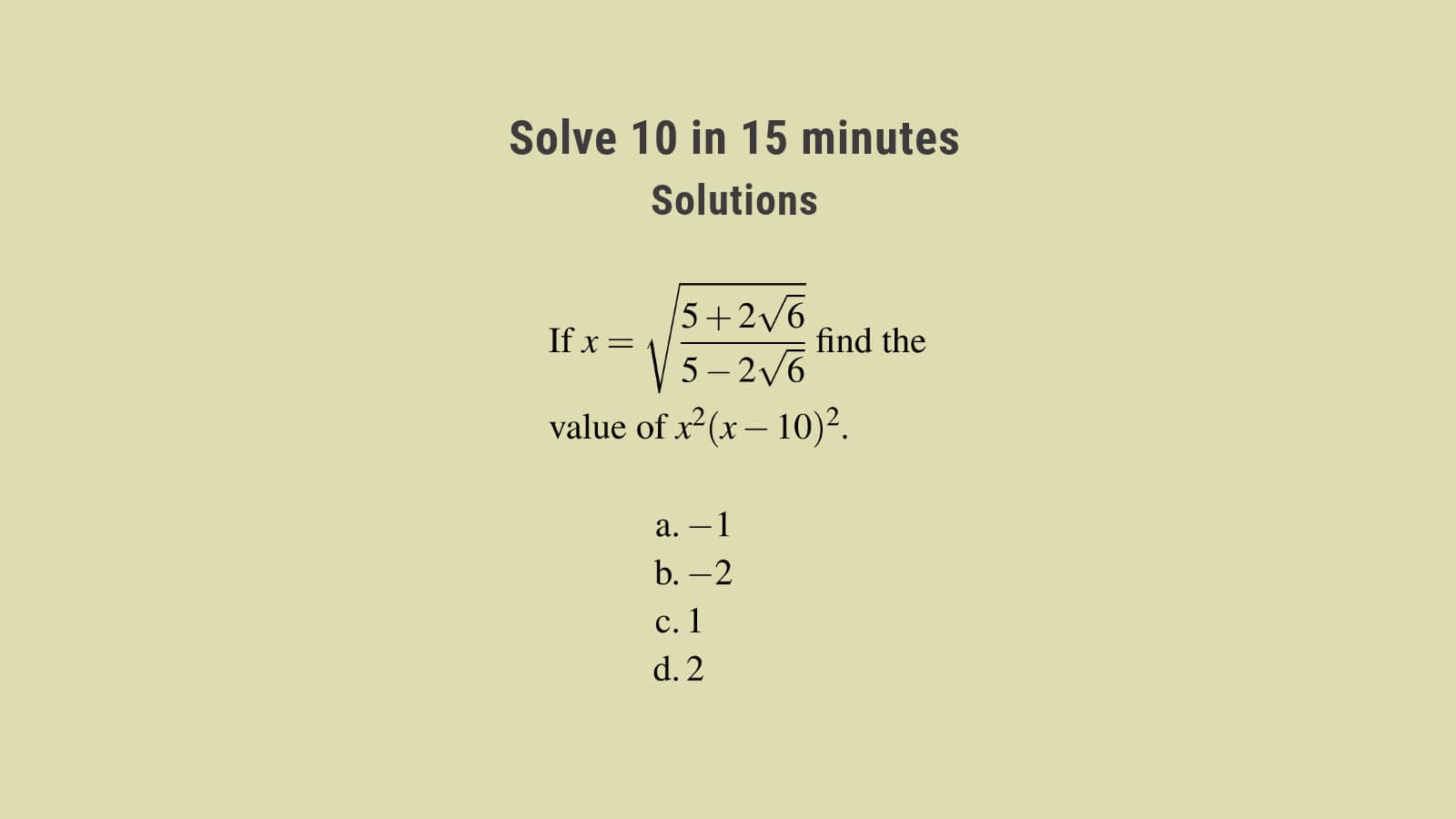
Solution to Surds and Indices questions for SSC CGL Set 73 - How to solve surds

From solution to surds and indices questions for SSC CGL 73, learn how to solve surds by rationalization of surds, surd term factoring and other methods.

For best results, take test from SSC CGL level Question Set 73, Surds and Indices 7 before going through the solutions.

For full confidence on how to solve surds and indices questions, use the question sets, solution sets and concept articles on surds and indices from the guide,

Guide to Fractions, Surds and Indices problem solving.

Solution to Surds and Indices questions for SSC CGL 73 - How to solve surds - answering time was 15 mins

Problem 1.

The value of $\displaystyle\frac{3\sqrt{2}-2\sqrt{3}}{3\sqrt{2}+2\sqrt{3}}+\displaystyle\frac{\sqrt{12}}{\sqrt{3}-\sqrt{2}}$ is,

1. $11$
2. $-12$
3. $12$
4. $-11$

Solution 1: Problem analysis and solving

Simplify the first term by taking common factor $\sqrt{6}$ out of both terms in numerator and denominator. The factor is cancelled out and the fraction term simplified greatly.

This is application of surd term factoring technique.

Simplify the numerator of the second term also by taking factor 2 out of square root.

Result of these two actions is,

$E=\displaystyle\frac{3\sqrt{2}-2\sqrt{3}}{3\sqrt{2}+2\sqrt{3}}+\displaystyle\frac{\sqrt{12}}{\sqrt{3}-\sqrt{2}}$

$=\displaystyle\frac{\sqrt{6}(\sqrt{3}-\sqrt{2})}{\sqrt{6}(\sqrt{3}+\sqrt{2})}+\displaystyle\frac{2\sqrt{3}}{\sqrt{3}-\sqrt{2}}$

$=\displaystyle\frac{\sqrt{3}-\sqrt{2}}{\sqrt{3}+\sqrt{2}}+\displaystyle\frac{2\sqrt{3}}{\sqrt{3}-\sqrt{2}}$.

The denominators are ready for applying rationalization of surds technique,

$E=(\sqrt{3}-\sqrt{2})^2+2\sqrt{3}(\sqrt{3}+\sqrt{2})$.

If you can spot the common factor of $\sqrt{6}$, you can reach this result quick.

Evaluate the products,

$E=3-2\sqrt{6}+2+6+2\sqrt{6}$

$=11$.

Simple at the end.

Answer: Option a: $11$.

Key concepts and techniques used:

1. Surd term factoring.
2. Rationalization of surds.
3. Surd arithmetic.
4. Question solved in mind.

Problem 2.

Value of $\displaystyle\frac{\sqrt{5}+\sqrt{3}}{\sqrt{80}+\sqrt{48}-\sqrt{45}-\sqrt{27}}$ is,

1. $-2$
2. $-1$
3. $2$
4. $1$

Solution 2: Problem analysis and solving

Identify the key pattern in the four surds in the denominator - each of the four surds has a factor that is a square, 16, 16, 9 and 9 respectively.

Apply surd term factoring by taking the squares out of the square roots,

$\displaystyle\frac{\sqrt{5}+\sqrt{3}}{\sqrt{80}+\sqrt{48}-\sqrt{45}-\sqrt{27}}$

$=\displaystyle\frac{\sqrt{5}+\sqrt{3}}{4\sqrt{5}+4\sqrt{3}-3\sqrt{5}-3\sqrt{3}}$

$=\displaystyle\frac{\sqrt{5}+\sqrt{3}}{\sqrt{5}+\sqrt{3}}$

$=1$.

The numerator and denominator cancel out.

Answer. Option d: $1$.

Key concepts and techniques used:

1. Key Pattern identification.
2. Surd term factoring.
3. Question solved in mind.
   

Problem 3.

Simplify $\displaystyle\frac{6}{2\sqrt{3}-\sqrt{6}}+\displaystyle\frac{\sqrt{6}}{\sqrt{3}+\sqrt{2}}-\displaystyle\frac{4\sqrt{3}}{\sqrt{6}-\sqrt{2}}$ is,

1. $1$
2. $2$
3. $0$
4. $-1$

Solution 3: Problem analysis and solving

In such a problem with multiple surd fractions, we always look for two patterns or opportunities,

1. Surd term factoring, and,
2. Rationalization of surds.

With such directed attention, the first term could easily be simplified by first factoring $\sqrt{6}$ out of first term of the denominator,

$2\sqrt{3}=\sqrt{6}\times{\sqrt{2}}$,

And then factoring $\sqrt{6}$ out of the whole denominator and canceling it out with the numerator,

$\displaystyle\frac{6}{2\sqrt{3}-\sqrt{6}}$

$=\displaystyle\frac{\sqrt{6}}{\sqrt{2}-1}$

$=\sqrt{6}(\sqrt{2}+1)$, rationalization by multiplying and dividing the term with $(\sqrt{2}+1)$.

This is Surd term factoring and Rationalization of surds together.

Second term is rationalized by multiplying and dividing it with $\sqrt{3}-\sqrt{2}$. Result is,

$\displaystyle\frac{\sqrt{6}}{\sqrt{3}+\sqrt{2}}$

$=\sqrt{6}(\sqrt{3}-\sqrt{2})$.

Surd term factoring could again be applied on the third term by factoring out $\sqrt{2}$ from the numerator and both terms of the denominator. It cancels out. Result is,

$\displaystyle\frac{4\sqrt{3}}{\sqrt{6}-\sqrt{2}}$

$=\displaystyle\frac{2\sqrt{6}}{\sqrt{3}-1}$

$=\sqrt{6}(\sqrt{3}+1)$, by Rationalization of surds.

Add the three terms and take common factor $\sqrt{6}$,

$E=\sqrt{6}(\sqrt{2}+1+\sqrt{3}-\sqrt{2}-\sqrt{3}-1)=0$.

By surd term factoring surd factors cancelled out between the numerators and denominators.

Strategy of simplifying surds denominators first and then rationalize.

Answer: Option c: $0$.

Key concepts and techniques used:

1. Surd term factoring
2. Denominator simplification first.
3. Rationalization of surds.
4. Question solved in mind.

Problem 4.

Simplify $\displaystyle\frac{4\sqrt{18}}{\sqrt{12}}-\displaystyle\frac{8\sqrt{75}}{\sqrt{32}}+\displaystyle\frac{9\sqrt{2}}{\sqrt{3}}$.

1. $2$
2. $0$
3. $-1$
4. $1$

Solution 4: Problem analysis and solving

Applying surd term factoring on all the terms,

$\displaystyle\frac{4\sqrt{18}}{\sqrt{12}}-\displaystyle\frac{8\sqrt{75}}{\sqrt{32}}+\displaystyle\frac{9\sqrt{2}}{\sqrt{3}}$

$=\displaystyle\frac{12\sqrt{2}}{2\sqrt{3}}-\displaystyle\frac{40\sqrt{3}}{4\sqrt{2}}+\displaystyle\frac{9\sqrt{2}}{\sqrt{3}}$

$=2\sqrt{6}-5\sqrt{6}+3\sqrt{6}$,

$=0$.

Answer: Option b: $0$.

In the last step, integer coefficients in the three numerators 6, 10 and 9 are split into factors of surds,

$6=2\sqrt{3}\times{\sqrt{3}}$,

$10=5\sqrt{2}\times{\sqrt{2}}$, and,

$9=3\sqrt{3}\times{\sqrt{3}}$.

This is reverse surd term factoring.

Key concepts and techniques used:

1. Fraction arithmetic
2. Surd term factoring
3. Reverse surd term factoring
4. Question solved in mind.

Reverse surd term factoring

An example of reverse surd term factoring,

$9\sqrt{2}=3(\sqrt{3})^2\sqrt{2}=3\sqrt{3}\sqrt{6}$.

Objective: To extract a surd factor from an integer to cancel out the surd term in denominator.

Problem 5.

Value of

$\displaystyle\frac{1}{1+\sqrt{2}}+\displaystyle\frac{1}{\sqrt{2}+\sqrt{3}}+\displaystyle\frac{1}{\sqrt{3}+\sqrt{4}}$

$+\displaystyle\frac{1}{\sqrt{4}+\sqrt{5}}+\displaystyle\frac{1}{\sqrt{5}+\sqrt{6}}+\displaystyle\frac{1}{\sqrt{6}+\sqrt{7}}$

$+\displaystyle\frac{1}{\sqrt{7}+\sqrt{8}}+\displaystyle\frac{1}{\sqrt{8}+\sqrt{9}}$ is,

1. $0$
2. $-2$
3. $2$
4. $1$

Solution 5: Problem analysis and solving execution

Two key patterns identified are,

• Difference between the surd term values under square roots in each denominator is 1 (this will result in unity denominator when rationalized), and
• Adjacent denominators have a common surd term.

Rationalize the denominators.

It results in canceling out all the intermediate surd terms. One term from the first pair and one from the last pair are left.

Result is,

$E=3-1=2$.

Answer: Option c: $2$.

Key concepts and techniques used:

1. Key Pattern identification
2. Rationalization of surds
3. Question solved in mind.
   

Let us show the deductive steps.

Rationalize the denominators and place the larger term in each pair first in the subtractive numerator expressions,

$E=(\sqrt{2}-1)+(\sqrt{3}-\sqrt{2})+(\sqrt{4}-\sqrt{3})$

$+(\sqrt{5}-\sqrt{4})+(\sqrt{6}-\sqrt{5})+(\sqrt{7}-\sqrt{6})$

$+(\sqrt{8}-\sqrt{7})+(\sqrt{9}-\sqrt{8})$

$=\sqrt{9}-1$

$=2$.

Problem 6.

If $a=\displaystyle\frac{1}{3+2\sqrt{2}}$ and $b=\displaystyle\frac{1}{3-2\sqrt{2}}$, the value of $a^2b +ab^2$ is,

1. $-5$
2. $6$
3. $-6$
4. $5$

Solution 6: Problem analysis and solving execution

First we identify the two patterns,

$ab=\displaystyle\frac{1}{3+2\sqrt{2}}\times{\displaystyle\frac{1}{3-2\sqrt{2}}}$

$=\displaystyle\frac{1}{9-8}$

$=1$, and,

$a+b=\displaystyle\frac{1}{3+2\sqrt{2}}+\displaystyle\frac{1}{3-2\sqrt{2}}$

$=6$, the denominator is simplified to 1, and the surd terms cancel out in the numerator.

Turning our attention to the target expression the third pattern is identified as,

$a^2b+ab^2=ab(a+b)$.

So the answer turns out to be 6.

Answer: Option b: $6$.

Key concepts and techniques used: 

1. Key pattern identification
2. Surd simplification
3. Question solved in mind.

Done easily in mind. Quick solution depended on the similar pattern identification and use.

Problem 7.

If $x=\displaystyle\frac{\sqrt{3}-\sqrt{2}}{\sqrt{3}+\sqrt{2}}$ and $y=\displaystyle\frac{\sqrt{3}+\sqrt{2}}{\sqrt{3}-\sqrt{2}}$, the value of $x^3+y^3$ is,

1. 807
2. 907
3. 870
4. 970

Solution 7: Problem analysis and solving execution

The patterns identified in the given expressions are simple value of $xy$ and $x+y$,

$xy=1$, and 

$x+y=(\sqrt{3}-\sqrt{2})^2+(\sqrt{3}+\sqrt{2})^2$

$=10$.

Knowing that the sum of cubes expands to,

$x^3+y^3=(x+y)(x^2-xy+y^2)$,

we express it in terms of $xy$ and $x+y$,

$x^3+y^3=(x+y)\left[(x+y)^2-3xy\right]$

$=10\times{(100-3)}$

$=970$.

Answer: Option d: 970.

Key concepts and techniques used: 

1. Key pattern identification
2. Input driven target transformation
3. Surd algebra.
4. Question solved in mind.

Problem 8.

If $x=\sqrt{\displaystyle\frac{5+2\sqrt{6}}{5-2\sqrt{6}}}$ find the value of $x^2(x-10)^2$,

1. $-1$
2. $-2$
3. $1$
4. $2$

Solution 8: Problem analysis and solution by double square root surd simplification

The value expression of $x$ has double square root surd that may need simplification.

But on second look, identify the key opportunity of transforming the surd expression inside the square root into a square of sum of surds.

Rationalize the denominator inside the square root by multiplying and dividing with $(5+2\sqrt{6})$,

$x=\sqrt{\displaystyle\frac{5+2\sqrt{6}}{5-2\sqrt{6}}\times{\displaystyle\frac{5+2\sqrt{6}}{5+2\sqrt{6}}}}$

$=\sqrt{\displaystyle\frac{(5+2\sqrt{6})^2}{5^2-4\times{6}}}$

$=5+2\sqrt{6}$.

The patterns of $5^2-2^2\times{(\sqrt{6})^2}=1$ and $(5+2\sqrt{6})^2$ in the numerator help to identify the opportunity.

Now it is time to evaluate the target expression with this simplified value of $x$.

Again identify the second opportunity when evaluating $(x-10)$,

$x-10=5+2\sqrt{6}-10=-(5-2\sqrt{6})$.

Lastly, identify and use the third opportunity of transforming the product of two squared factors as a square of product of two factors,

$x^2(x-10)^2=[x(x-10)]^2$

$=[-(5+2\sqrt{6})(5-2\sqrt{6})]^2$

$=[-1]^2$

$=1$.

It is a cleverly constructed difficult surds question that you should solve easily in mind if you identify and use the three opportunities.

Answer: Option c: $1$.

Key concepts and techniques used:

1. Problem analysis.
2. Key opportunity identification in three stages to use the property of difference of squares of $5$ and $2\sqrt{6}$ as 1.
3. Rationalization of surds.
4. Question solved in mind.
   

Problem 9.

Arrange $3^{34}$, $2^{51}$, and $7^{17}$ in descending order,

1. $3^{34} \gt 2^{51} \gt 7^{17}$
2. $2^{51} \gt 3^{34} \gt 7^{17}$
3. $3^{34} \gt 7^{17} \gt 2^{51}$
4. $7^{17} \gt 2^{51} \gt 3^{34}$

Problem analysis and solving execution by base equalization

Identify the pattern that all three powers are multiples of 17,

$34=2\times{17}$

$51=3\times{17}$.

To compare the three values then, their powers are to be equalized to 17.

How to do it?

Move down the factors of 17 in the power on to the bases.

$2^{51}=(2^3)^{17}=8^{17}$

$3^{34}=(3^2)^{17}=9^{17}$.

The third one is, $7^{17}$.

Base equalization technique is applied here to make all the powers equal and power bases transformed.

Instead of the power bases, the powers themselves are equalized.

To compare the three terms, the indices comparison rule used is,

If $a \gt b$, then $a^x > b^x$.

Power 17 being the same, $3^{34}$ is the largest of the three as its power base 9 is the largest.

Second highest is $2^{51}$ as its power base 8 is second largest.

Automatically, $7^{17}$ becomes the smallest of the three.

The three values arranged in descending order of decreasing values is,

$3^{34} \gt 2^{51} \gt 7^{17}$.

Answer. Option a: $3^{34} \gt 2^{51} \gt 7^{17}$.

Key concepts and techniques used:

1. Key Pattern identification.
2. Indices rule: If $a^x=b^x$, then $a=b$.
3. Base equalization technique, in this case powera are equalized.
4. Question solved in mind.

Problem 10.

$4^{61}+4^{62}+4^{63}+4^{64}$ is divisible by,

1. $3$
2. $11$
3. $13$
4. $17$

Solution 10: Problem analysis and solving

Taking out $4^{61}$ as the common factor the given expression turns to,

$4^{61}+4^{62}+4^{63}+4^{64}$

$=4^{61}(1+4+4^2+4^3)$

$=4^{61}(85)$, divisible by 17.

Answer: Option d: 17.

Key concepts and techniques used:

1. Key pattern identification.
2. Factoring out term with large power to get a simple factor that is easy to evaluate.
3. Factorization.
4. Question solved in mind.

---

Guided help on Fractions, Surds and Indices in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on fractions, surds and indices in Suresolv, follow the guide,

Suresolv Fractions, Surds and Indices Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II and Other Competitive exams.

The guide list of articles includes ALL articles on Fractions, Surds and Indices​ and relevant topics in Suresolv and is up-to-date.

---