SSC CGL level Solution Set 82, Trigonometry 8

Quick solution to trigonometry questions for SSC CGL Set 82

Trigonometry for competitive exams: SSC CGL Solution Set 82 explains to you how to solve hard trigonometry questions quickly by trigonometry techniques.

For best results take the test first at,

SSC CGL Question set 82: Trigonometry for competitive exams.

---

Solutions to trigonometry for competitive exams SSC CGL Set 82 - testing time was 15 mins

Problem 1.

If $(1+\tan^2 \theta)=\displaystyle\frac{625}{49}$, then the value of $(\sqrt{\sin \theta +\cos \theta})$, where $\theta$ is acute, is,

1. $\displaystyle\frac{5}{7}$
2. $\displaystyle\frac{\sqrt{31}}{5}$
3. $1$
4. $\displaystyle\frac{5}{4}\sqrt{\displaystyle\frac{31}{42}}$

Solution 1: Problem analysis and execution

From given expression we'll get value of $\sec \theta=\displaystyle\frac{25}{7}$ first without any delay.

So, $\cos \theta=\displaystyle\frac{7}{25}$, (no negative value as $\theta$ is acute), and

$\sin \theta=\sqrt{1-\cos^2 \theta}=\sqrt{1-\displaystyle\frac{49}{625}}=\displaystyle\frac{24}{25}$,

Thus the target expression,

$\sqrt{\sin \theta +\cos \theta}$

$=\sqrt{\displaystyle\frac{24+7}{25}}$

$=\displaystyle\frac{\sqrt{31}}{5}$.

Answer: Option b: $\displaystyle\frac{\sqrt{31}}{5}$.

Key concepts used: Basic trigonometry concepts -- Use of $\sin^2 \theta + \cos^2 \theta=1$ -- Solving in mind.

The problem could easily be solved in mind.

Problem 2.

If $2\text{cosec }^2 \theta=x$, then the value of $x$ is,

1. $\displaystyle\frac{\sec \theta}{\sec \theta -1}+\displaystyle\frac{\sec \theta}{\sec \theta+1}$
2. $\displaystyle\frac{\text{cosec } \theta}{\text{cosec } \theta-1}+\displaystyle\frac{\text{cosec } \theta}{\text{cosec } \theta+1}$
3. $\displaystyle\frac{\text{cosec } \theta}{\sec \theta-1}+\displaystyle\frac{\text{cosec } \theta}{\sec \theta+1}$
4. $\displaystyle\frac{\sec \theta}{\text{cosec } \theta-1}+\displaystyle\frac{\sec \theta}{\text{cosec } \theta+1}$

Solution 2: Solving by mathematical reasoning on choice values

Denominators of the four choices would either be $\tan^2 \theta$ inverted in numerator to $\text{cot}^2 \theta$ or $\text{cot}^2 \theta$ inverted in numerator to $\tan^2 \theta$. In the latter case, the $\sin^2 \theta$ in the numerator of $\tan^2 \theta$ cannot be neutralized to the ultimate target of $\text{cosec}^2 \theta$. So, one of the choices 'a' or 'c' would be the answer.

With $\text{cot}^2 \theta$ in the numerator derived from denominator combining of $(\sec \theta-1)(\sec \theta+1)=\tan^2 \theta$, the $\cos^2 \theta$ in the numerator can only be neutralized by $\sec^2 \theta$ leaving $\text{cosec}^2 \theta$.

So the first choice is the answer.

Solution 2: Deduction from choice 'a' to given expression: Just for checking

Expression in choice 'a',

$\displaystyle\frac{\sec \theta}{\sec \theta -1}+\displaystyle\frac{\sec \theta}{\sec \theta+1}$

$=\sec \theta\left[\displaystyle\frac{2\sec \theta}{(\sec \theta-1)(\sec \theta+1)}\right]$

$=\displaystyle\frac{2\sec^2 \theta}{\sec^2 \theta-1}$

$=\displaystyle\frac{2\sec^2 \theta}{\tan^2 \theta}$

$=(2\sec^2 \theta)(\text{cot}^2 \theta)$

$=2\text{cosec}^2 \theta$.

Answer: Option a: $\displaystyle\frac{\sec \theta}{\sec \theta -1}+\displaystyle\frac{\sec \theta}{\sec \theta+1}$.

Key concepts used: Mathematical reasoning -- Choice value test -- Solving in mind.

With mathematical reasoning followed by checking, this problem could easily be solved in mind in a few tens of seconds.

Problem 3.

If $\cos \left(\displaystyle\frac{\theta}{2}\right)=x$ then the value of $x$ is,

1. $\sqrt{\displaystyle\frac{1+\cos \theta}{2}}$
2. $\sqrt{\displaystyle\frac{1-\sin \theta}{2}}$
3. $\sqrt{\displaystyle\frac{1-\cos \theta}{2}}$
4. $\sqrt{\displaystyle\frac{1+\sin \theta}{2}}$

Solution 3: Problem analysis, pattern identification and compound angles

The need is to use expanded expression for $\sin 2\alpha$ and $\cos 2\alpha$. You may remember just these two, but it is much better to know the relations for $\sin (\alpha+\beta)$ and $\cos (\alpha +\beta)$. Deriving varieties of compound angle expressions as well as multiple and submultiple angle relations becomes possible then with these two relations.

$\sin (\alpha+\beta)=\sin \alpha\cos \beta+\cos \alpha\sin \beta$,

So that, $\sin 2\alpha=2\sin \alpha\cos \alpha$.

Similarly,

$\cos (\alpha+\beta)=\cos \alpha\cos \beta-\sin \alpha\sin \beta$,

So that,

$\cos 2\alpha=\cos^2 \alpha -\sin^2 \alpha$.

In this problem, $\alpha=\displaystyle\frac{\theta}{2}$.

Analyzing the problem we decide firmly that expression for $\cos 2\alpha$ will give us solution as it involves $\cos^2 \alpha$ and $\sin^2 \alpha$ which can be converted to $\cos^2 \alpha$.

Our goal is to transform the right choice expression in terms of $\cos \alpha=\cos \left(\displaystyle\frac{\theta}{2}\right)=x$ and solve for $x$.

Solution 3: Problem Solving execution

Using the expanded expression for $\cos 2\alpha$,

$\cos 2\alpha=\cos^2 \alpha -\sin^2 \alpha=2\cos^2 \alpha -1$,

Or, $cos^2 \alpha=\displaystyle\frac{1+\cos 2\alpha}{2}$,

Or, $\cos \alpha=\sqrt{\displaystyle\frac{1+\cos 2\alpha}{2}}$

Or, $x=\cos \left(\displaystyle\frac{\theta}{2}\right)=\sqrt{\displaystyle\frac{1+\cos \theta}{2}}$.

Answer: Option a: $\sqrt{\displaystyle\frac{1+\cos \theta}{2}}$.

Key concepts used: Problem analysis -- Key pattern identification -- Trigonometric compound angles -- Trigonometric multiple and submultiple angles -- Mathematical reasoning --  Solving in mind.

The problem could easily be solved mentally in a few tens of seconds.

Problem 4.

If $x$ and $y$ are positive acute angles such that $\sin (2x+3y)=\displaystyle\frac{\sqrt{3}}{2}$ and $\cos (4x-3y)=\displaystyle\frac{\sqrt{3}}{2}$, then the value of $\tan (6x-3y)$ is,

1. $\displaystyle\frac{1}{\sqrt{3}}$
2. $0$
3. $\sqrt{3}$
4. $1$

Solution 4: Problem analysis and solution using trigonometric ratio values

Using the concept of trigonometric ratio values we have,

$\sin (2x+3y)=\displaystyle\frac{\sqrt{3}}{2}=\sin 60^0$,

Or, $(2x+3y)=60^0$, and

$\cos (4x-3y)=\displaystyle\frac{\sqrt{3}}{2}=\cos 30^0$,

Or, $(4x-3y)=30^0$.

Adding the two equations in $x$ and $y$ eliminates $y$ and we get,

$6x=90^0$,

Or, $2x=30^0$.

Substituting this value in the first equation we get,

$(2x+3y)=60^0$,

Or, $3y=30^0$.

So, $(6x-3y)=90^0-30^0=60^0$.

Again using trigonometric ratio value for $\tan 60^0=\sqrt{3}$, we get,

$\tan (6x-3y)=\tan 60^0=\sqrt{3}$.

Answer: Option c: $\sqrt{3}$.

Key concepts used: Trigonometric ratio values -- Linear equations in two variables -- Solving in mind.

This problem could also be solved in mind easily.

Problem 5.

The value of $\displaystyle\frac{\sec^3 \theta - \tan^3 \theta}{\sec \theta - \tan \theta}-2\tan^2 \theta-\sec \theta\tan \theta$ is,

1. $0$
2. $1$
3. $-1$
4. $2$

Solution 5: Problem analysis and execution: sum of cubes and trigonometric expression deduction

The numerator of the first term when expanded in its two factor product form of sum of cubes, the denominator is cancelled out,

$\displaystyle\frac{\sec^3 \theta - \tan^3 \theta}{\sec \theta - \tan \theta}$

$=\displaystyle\frac{(\sec \theta - \tan \theta)(\sec^2 \theta+\sec \theta\tan \theta+\tan^2 \theta)}{\sec \theta - \tan \theta}$

$=\sec^2 \theta +\sec \theta\tan \theta+\tan^2 \theta$

$=2\tan^2 \theta+\sec \theta\tan \theta+1$

First two terms are cancelled out against the second and third terms of the given expression, leaving 1 as the result.

Answer: Option b: $1$.

Key concepts used: Key pattern identification -- Sum of cubes -- $(\sec^2 \theta=1+\tan^2 \theta)$ -- Solving in mind.

Problem 6.

If $\text{cot } \alpha=\left(\displaystyle\frac{\sin \beta}{1-\cos \beta}\right)$, then the value of $\text{cot } 2\alpha$ is,

1. $\text{cot } \beta$
2. $\tan \beta$
3. $\text{cot } 2\beta$
4. $\text{cot } \displaystyle\frac{\beta}{2}$

Solution 6: Problem analysis: Key pattern identification: Denominator simplification

At the outset we identify the opportunity for simplifying the denominator of the RHS of the given equation,

$\text{cot } \alpha=\left(\displaystyle\frac{\sin \beta}{1-\cos \beta}\right)$

$=\left(\displaystyle\frac{\sin \beta(1+\cos \beta)}{1-\cos^2 \beta}\right)$

$=\displaystyle\frac{1+\cos \beta}{\sin \beta}$.

We have used the technique of denominator simplification by multiplying both numerator and denominator with $(1+\cos \beta)$ thereby reducing the denominator to a single term.

Our experience is,

Simplifying the denominator to a single term always simplifies subsequent processes.

This the key pattern we have used in this case.

Solution 6: Use of multiple angle function expressions for $\sin 2\alpha$ and $\cos 2\alpha$

Using multiple angle function expressions for $\sin 2\alpha$ and $\cos 2\alpha$,

$\text{cot }2\alpha=\displaystyle\frac{\cos 2\alpha}{\sin 2\alpha}$

$=\displaystyle\frac{\cos^2 \alpha-\sin^2 \alpha}{2\sin \alpha\cos \alpha}$

$=\displaystyle\frac{1}{2}\left(\text{cot }\alpha -\displaystyle\frac{1}{\text{cot }\alpha}\right)$

$=\displaystyle\frac{1}{2}\left(\displaystyle\frac{1+\cos \beta}{\sin \beta}-\displaystyle\frac{1-\cos \beta}{\sin \beta}\right)$

$=\displaystyle\frac{1}{2}(2\text{cot }\beta)$,

$=\text{cot }\beta$.

Answer: Option a: $\text{cot }\beta$.

Key concepts used: Problem analysis -- Key pattern identification -- Denominator simplification -- Multiple angle trigonometric functions -- Solving in mind.

Though we have detailed the numerous steps, with denominator simplification, it was easy to see the solution at the end without writing the steps.

Problem 7.

The simplified value of $\left(\displaystyle\frac{1}{\sec \theta+\tan \theta}\right)^2$ is,

1. $\sec \theta+\tan \theta$
2. $\sin \theta\cos \theta$
3. $\displaystyle\frac{1-\cos \theta}{1+\cos \theta}$
4. $\displaystyle\frac{1-\sin \theta}{1+\sin \theta}$

Solution 7: Problem analysis: Key pattern identification: Friendly trigonometric function pair: Solving in mind

We call $\sec \theta$, $\tan \theta$ as one of the three friendly trigonometric function pairs not only because of the frequently used simplifying relation, $(\sec^2 \theta=1+\tan^2 \theta)$, but more importantly because of the corollary inverse relation,

$\sec \theta +\tan \theta=\displaystyle\frac{1}{\sec \theta -\tan \theta}$.

Using the inverse relation for one of the two factors, $\displaystyle\frac{1}{\sec \theta+\tan \theta}$ in the given expression we transform it immediately to,

$\left(\displaystyle\frac{1}{\sec \theta+\tan \theta}\right)^2$

$=\displaystyle\frac{\sec \theta-\tan\theta}{\sec \theta+\tan \theta}$

$=\displaystyle\frac{1-\sin \theta}{1+\sin \theta}$, by multiplying numerator and denominator with $\cos \theta$.

It is a quick mental solution.

Answer: Option d: $\displaystyle\frac{1-\sin \theta}{1+\sin \theta}$.

Key concepts used: Key pattern identification -- Frinedly trigonometric function pair -- Solving in mind.

Problem 8.

The simplified value of $(\sec \theta-cos \theta)(\sec \theta+\cos \theta)$ is,

1. $2\tan^2 \theta$
2. $\sin^2 \theta+\tan^2 \theta$
3. $\sin^2 \theta\tan^2 \theta$
4. $2\sin^2 \theta$

Solution 8: Problem analysis and solving in mind

The given expression,

$(\sec \theta-cos \theta)(\sec \theta+\cos \theta)$

$=\sec^2 \theta-\cos^2 \theta$

$=1+\tan^2 \theta-(1-\sin^2 \theta)$

$=\sin^2 \theta+\tan^2 \theta$.

Answer: Option b: $\sin^2 \theta+\tan^2 \theta$.

Key concepts used: Basic trigonometric concepts -- Friendly trigonometric function pairs, $(sec^2 \theta=1+\tan^2\theta)$ and $(\cos^2 \theta=1-\sin^2 \theta)$.

Problem 9.

The simplified value of $\displaystyle\frac{\tan \theta}{1-\text{cot }\theta}+\displaystyle\frac{\text{cot } \theta}{1-\tan\theta}-\displaystyle\frac{2}{\sin \theta}$ is,

1. $-1$
2. $2$
3. $0$
4. $1$

Solution 9: Problem analysis and pattern identification

This is a three additive fraction term simplification problem. The first principle to be followed in such cases is,

When three or more additive fraction terms are to be combined for simplification, choose the two more suitable terms first and combine. At the next step combine the result of the first addition with the next term and so on.

Accordingly we choose the first two additive terms for combining,

$\displaystyle\frac{\tan \theta}{1-\text{cot }\theta}+\displaystyle\frac{\text{cot } \theta}{1-\tan\theta}$.

The first impulse in such a case always is to take a product of the denominators to form the new denominator and cross-multiply and add to form the new numerator. This generally lands you into a complicated time-consuming situation.

The best approach is,

To try for equalization of the two denominators, even if partially, by finding common patterns and transforming the two terms. This is key pattern identification combined with denominator equalization, a very powerful way  to solve problems quickly.

As we examined the two denominators, sure enough we found the pattern we were looking for,

$\displaystyle\frac{\tan \theta}{1-\text{cot }\theta}+\displaystyle\frac{\text{cot } \theta}{1-\tan\theta}$

$=\displaystyle\frac{\sin^2 \theta}{\cos \theta(\sin \theta-\cos \theta)}-\displaystyle\frac{\cos^2 \theta}{\sin \theta(\sin \theta-\cos\theta)}$

$=\displaystyle\frac{\sin^3 \theta-\cos^3 \theta}{\sin \theta\cos \theta(\sin \theta-\cos \theta)}$

$=\displaystyle\frac{\sin^2 \theta+\sin \theta\cos \theta+\cos^2 \theta}{\sin \theta\cos \theta}$, as $(x^3-y^3)=(x-y)(x^2+xy+y^2)$

$=\displaystyle\frac{1+\sin \theta\cos \theta}{\sin \theta\cos \theta}$, as $(\sin^2 \theta+\cos^2 \theta)=1$.

The third term also simplifies conveniently to,

$\displaystyle\frac{2}{\sin 2\theta}=\frac{1}{\sin \theta\cos \theta}$.

It is cancelled out leaving 1 as the final result.

Answer: Option d: $1$.

Key concepts used: Key pattern identification -- Denominator equalization -- Sum of cubes expression -- Basic trigonometry concepts -- Multiple angle trigonometric functions.

We didn't solve this problem wholly in mind and wrote down a few intermediate steps. For this solution, problem solving principles and requisite methods had to be used more than once.

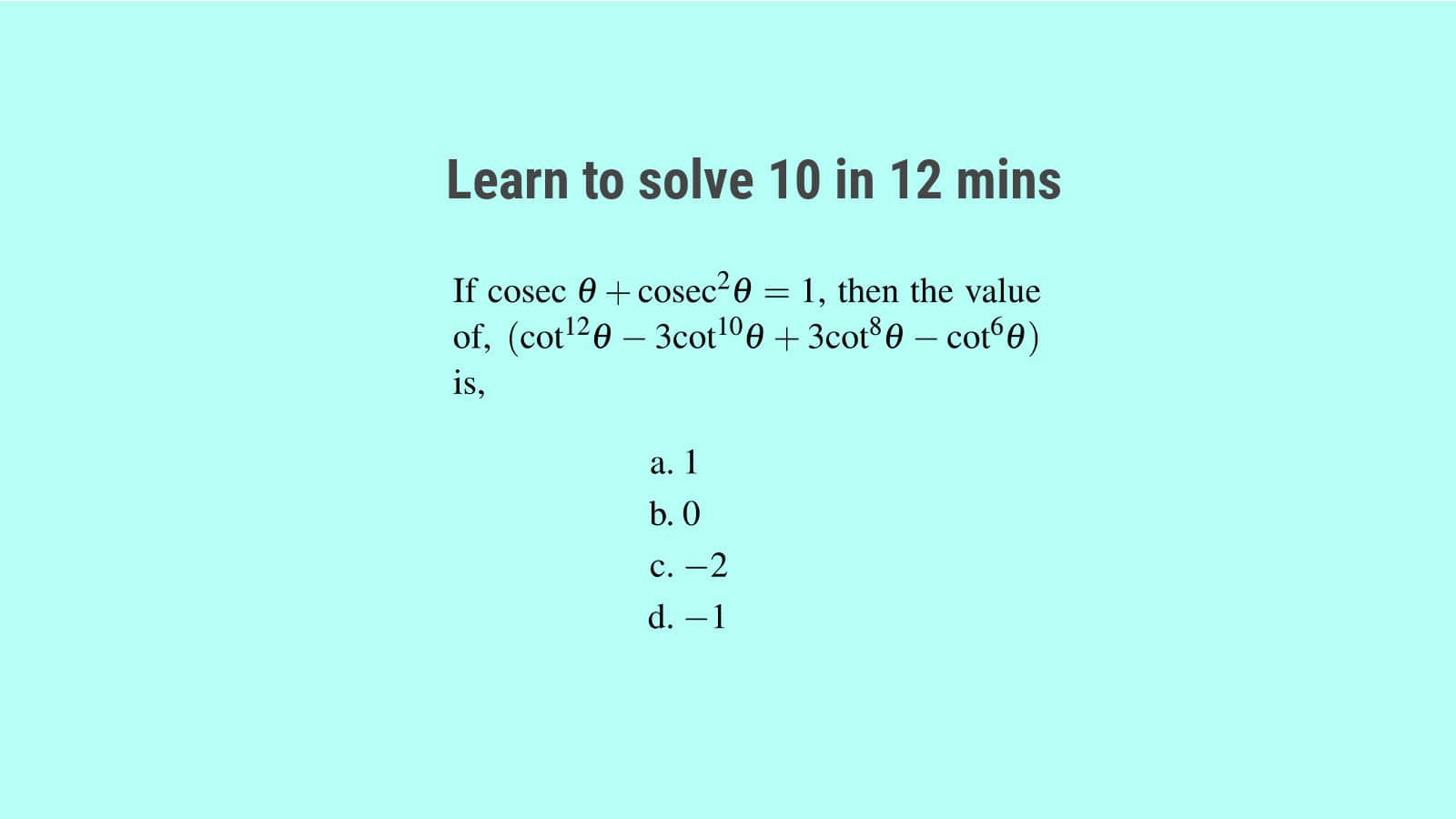
Problem 10.

If $\text{cosec }\theta+\text{cosec}^2 \theta=1$, then the value of, $(\text{cot}^{12} \theta-3\text{cot}^{10} \theta+3\text{cot}^{8} \theta-\text{cot}^{6} \theta)$ is,

1. $1$
2. $0$
3. $-2$
4. $-1$

Solution 10: Problem analysis, Strategy decision and pattern identification

Such a problem as the given one can usually be approached in two ways,

1. Either evaluate the value of $\text{cot } \theta$ from the given expression,
2. Or, Simplify the target expression and use the simple value of the expression on the LHS to achieve desired total simplification to a single numeric value.

We have a brief look at the given expression and decided that first approach is impracticable.

So we gave a closer look at the target expression and identified it as a cube of sum in powers of $\text{cot } \theta$. We felt sure, this must be the way to proceed. This is the point of key pattern identification.

Solution 10: Problem solving execution

The target expression,

$(\text{cot}^{12} \theta-3\text{cot}^{10} \theta+3\text{cot}^{8} \theta-\text{cot}^{6} \theta)$

$=(\text{cot}^4 \theta-\text{cot}^2 \theta)^3$

$=\left[(\text{cot}^2 \theta+\text{cot } \theta)(\text{cot}^2 \theta-\text{cot }\theta)\right]^3$

$=\left[(\text{cosec}^2 \theta-1+\text{cot } \theta)(\text{cosec}^2 \theta-1-\text{cot }\theta)\right]^3$

$=\left[(\text{cosec} \theta-\text{cot } \theta)(\text{cosec} \theta+\text{cot }\theta)\right]^3$, from given $\text{cosec }\theta+\text{cosec}^2 \theta=1$, $-\text{cosec }\theta=\text{cosec}^2 \theta-1$,

$=(\text{cosec}^2 \theta-\text{cot}^2 \theta)^3$

$=1$.

Answer: Option a: $1$.

Key concepts and techniques used: Problem analysis and key pattern identification -- Strategic problem solving approach -- Cube of sum expression -- Basic algebraic concepts -- Basic trigonometry concepts.

Note: Observe that in many of the Trigonometric problems basic and rich algebraic concepts and techniques are to be used. In fact that is the norm. Algebraic concepts are frequently used for elegant solutions of Trigonometric problems.

---

Guided help on Trigonometry in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Trigonometry in Suresolv, follow the guide,

Reading and Practice Guide on Trigonometry in Suresolv for SSC CHSL, SSC CGL, SSC CGL Tier II Other Competitive exams.

The guide list of articles is up-to-date.

---