
Difficult geometry questions for SSC CGL Solved question set 94
Solve 10 difficult geometry questions for SSC CGL in 15 minutes in Set 94. Verify correctness from answers and learn to solve quickly from solutions.
The solved question set contains,
- Difficult geometry questions for SSC CGL to be answered in 15 minutes (10 chosen questions)
- Answers to the questions, and,
- Quick solutions to the questions.
For best results, take the test first and then learn to solve quickly from solutions.
10 Difficult geometry questions for SSC CGL Set 94 - answering time 15 mins
Q1. In $\triangle ABC$, $\angle BAC=90^0$ and AD is drawn perpendicular to BC. If $BD=7$ cm and $CD=28$ cm, what is the length (in cm) of AD?
- $3.5$
- $10.5$
- $14$
- $7$
Q2. From an external point two tangents to a circle are drawn. The chord passing through the points of contact subtends an angle of $72^0$ at the centre. The angle between the tangents is,
- $108^0$
- $36^0$
- $144^0$
- $72^0$
Q3. The difference between the largest and the smallest angle of a triangle whose angles are in the ratio of $5:3:10$ is,
- $70^0$
- $50^0$
- $20^0$
- $30^0$
Q4. The length of a chord which is at a distance of 12 cm from the centre of circle of radius 13 cm is,
- $6$ cm
- $10$ cm
- $12$ cm
- $5$ cm
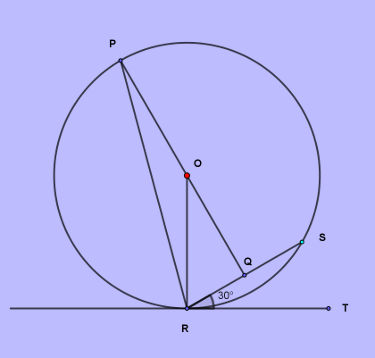
Q5. In the given figure, O is the centre of the circle, OQ is perpendicular to RS and $\angle SRT=30^0$. If $RS=10\sqrt{2}$ units, what is the value of $PR^2$?
- $100(3+2\sqrt{3})$ sq units
- $200(2+\sqrt{3})$ sq units
- $200(1+\sqrt{3})$ sq units
- $300(2+\sqrt{3})$ sq units
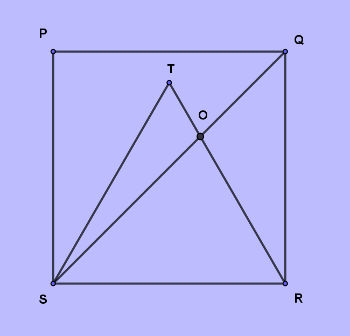
Q6. In the given figure, $PQRS$ is a square and $\triangle SRT$ is an equilateral triangle. What is the value (in degrees) of $\angle SOR$?
- $60$
- $45$
- $75$
- $55$
Q7. If PQ and PR be the two tangents to a circle with centre O such that $\angle QPR=120^0$ then $\angle POQ$ is,
- $60^0$
- $45^0$
- $90^0$
- $30^0$
Q8. AB is a chord of a circle with O as centre. C is a point on the circle such that $OC \perp AB$ and OC intersects AB at P. If $PC=2$ cm and $AB=6$ cm then the diameter of the circle is,
- $6$ cm
- $13$ cm
- $12$ cm
- $6.5$ cm
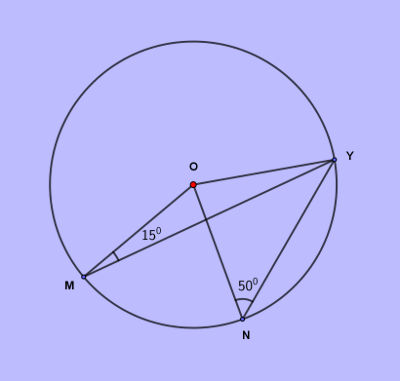
Q9. In the given figure, $\angle ONY=50^0$ and $\angle OMY=15^0$. Then the value of $\angle MON$ is,
- $20^0$
- $30^0$
- $70^0$
- $40^0$
Q10. In a right-angled $\triangle ABC$, $\angle ABC=90^0$; $BN$ is perpendicular to $AC$, $AB=6$ cm, $AC=10$ cm. Then $AN:NC$ is,
- $1:4$
- $3:16$
- $3:4$
- $9:16$
Answers to the 10 difficult geometry questions for SSC CGL Set 94
Q1. Answer: Option c: $14$.
Q2. Answer: Option a: $108^0$.
Q3. Answer: Option a: $70^0$.
Q4. Answer: Option b: $10$ cm.
Q5. Answer: Option b: $200(2+\sqrt{3})$ sq units.
Q6. Answer: Option c: $75$.
Q7. Answer: Option d: $30^0$.
Q8. Answer: Option d: $6.5$ cm.
Q9. Answer: Option c: $70^0$.
Q10. Answer: Option d: $9:16$.
Solutions to 10 difficult geometry questions for SSC CGL Set 94 - answering time was 15 mins
Q1. In $\triangle ABC$, $\angle BAC=90^0$ and AD is drawn perpendicular to BC. If $BD=7$ cm and $CD=28$ cm, what is the length (in cm) of AD?
- $3.5$
- $10.5$
- $14$
- $7$
Solution 1: Quick solution by draft drawing, use of Pythagoras theorem and by algebraic relations
First make a quick draft drawing depicting the problem,
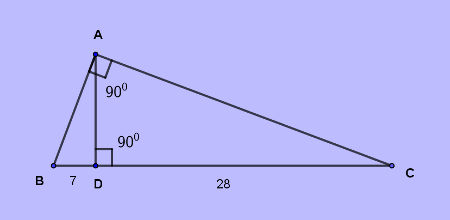
For convenience let's assume, $x=AB$ and $y=AC$.
By Pythagoras theorem, in right $\triangle ABC$ square of hypotenuse BC equals sum of squares of $x$ and $y$,
$x^2+y^2=35^2=(7+28)^2$.
In right $\triangle ABD$,
$AD^2+7^2=x^2$.
And in right $\triangle ACD$,
$AD^2+28^2=y^2$.
Add the two equations,
$2AD^2=(x^2+y^2)-(7^2+28^2)$
$=(7+28)^2-(7^2+28^2)=2\times{7}\times{28}$, as $(a+b)^2-(a^2+b^2)=2ab$
Or, $AD^2=7\times{28}=49\times{4}$.
So, $AD=7\times{2}=14$ cm.
Use of algebraic relations minimized computing time. This is a type of smart evaluation.
Answer: Option c: $14$.
Key concepts used: Draft drawing -- Pythagoras theorem -- Use of algebraic formula to speed up calculations -- Smart evaluation.
An alternate solution
The problem can be solved faster using trigonometric relations and complementary angle trigonometric functions, both belonging to the domain of trigonometry.
Assume $\angle ABC=\theta$.
So in right $\triangle ABD$, $\tan \theta =\displaystyle\frac{AD}{BD}$.
Similarly in right $\triangle ADC$, $\tan \angle ACD=\displaystyle\frac{AD}{CD}$.
As $\angle BAC=90^0$ in $\triangle ABC$,
$\angle ACD=180^0-90^0-\angle ABD=90^0-\angle ABD=90^0-\theta$.
By Complementary angle trigonometric function relations, for acute angle $\theta$,
$\tan (90^0-\theta)=\text{cot }\theta$.
So, $\tan \angle ACD=\text{cot }\theta=\displaystyle\frac{AD}{CD}$,
Or, $\tan \theta=\displaystyle\frac{CD}{AD}=\frac{AD}{BD}$,
Or, $AD^2=7\times{28}$,
Or, $AD=14$ cm.
Knowing the trigonometric concepts, this method is easier to use.
Answer: Option c: $14$.
Key concepts used: Key pattern identification that $\angle ABD$ and $\angle ACD$ are complementary angles and two $\tan$ functions with common height $AD$ can be directly related -- Solving in mind -- Use of many ways technique in solving the same problem in two different ways.
Note: If you try to solve problems in many different ways regularly, your general problem solving ability gets stronger.
Q2. From an external point two tangents to a circle are drawn. The chord passing through the points of contact subtends an angle of $72^0$ at the centre. The angle between the tangents is,
- $108^0$
- $36^0$
- $144^0$
- $72^0$
Solution 2: Quick solution by draft drawing and angle bisection property of two tangents to a circle from a common external point
Make a quick draft drawing for ease of solving the problem,
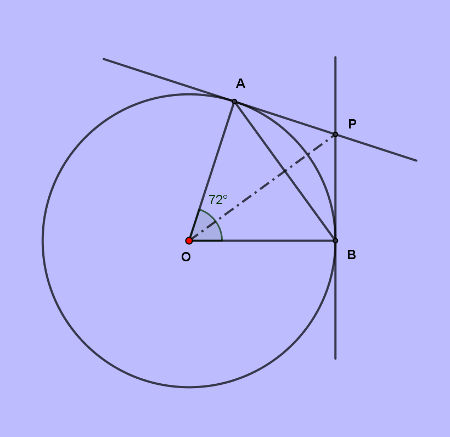
The two tangents to the circle with centre at O create a pair of congruent triangles $\triangle PAO$ and $\triangle PBO$.
Note: This is the basic property of two tangents from an external point. The reason why it is true is, in two right triangles $\triangle PAO$ and $\triangle PBO$, hypotenuse $PO$ common and second side radii $AO=BO$ making the third side also equal by Pythagoras theorem.
In two congruent triangles all three pairs of corresponding angles are equal.
So, $PO$ bisects both angles $\angle AOB$ and $\angle APB$.
It follows,
$\angle AOP=\displaystyle\frac{72^0}{2}=36^0$.
So, $\angle APO=180^0-90^0-36^0=54^0$.
And, $\angle APB=2\times{\angle APO}=108^0$.
Answer: Option a: $108^0$.
Key concepts used: Draft drawing (though not necessary, ease up solving) -- Angle bisection Property of two tangents to a circle from an external point -- Solving in mind.
An alternate faster solution
Without using the angle bisection property and using just the property that a tangent is perpendicular to the radius at tangent point, this problem can be solved faster.
In quadrilateral APBO, the two opposite angles $\angle PAO=\angle PBO=90^0$ so that sum of the other two opposite angles is also $180^0$,
$\angle AOB+\angle APB=180^0$,
Or, $\angle APB=180^0-72^0=108^0$.
This solution is immediate.
Key concepts used: Key pattern identification that two opposite angles in the quadrilateral are both $90^0$ so that the sum of other two opposite angles would also be $180^0$, this is a cyclic quadrilateral -- Quadrilateral angle properties -- Solving in mind -- Many ways technique.
Q3. The difference between the largest and the smallest angle of a triangle whose angles are in the ratio of $5:3:10$ is,
- $70^0$
- $50^0$
- $20^0$
- $30^0$
Solution 3: Immediate solution by HCF reintroduction ratio technique and Angles in triangle concept
Introduce the cancelled out HCF as the factor $x$ to each term of the ratio of angles to get the actual value of angles as,
$5x$, $3x$ and $10x$.
Total of angles,
$180^0=3x+5x+10x=18x$,
Or, $x=10^0$.
Difference between the largest and the smallest angle is,
$10x-3x=7x=70^0$.
Answer: Option a: $70^0$.
Key concepts used: Total of angles in a triangle $180^0$ -- HCF reintroduction technique in a ratio to get actual values -- Angles in triangle concept -- Solving in mind.
Q4. The length of a chord which is at a distance of 12 cm from the centre of circle of radius 13 cm is,
- $6$ cm
- $10$ cm
- $12$ cm
- $5$ cm
Solution 4: Immediate solution by visualization of a right triangle with distance 12 cm as the height, hypotenuse as radius 13 cm and base as half of chord length
Though not necessary the following draft drawing helps easy visualization,
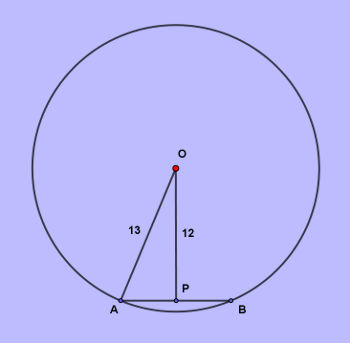
In right $\triangle OAP$, by Pythagoras theorem,
$AP^2=13^2-12^2=25$,
Or, $AP=5$.
By chord bisection property, a perpendicular from the centre of a circle to a chord bisects it.
So, OP bisected chord AB, and,
$AB=2AP=10$ cm.
Answer: Option b: $10$ cm.
Key concepts used: Visualization -- Pythagoras theorem -- Chord bisection by a perpendicular from centre of a circle -- Solving in mind.
Q5. In the given figure, O is the centre of the circle, OQ is perpendicular to RS and $\angle SRT=30^0$. If $RS=10\sqrt{2}$ units, what is the value of $PR^2$?

- $100(3+2\sqrt{3})$ sq units
- $200(2+\sqrt{3})$ sq units
- $200(1+\sqrt{3})$ sq units
- $300(2+\sqrt{3})$ sq units
Solution 5: Quick solution by using trigonometric ratios for selected angles, chord bisection property in a circle, Algebraic simplification, Surd term factoring and Smart evaluation
We'll add variable labels for smart evaluation in the given figure as, $x=OR$, and $y=OQ$,
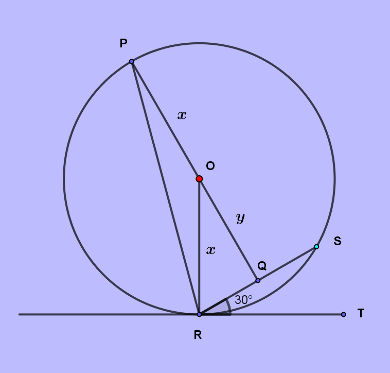
AS TR is tangent to the circle at point R where OR is the radius of the circle, OR is perpendicular to TR by tangent property.
It follows, $\angle ORS=90^0-\angle SRT=90^0-30^0=60^0$.
In $\triangle ORQ$, trigonometric ratio,
$\tan 60^0=\sqrt{3}=\displaystyle\frac{OQ}{QR}=\frac{y}{QR}$.
Again by chord bisection property in a circle, any chord is bisected by a line segment passing through the centre and perpendicular to the chord.
So, OQ being perpendicular to SR, it bisects SR at Q, that is,
$QR=5\sqrt{2}$ units.
Substituting,
$\displaystyle\frac{y}{QR}=\frac{y}{5\sqrt{2}}=\sqrt{3}$,
Or, $y=5\sqrt{6}$ units.
Similarly,
$\cos 60^0=\displaystyle\frac{1}{2}=\frac{QR}{x}$,
Or, $x=10\sqrt{2}$ units.
As OR and OP both are radii,
$OP=OR=x=10\sqrt{2}$ units.
And in right $\triangle PQR$, square of hypotenuse,
$PR^2=(OP+OQ)^2+QR^2$
$=(x+y)^2+(x^2-y^2)$, In right $\triangle ORQ$, $QR^2=x^2-y^2$,
$=2x^2+2xy$, $y^2$ cancels out
$=2x(x+y)$
$=20\sqrt{2}(10\sqrt{2}+5\sqrt{6})$
$=200(2+\sqrt{3})$ units, factoring out surd $5\sqrt{2}$.
Algebraic manipulation along with surd term factoring bypasses arithmetic calculations speeding up the solution process. This is called smart evaluation.
Answer: Option b: $200(2+\sqrt{3})$ sq units.
Key concepts used: Trigonometric ratios for selected angles -- Chord bisection property in a circle -- Smart evaluation -- Algebraic simplification -- Surd term factoring.
Q6. In the given figure, $PQRS$ is a square and $\triangle SRT$ is an equilateral triangle. What is the value (in degrees) of $\angle SOR$?

- $60$
- $45$
- $75$
- $55$
Solution 6: Quick solution by angle properties of an equilateral triangle and a square
The following figure is used for explaining the solution,
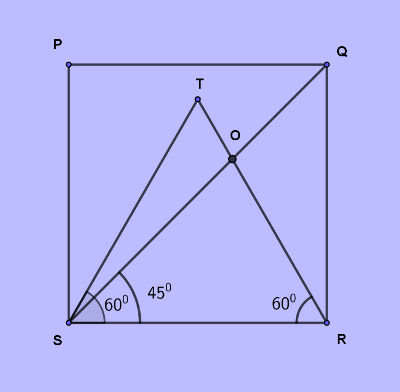
$\triangle SRT$ being an equilateral triangle, each of its internal angles equals $60^0$. So,
$\angle SRT=60^0$.
Again, each internal angle of a square is $90^0$ and a diagonal of the square bisects both the internal angles. So,
$\angle RSQ=\angle RSO=45^0$.
In $\triangle RSO$,
$\angle ROS=180^0-(45^0+60^0)=75^0$.
Answer: Option c: $75$.
Key concepts used: Angle properties of an equilateral triangle and a square -- Solving in mind.
Q7. If PQ and PR be the two tangents to a circle with centre O such that $\angle QPR=120^0$ then $\angle POQ$ is,
- $60^0$
- $45^0$
- $90^0$
- $30^0$
Solution 7: Immediate solution by basic tangent property and angle bisection property of two tangents to a circle from an external point
Though not necessary the following draft drawing helps accurate visualization,
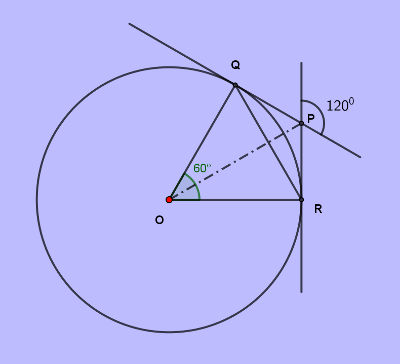
By basic tangent property opposite angles in quadrilateral PQOR,
$\angle PQO=\angle PRO=90^0$.
So,
$\angle QOR=180^0-\angle QPR=180^0-120^0=60^0$.
By angle bisection property of two tangents to a circle from an external point due to congruence of two triangles formed, line segment PO bisects both angles, $\angle QPR$ and $\angle QOR$.
So,
$\angle POQ=\frac{1}{2}\angle QOR=30^0$.
Answer: Option d: $30^0$.
Key concepts used: Basic tangent property -- Angle bisection property of two tangents to a circle from an external point -- Solving in mind.
Q8. AB is a chord of a circle with O as centre. C is a point on the circle such that OC is perpendicular to AB and OC intersects AB at P. If $PC=2$ cm and $AB=6$ cm then the diameter of the circle is,
- $6$ cm
- $13$ cm
- $12$ cm
- $6.5$ cm
Solution 8: Quick solution by chord bisection property, Pythagoras theorem and algebraic simplification
The following graphic will be used for explaining the solution,
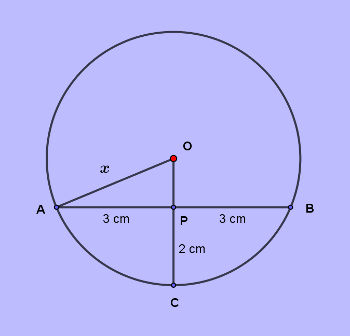
Given $PC=2$ cm, and chord $AB=6$ cm.
As OC, a radius, say of length $x$ is perpendicular to chord AB at P, it bisects the chord at P.
So, $AP=3$ cm.
In right $\triangle OAP$, by Pythagoras theorem,
$OA^2=AP^2+OP^2=3^2+(OC-PC)^2$,
Or, $x^2=9+(x-2)^2=9+x^2-4x+4$,
Or, $4x=13$,
Or, $\text{Diameter } 2x=\displaystyle\frac{13}{2}=6.5$ cm.
A bit of algebra helps to solve the problem in no time.
Answer: Option d: $6.5$ cm.
Key concepts used: Chord bisection property -- Pythagoras theorem -- Algebraic simplification -- Solving in mind.
Note: In Geometry, Trigonometry and Arithmetic problem solving, often algebraic simplification helps to solve the problem cleanly and quickly.
Q9. In the given figure, $\angle ONY=50^0$ and $\angle OMY=15^0$. Then the value of $\angle MON$ is,

- $20^0$
- $30^0$
- $70^0$
- $40^0$
Solution 9: Immediate solution by key pattern identification of two isosceles triangles
Easiest solution is to identify first the $\triangle ONY$ to be an isosceles triangle with radii $ON=OY$, so that,
$\angle OYN=\angle ONY=50^0$.
The third angle in the triangle evaluates to,
$\angle NOY=180^0-(50^0+50^0)=80^0$.
Identify the second key pattern that the $\triangle OMY$ is isosceles as radii, $OM=OY$.
So,
$\angle OYM=\angle OMY=15^0$.
The third angle in the $\triangle OMY$,
$\angle MOY=180^0-(15^0+15^0)=150^0$.
Then, $\angle MON=\angle MOY-\angle NOY=150^0-80^0=70^0$.
Answer: Option c: $70^0$.
Key concepts used: Key pattern identification of two useful isosceles triangles and angle property of triangles -- Solving in mind.
Q10. In a right-angled $\triangle ABC$, $\angle ABC=90^0$; $BN$ is perpendicular to $AC$, $AB=6$ cm, $AC=10$ cm. Then $AN:NC$ is,
- $1:4$
- $3:16$
- $3:4$
- $9:16$
Solution 10: Quick solution by identifying two suitable similar triangles and side ratio equality property of two similar triangles
The following graphic describes the problem.
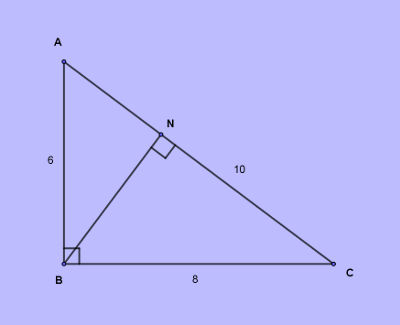
Before any action, first step is to evaluate the length of the third side BC by Pythagoras theorem,
$BC=\sqrt{10^2-6^2}=\sqrt{64}=8$ cm.
Now identify two suitable similar triangles.
The right $\triangle ABC$ and $\triangle BNC$ are similar as,
$\angle C$ is common, and,
$\angle ABC=\angle BNC$ so that the third angles are also equal,
$\angle NBC=\angle BAC$.
By the property of two similar triangles, the ratio of corresponding sides are equal,
$\displaystyle\frac{NC}{BC}=\frac{BC}{AC}$.
In LHS of the ratio, first pair of sides of $\triangle NBC$ in numerator and of $\triangle ABC$ in denominator are opposite to angles $\angle NBC=\angle BAC$.
In RHS of the ratio, second pair of sides of $\triangle NBC$ and $\triangle ABC$ respectively are opposite to right angles,
$\angle BNC=\angle ABC$. Numerators are from $\triangle NBC$ and denominator from $\triangle ABC$.
This is how in a similar triangle, corresponding pairs of sides are equated (primarily identified as sides opposite to angles that are equal).
So,
$AC\times{NC}=BC^2=64$,
Or, $NC=\displaystyle\frac{64}{10}=6.4$, and,
$AN=AC-NC=10-6.4=3.6$.
Ratio, $AN:NC=3.6:6.4=9:16$.
Answer: Option d: $9:16$.
Key concepts used: Pythagoras theorem -- Key pattern identification of two similar triangles -- Side ratio equality of corresponding sides of two similar triangles -- Ratio concepts -- Solving in mind.
End note
Observe that, each of the problems could be quickly and cleanly solved in minimum number of steps using special key patterns and methods in each case.
This is the hallmark of quick problem solving:
- Concept based pattern and method formation, and,
- Identification of the key pattern and use of the method associated with it. Every special pattern has its own method, and not many such patterns are there.
Important is the concept based pattern identification and use of quick problem solving method.
Recommendation: In Geometry problem solving, first represent the problem figure quickly as a draft figure. It need not be perfect at all. Then analyze the problem to identify the useful patterns and apply suitable methods. Identify what element value is required to be known for the final answer, and then work backwards.
If necessary, introduce new elements such as an angle, a side or a line segment. Or, if you find it faster, use trigonometric, algebraic or arithmetic concepts such ratios without any hesitation. Your goal is to arrive at the correct answer quickly and accurately.
And one more important point to remember, you must not depend on guesswork in this math exam.
Keep using the basic concepts and use the advanced concepts or rules only when you require it.
Guided help on Geometry in Suresolv
To get the best results out of the extensive range of articles of tutorials, questions and solutions on Geometry in Suresolv, follow the guide,
The guide list of articles includes ALL articles on Geometry and relevant topics in Suresolv and is up-to-date.
