LCM HCF questions for SSC CHSL with Answers and Quick Solutions 1

Submitted by Atanu Chaudhuri on Sat, 18/01/2020 - 16:59

LCM HCF Questions for SSC CHSL with Answers and Quick Solutions 1

LCM and HCF Questions for SSC CHSL 1st Set: Answers and Quick Solutions

First set of LCM HCF questions for SSC CHSL with answers and quick solutions. Questions are on LCM, LCM of fractions, HCF by factorization and more.

These are previous year LCM HCF questions for SSC CHSL.

It contains,

Question set on LCF and HCF for SSC CHSL to be answered in 15 minutes (10 chosen questions)
Answers to the questions, and
Quick conceptual solutions to the questions.

For maximum gains, the test should be taken first, and then the solutions are to be referred to.

LCM HCF Questions for SSC CHSL exam 1st Set - answering time15 mins

Q1. The least number which when divided by 16, 18, 20 and 25 leaves 4 as remainder in each case but when divided by 7 leaves no remainder, is,

18002
18004
18000
17004

Q2. LCM of $\displaystyle\frac{2}{3}$, $\displaystyle\frac{4}{9}$ and $\displaystyle\frac{5}{6}$ is,

$\displaystyle\frac{8}{27}$
$\displaystyle\frac{20}{27}$
$\displaystyle\frac{20}{3}$
$\displaystyle\frac{10}{3}$

Q3. A milk vendor has 24 litres of cow milk, 42 litres of toned milk and 63 litres of double toned milk. If he wants to pack them in cans so that each can contains same litres of milk and does not want to mix any two kinds of milk in a can, then the least number of cans required is,

Q4. A farmer has 945 cows and 2475 sheep. He farms them in flocks, keeping cows and sheep separate and having same number of animals in each flock. If these flocks are as large as possible, then the maximum number of animals in each flock and total number of flocks required for the purpose respectively are,

45 and 76
15 and 228
46 and 75
9 and 380

Q5. There are 24 peaches, 36 apricots and 60 bananas and these have to be arranged in several rows in such a way that every row contains the same number of fruits of only one kind. What is the number of rows required to make this arrangement with maximum number of fruits in a row?

Q6. A number between 1000 and 2000 which when divided by 30, 36 and 80 gives a remainder 11 in each case is,

1523
1451
1712
1641

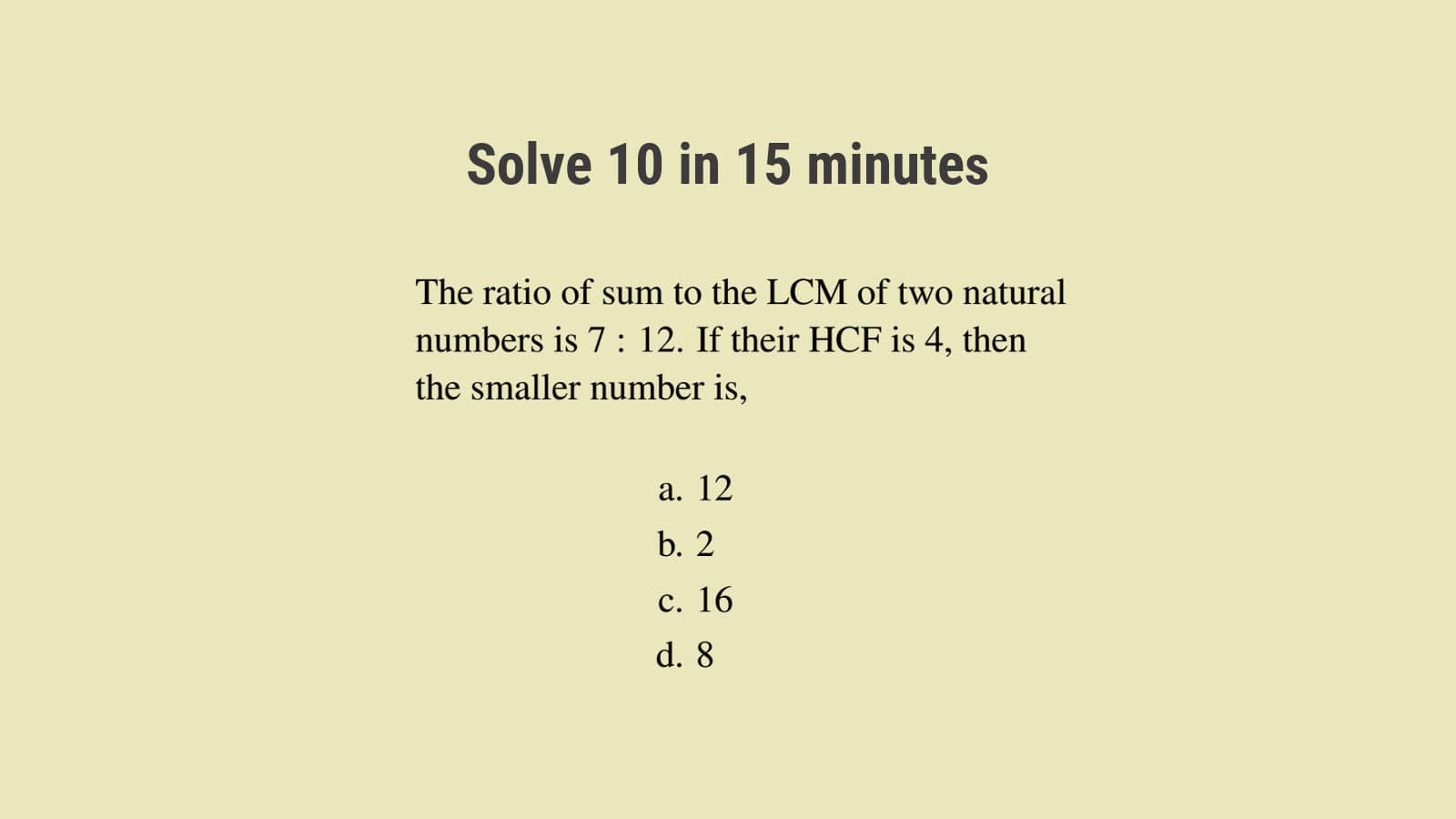
Q7. The ratio of sum to the LCM of two natural numbers is 7 : 12. If their HCF is 4, then the smaller number is,

Q8. The smallest 5 digit number which is divisible by 12, 18, and 21 is,

10224
30526
50321
10080

Q9. A fraction becomes $\displaystyle\frac{1}{6}$ when 4 is subtracted from its numerator and 1 is added to its denominator. If 2 and 1 are added to its numerator and denominator respectively, it becomes $\displaystyle\frac{1}{3}$. Then the LCM of the numerator and denominator of the said fraction must be,

Q10. If $A$ and $B$ are the HCF and LCM respectively of two algebraic expressions $x$ and $y$, and $A+B=x+y$, then value of $A^3+B^3$ is,

$x^3-y^3$
$x^3+y^3$
$y^3$
$x^3$

Answers to LCM HCF Questions for SSC CHSL exam 1st Set

Q1. Answer: Option b: 18004.

Q2. Answer: Option c : $\displaystyle\frac{20}{3}$.

Q3. Answer: Option b: 6.

Q4. Answer: Option a: 45 and 76.

Q5. Answer: Option d: 10.

Q6. Answer: Option b : 1451.

Q7. Answer: Option a: 12.

Q8. Answer: Option d: 10080.

Q9. Answer: Option a: 70.

Q10. Answer: Option b: $x^3+y^3$.

Quick Solutions to LCM HCF Questions for SSC CHSL exam 1st Set - answering time was 15 mins

Q1. The least number which when divided by 16, 18, 20 and 25 leaves 4 as remainder in each case but when divided by 7 leaves no remainder, is,

18002
18004
18000
17004

Solution 1: Problem analysis and solution by LCM, and Enumeration or Trial on choice values

The least such number will be a multiple of the LCM of the four numbers 16, 18, 20, 25 with 4 added to it.

The second condition that the desired number must satisfy is—it must be divisible by 7 also. The desired number will not then be the LCM plus 4 but much larger. This is so because LCM 3600 plus 4, that is, 3604 is not divisible by 7. We'll see this in details shortly.

To find the desired number, first step is to find the LCM, because the number has to be a multiple of LCM with 4 added to it.

It is easy to find the LCM mentally, but for ease of explanation we"ll show you the process of finding the LCM by factorization.

The prime factors of the given numbers 16, 18, 20 and 25 are,

$16=2\times{2}\times{2}\times{2}$

$18=2\times{3}\times{3}$

$20=2\times{2}\times{5}$

$25=5\times{5}$.

16 has 4 numbers of 2's. Include 16 as the first non-prime factor of LCM as the other three numbers have lesser number of 2's. Strike out all occurrences of 2's in the other numbers.

This result in Second number 18 contributing 2 numbers of 3's as unique prime factors, that is, 9 as second non-prime factor of LCM because 20 and 25 have no factor of 3 more than 2 in number.

At this intermediate stage, we have the partially formed LCM, $16\times{9}$ with the third and fourth numbers 20 and 25 yet to be considered.

With 16 already included in the partial LCM, 4 is struck out from 20 leaving 1 number of 5. As 25 contributes to 2 numbers of 5, just include 25 in the LCM to take care of 5 in 20.

Finally LCM of 16, 18, 20, 25 is,

$16\times{9}\times{25}=3600$.

You can now solve the problem in two ways.

Solution to Problem 1: By Enumeration

First enumeration: LCM multiplied with 1 and 4 added to it,

$3600+4=3604$.

Test for divisibility of 7. The number is not divisible by 7.

Second enumeration: LCM multiplied with 2 and 4 added to it,

$3600\times{2}+4=7204$, not divisible by 7.

Third enumeration: LCM multiplied by 3 and 4 added to it,

$3600\times{3}+4=10804$, not divisible by 7.

Fourth enumeration: LCM multiplied by 4 and 4 added to it,

$3600\times{4}+4=14404$, not divisible by 4.

Fifth enumeration: LCM multiplied by 5 and 4 added to it,

$3600\times{5}+4=18004$, divisible by 7 at last with quotient 2572.

18004 of option b is the answer.

This method is shown to make the mechanism of how this least number is formed clear to you.

In practice, the second method of trial on choice values is faster.

Solution to Problem 1: By trial on Choice values

In this method, we'll test the choice values.

You can carry out two tests on each choice value,

Subtract 4 from the choice value and test for divisibility by 3600, and/or
Test to see whether the choice value is divisible by 7.

The solution must satisfy both the conditions. So carry out any of the above two tests on each choice value. If test fails you can simply eliminate the choice from further consideration without making the second test.

In practice, by observation, you can easily identify 18004 as the only choice value which satisfies the first condition. Divide by 7 for confirmation if you want,

$\displaystyle\frac{18004}{7}=2572$.

So, 18000 is the multiple of the four given numbers and when 4 is added, 18004 also becomes divisible by 7. This is the least such number.

Answer: Option b: 18004.

Key concepts used: LCM -- Factors and multiples -- Trial on choice values -- Enumeration method -- Solving in mind.

Q2. LCM of $\displaystyle\frac{2}{3}$, $\displaystyle\frac{4}{9}$ and $\displaystyle\frac{5}{6}$ is,

$\displaystyle\frac{8}{27}$
$\displaystyle\frac{20}{27}$
$\displaystyle\frac{20}{3}$
$\displaystyle\frac{10}{3}$

Solution 2: Problem solving using LCM of fractions method

LCM of fractions $\displaystyle\frac{2}{3}$, $\displaystyle\frac{4}{9}$ and $\displaystyle\frac{5}{6}$ is given by,

$\displaystyle\frac{\text{LCM of numerators}}{\text{HCF of denominators}}$

$=\displaystyle\frac{4\times{5}}{3}=\frac{20}{3}$.

Mechanism of LCM of fractions formula

When you divide the LCM of fractions by any of the fractions, the numerator of the fraction divides it and denominator of the fraction multiplies it.

As the numerator of the target LCM 20 is actually the LCM of the numerators of the fractions 2, 4 and 5, it is a multiple of all four numerators—numerator of each of the fractions will divide the LCM numerator exactly, resulting in an integer.

So LCM of the numerators could have been the LCM of the fractions, as denominator of each fraction multiplies it and the result of division remains an integer.

For example, in case of dividing 20 by first fraction, $\displaystyle\frac{2}{3}$ you get,

$\displaystyle\frac{20}{\displaystyle\frac{2}{3}}=10\times{3}=30$.

For the second fraction division you get,

$\displaystyle\frac{20}{\displaystyle\frac{4}{9}}=5\times{9}=45$.

And for the third fraction division you get,

$\displaystyle\frac{20}{\displaystyle\frac{5}{6}}=4\times{6}=24$.

The necessary condition of being a multiple of the three fractions is satisfied as all the results of divisions are integers.

But is it the least such multiple?

Observe the results of the three divisions of LCM of numerators 20 by each fraction—the result of division by the numerator of the fractions 2, 4 and 5 are further multiplied by the denominator of the fractions, 3, 6 and 9 respectively.

In all the results the factor common to the three denominators 3 is a product in the result thus increasing it. This common factor 3 is in fact the HCF of the denominators.

You must then reduce the result of division, by dividing the LCM of the numerators itself by this HCF of the denominators forming the LCM of the fractions, so that when the fractions divide this LCM of the fractions, the HCF of denominators 3 cancels out thus reducing the result of division to its minimum value.

On dividing the LCM of fractions $\displaystyle\frac{20}{3}$ thus formed, by the fractions, the results of division will now be just,

$10$, $15$ and $8$, still integers with no common factor any more.

Answer: Option c: $\displaystyle\frac{20}{3}$.

Key concepts used: LCM of fractions -- Mechanism of LCM of fractions formula -- Solving in mind.

Solution 3: Problem analysis and solution by of the concept of least number of cans each of highest possible capacity

You'll get the least number of cans to pack 21 litres of cow milk, 42 litres of toned milk and 63 litres of double toned milk, if you find the highest capacity of such a can in each of which only one type of milk is packed.

21 litres, the HCF of 21 litres, 42 litres and 63 litres gives the highest such common capacity. This is the HCF of the three amounts, 21 litres, 42 litres and 63 litres.

You would require then, 1 such can of 21 litres to pack the whole amount of cow milk, 2 such cans to pack whole amount of toned milk and 3 such cans to pack the whole amount of double toned milk.

The minimum total number of cans satisfying the given conditions would then be,

$1+2+3=6$.

The graphic below shows the visualization of the solution,

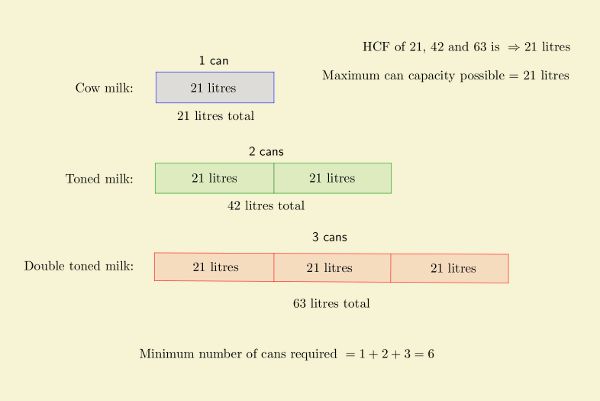
ssc-chsl-solved-questions-hcf-lcm-1-q3

Answer: Option b: 6.

Key concepts used: Highest capacity of cans gives minimum number of cans required -- HCF of amounts of milk gives highest capacity of cans required -- Solving in mind

45 and 76
15 and 228
46 and 75
9 and 380

Solution 4: Problem solving using concept of maximum size of container as HCF gives minimum number of containers

The HCF of 945 and 2475 will give the maximum size of flock possible for both cows and sheep and that will give you the minimum number of equal sized flocks.

To find the HCF of 945 and 2475 we'll first factorize the two numbers,

$945=9\times{5}\times{7}\times{3}$, and

$2475=9\times{25}\times{11}$.

Common are two factors, 9 and 5, product of which is 45. This is the HCF of 945 and 2475 and this flock size will give you the minimum number of maximum sized flocks possible.

At this point without calculating the total number of flocks with this flock size, you can pin-point the answer as option a, as that is the only option with flock size 45.

For clarity though let's find out the number of flocks.

For 945 cows with flock size 45, number of flocks is 21, and for 2475 sheep, with the flock size of 45 number of flocks is 55. Total number of flocks is,

$21+55=76$.

Answer: Option a: 45 and 76.

Key concepts used: Maximum equal container size gives minimum number of containers -- HCF of each content amount gives maximum equal container size.

Solution 5: Problem analysis and solving by concept of maximum size of container as HCF gives minimum number of containers

For each row containing same number fruits of only one kind, the number of fruits in each row must be a factor common to the number of fruits of three types. And the number of such rows to be minimum, the row size must be the largest common factor to the number of fruits of the three types.

So, you have to find the HCF of the three numbers 24, 36 and 60.

Factorize the three numbers to identify the HCF,

$24=12\times{2}$,

$36=12\times{3}$,

$60=12\times{5}$.

The HCF is, 12.

The maximum row size satisfying the given conditions is then 12.

The total number of rows is,

$2+3+5=10$.

Answer: Option d: 10.

Key concepts used: HCF as maximum container size for holding same number of fruits in each row with all rows of same size -- Row size as HCF results in minimum number of rows -- Solving in mind.

Q6. A number between 1000 and 2000 which when divided by 30, 36 and 80 gives a remainder 11 in each case is,

1523
1451
1712
1641

Solution 6: Problem analysis and solution by LCM and multiple in a range concepts

A number which when divided by 30, 36 and 80 results in each case a remainder of 11, must be a multiple of the three numbers plus 11.

The smallest common multiple or LCM of 30, 36 and 80 will be found out from the factored numbers,

$30=2\times{3}\times{5}$,

$36=2\times{2}\times{3}\times{3}$, and

$80=2\times{2}\times{2}\times{2}\times{5}$.

The LCM is,

$3\times{3}\times{5}\times{16}=720$.

The next common multiple of 720 will be in the range between 1000 and 2000. It is,

$2\times{720}=1440$.

Add 11 to 1440 and you have your answer as, 1451.

Answer: Option b : 1451.

Key concepts used: LCM -- Common multiple within a range -- Same remainder for a set of numbers -- Solving in mind.

Q7. The ratio of sum to the LCM of two natural numbers is 7 : 12. If their HCF is 4, then the smaller number is,

Solution 7: Problem analysis and Solving by Enumeration, Basic concepts of HCF and LCM and Ratio concept

Let the two numbers be $x$ and $y$.

The ratio can then be expressed as,

$\displaystyle\frac{x+y}{\text{LCM of }x\text{ and }y}=\frac{7}{12}$.

From concepts of HCF and LCM, we know,

$xy=\text{HCF}\times{\text{LCM}}$,

Or, $xy=4\times{\text{LCM}}$.

Multiplying denominators of both sides in the first equation by 4 and replacing the LHS denominator by $xy$ we get,

$\displaystyle\frac{x+y}{xy}=\displaystyle\frac{1}{x}+\displaystyle\frac{1}{y}=\displaystyle\frac{7}{48}$.

This means sum of inverses of $x$ and $y$ is $\displaystyle\frac{7}{48}$.

Now we'll resort to enumeration of possible values forming numerator 7.

First case: $7=6+1$,

$x=\displaystyle\frac{48}{6}=8$ and $y=48$.

HCF in this case is 8 and it violates given value of HCF.

Second case: $7=5+2$ is invalid as it will result in fractional $x=\displaystyle\frac{48}{5}$.

Third case: $7=4+3$.

$x=\displaystyle\frac{48}{4}=12$, and $y=\displaystyle\frac{48}{3}=16$.

HCF of the two is 4 and LCM is 48.

Ratio of sum and LCM is,

$\displaystyle\frac{28}{48}=\frac{7}{12}$.

All conditions satisfied.

The smaller number is then, 12.

Let's show you the faster conceptual solution.

Both the sum of the two numbers and LCM must have the HCF included in them which after cancelling out resulted in the fractional ratio $\displaystyle\frac{7}{12}$.

Reintroduce then this cancelled out HCF by multiplying with 4 to get the actual values of the sum and LCM as respectively,

$x+y=7\times{4}=28$, and

$\text{LCM}=12\times{4}=48$.

Mentally trying out combinations of values, $x=12$ and $y=16$ can be arrived at quickly that satisfy all conditions.

Answer: Option a: 12.

Key concepts used: HCF and LCM concepts -- Enumeration -- HCF reintroduction technique -- Ratio concept -- Solving in mind.

Q8. The smallest 5 digit number which is divisible by 12, 18, and 21 is,

10224
30526
50321
10080

Solution 8: Problem analysis and Solving by LCM and multiple in a range concepts

The smallest number that is divisible by 12, 18 and 21 is the LCM of the three numbers.

Let us find the LCM by factorizing the three numbers as,

$12=2\times{2}\times{3}$.

$18=2\times{3}\times{3}$, and

$21=3\times{7}$.

Product of 2 numbers of 2s, 2 numbers of 3s and 1 number of 7 will be the LCM. This is,

$4\times{9}\times{7}=252$.

Multiply 252 by 40 and just get into 5 digit zone,

$252\times{40}=10080$.

This must be the smallest 5 digit number divisible by all three given numbers. This is because if you subtract one number of 252 from 10080, the result 9828 becomes a 4 digit number.

Answer: Option d: 10080.

Key concepts used: LCM concepts -- Smallest multiple within a range -- Solving in mind.

Solution 9: Problem analysis and Solving by fraction change, linear equation solution and LCM concepts

Let the $x$ and $y$ be the numerator and denominator of the fraction.

By the first statement the new fraction formed is,

$\displaystyle\frac{x-4}{y+1}=\frac{1}{6}$.

Simplify to get the first linear equation as,

$6x-24=y+1$,

Or, $6x-y=25$.

Similarly in the second case,

$\displaystyle\frac{x+2}{y+1}=\frac{1}{3}$,

Or, $3x+6=y+1$,

Or, $3x-y=-5$.

Subtract this second equation from the first. Result is,

$3x=30$,

Or, $x=10=5\times{2}$.

Substitute in second equation,

$30-y=-5$,

Or, $y=35=5\times{7}$.

The LCM of the numerator and denominator is,

$2\times{5}\times{7}=70$.

Answer: Option a: 70.

Key concepts used: Fraction change problem -- Solution of linear equations by elimination -- LCM.

The fraction in normalized form by eliminating common factor between numerator and denominator is,

$\displaystyle\frac{10}{35}=\frac{2}{7}$.

But in that case, when 4 is subtracted from numerator and 1 is added to denominator, result becomes,

$\displaystyle\frac{2-4}{7+1}=-\frac{1}{4}$ violating the first given condition.

This is why you must keep the fraction in the form of $\displaystyle\frac{10}{35}$ that satisfies both the linear equations and hence both the given conditions.

Q10. If $A$ and $B$ are the HCF and LCM respectively of two algebraic expressions $x$ and $y$, and $A+B=x+y$, then value of $A^3+B^3$ is,

$x^3-y^3$
$x^3+y^3$
$y^3$
$x^3$

Solution 10: Problem analysis and Solution using relation between two expressions and their HCF and LCM, and two factor expanded form of sum of cubes

The relation between two expressions $x$ and $y$ and their HCF, LCM is,

$\text{HCF}\times{LCM}=xy$,

Or, $AB=xy$.

Now express $A^3+B^3$ in expanded two factor product form of,

$A^3+B^3=(A+B)(A^2-AB+B^2)$

$=(A+B)\left[(A+B)^2-3AB\right]$

$=(x+y)\left[(x+y)^2-3xy\right]$

$=x^3+y^3$.

Answer: Option b: $x^3+y^3$.

Key concepts used: Relation between two expressions and their HCF and LCM—product of two expressions equals product of their HCF and LCM -- Two factor expanded form of $A^3+B^3$.

Other solved set of LCM HCF questions for SSC CHSL follows,

LCM HCF questions for SSC CHSL with Answers and Quick Solutions 2.

These two sets cover most of the difficult LCM HCF questions for SSC CHSL.

Guided help on Number system, HCF LCM in Suresolv

To get the best results out of the extensive range of articles of tutorials, questions and solutions on Number system and HCF LCM in Suresolv, follow the guide,

Suresolv Number system, HCF LCM Reading and Practice Guide for SSC CHSL, SSC CGL, SSC CGL Tier II, Bank PO and Other Competitive exams.

The guide list of articles is up-to-date.

SSC CHSL level Question and Solution sets

Work and Time, Pipes and Cisterns

SSC CHSL level Solved Question set 1 on Work time 1

SSC CHSL level Solved Question set 2 on Work time 2

Number System, HCF and LCM

SSC CHSL level Solved Question set 3 on Number system 1

SSC CHSL level Solved Question set 4 on Number system 2

SSC CHSL level Solved Question set 5 on HCF and LCM 1

SSC CHSL level Solved Question set 6 on HCF and LCM 2

You are here

SSC CHSL Solved question Set 5, HCF LCM 1